A piece of wire is bent in the shape of a parabola y=kx2 (y-axis vertical) with a bead of mass m on it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated parallel to the x-axis with a constant acceleration a. The distance of the new equilibrium position of the bead, where the bead can stay at rest with respect to the wire, from the y-axis is:
a/gk
a/2gk
2a/gk
a/4gk
The correct Answer is:B
To solve the problem, we need to find the new equilibrium position of the bead on the parabolic wire when the wire is accelerated with a constant acceleration a parallel to the x-axis.
1. Understanding the Parabola:
The equation of the parabola is given as y=kx2. This means that the parabola opens upwards with its vertex at the origin (0,0).
2. Forces Acting on the Bead:
When the wire is accelerated, the bead experiences a normal force N from the wire and its weight mg acting downwards. The normal force can be resolved into two components:
- Horizontal component: Ncosθ
- Vertical component: Nsinθ
3. Equations of Motion:
In the horizontal direction, the bead must satisfy:
Ncosθ=ma
In the vertical direction, the bead must satisfy:
Nsinθ=mg
4. Dividing the Equations:
Dividing the horizontal equation by the vertical equation gives:
NcosθNsinθ=mamg
This simplifies to:
cotθ=ag
5. Finding the Slope of the Parabola:
The slope of the parabola at any point is given by the derivative dydx. For the parabola y=kx2:
dydx=2kx
This slope at the bead's position can also be expressed in terms of the angle α as:
tanα=2kx
6. Relating Angles:
From geometry, we know that:
α+θ=π2
Therefore, we can express tanα in terms of cotθ:
tanα=cotθ=ga
7. Setting Up the Equation:
From the previous steps, we have:
2kx=ga
8. Solving for x:
Rearranging gives:
x=g2ka
Final Answer:
The distance of the new equilibrium position of the bead from the y-axis is:
x=a2kg
The equation of the parabola is given as y=kx2. This means that the parabola opens upwards with its vertex at the origin (0,0).
When the wire is accelerated, the bead experiences a normal force N from the wire and its weight mg acting downwards. The normal force can be resolved into two components:
- Horizontal component: Ncosθ
- Vertical component: Nsinθ
In the horizontal direction, the bead must satisfy:
Ncosθ=ma
In the vertical direction, the bead must satisfy:
Nsinθ=mg
Dividing the horizontal equation by the vertical equation gives:
NcosθNsinθ=mamg
This simplifies to:
cotθ=ag
The slope of the parabola at any point is given by the derivative dydx. For the parabola y=kx2:
dydx=2kx
This slope at the bead's position can also be expressed in terms of the angle α as:
tanα=2kx
From geometry, we know that:
α+θ=π2
Therefore, we can express tanα in terms of cotθ:
tanα=cotθ=ga
From the previous steps, we have:
2kx=ga
Rearranging gives:
x=g2ka
The distance of the new equilibrium position of the bead from the y-axis is:
x=a2kg
Similar Questions
A piece of wire is bent in the shape of a parabola y=kx2 (y axis vertical) with a bead of mass mon it. The bead can slide on the wire without friction. It stays at the lowest point of the parabola when the wire is at rest. The wire is now accelerated paralle to the x-axis with a constant acceleration a. The distance of the the new equilibrium position of the bead. Find the x-coordinate where the bead can stay at rest with respect to the wire ?
View SolutionA straight wire carrying current is parallel to the y-axis as shown in Fig. The
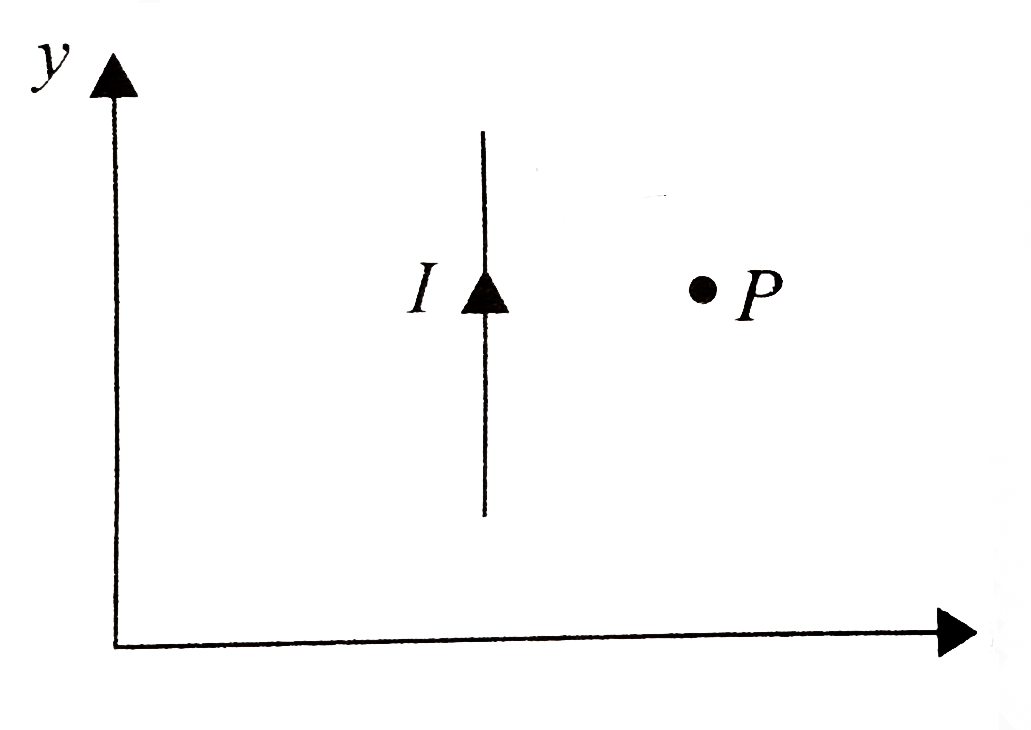
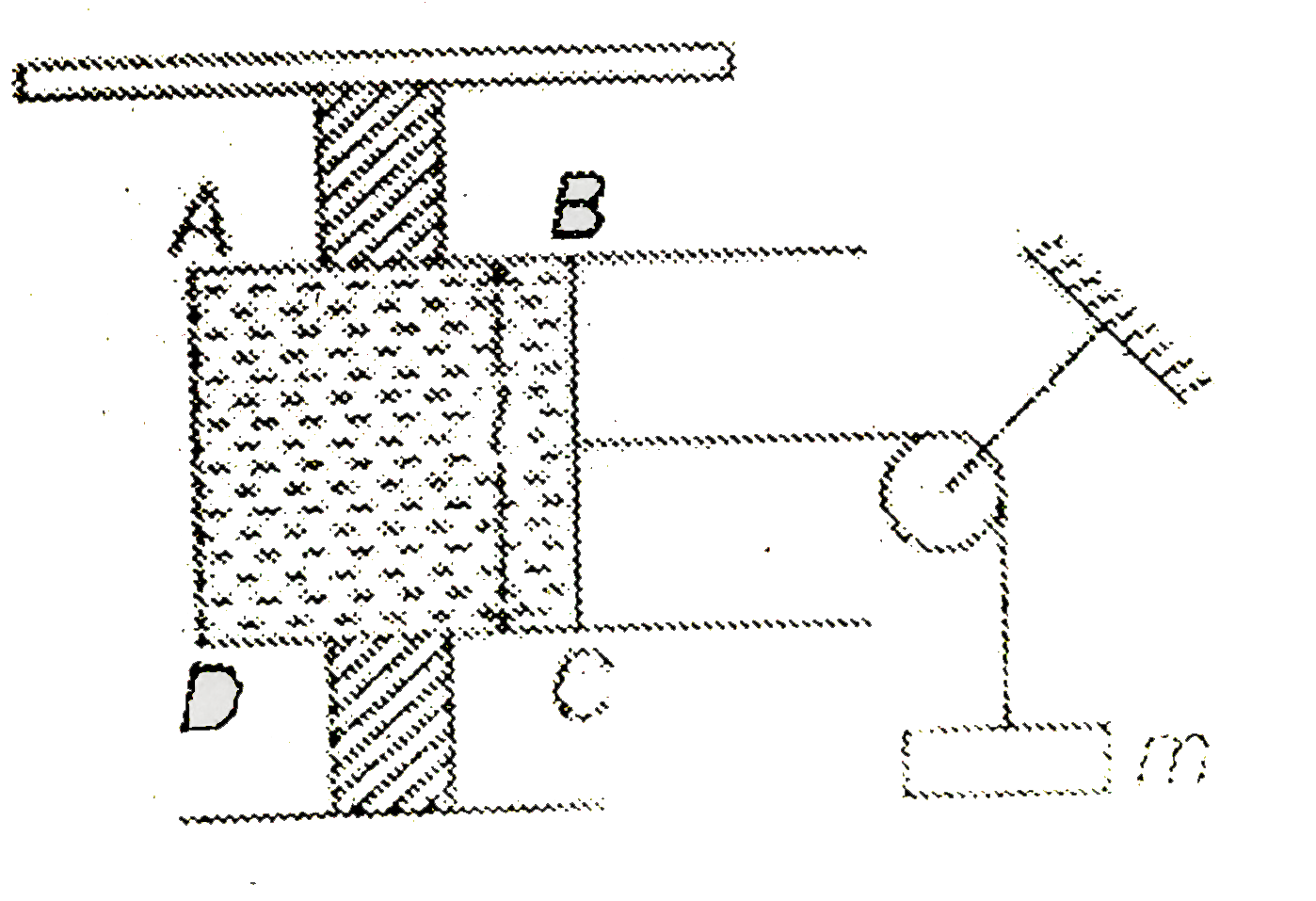
View SolutionA wire frame ABCD has a soap film. The wire BC can slide on the frame without friction and it is in equilibrium in the position shown in the figure. Find m, if T is the surface tension of the liquid.
View SolutionA bead of mass m is fitted on a rod and can move on it without friction. Initially the bead is at the middle of the rod moves transitionally in the vertical plane with an accleration a0 in direction forming angle α with the rod as shown. The acceleration of bead with respect to rod is:
z
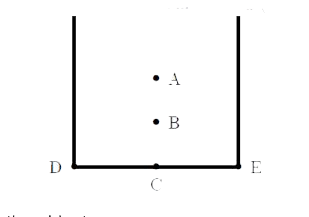
View SolutionA thick uniform wire is bent into the shape of the letter “U” as shown. Which point indicates the location of the center of mass of this wire?
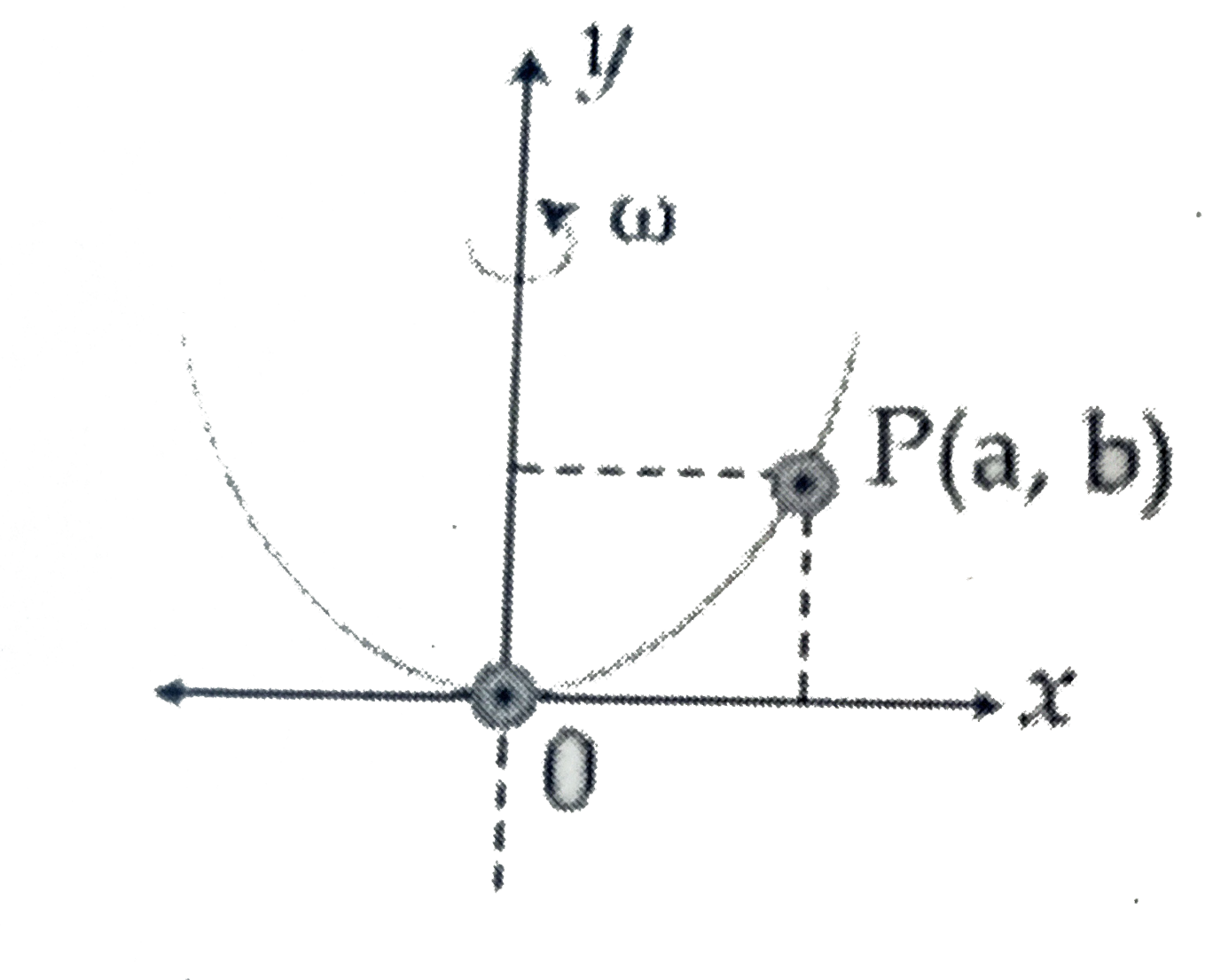
View SolutionA bead of mass m stays at point P (a,b) on a wire bent in the shape of a parabola y=4Cx2 and rotating with angular speed ω (see figure ) . The value of ω is (neglect friction),
View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is

View SolutionA bead of mass m is located on a parabolic wire with its axis vertical and vertex at the origin as shown in figure and whose equastion is x2=4ay. The wire frame is fixed and the bead is released from the point y=4a on the wire frame from rest. The tangential acceleration of the bead when it reaches the position given by y=a is

View SolutionAngular velocity of smooth parabolic wire y = 4c(x)^(2) about axis of parabola in vertical plane if bead of mass m does not slip at (a,b) will be
View SolutionA smooth wire of length 2πr is bent into a circle and kept in a vertical plane. A bead can slide smoothly on the wire. When the circle is rotating with angular speed about the vertical diameter AB, as shown in the figure, the bead is at rest with respect to the circular ring at potion P as shown. Then the value of ω2 is equal to:
View Solution
