The velocity of a particle is zero at time t = 2s, then
displacement must be zero in the interval t = 0 to t = 2 s.
acceleration may be zero at t = 2 s.
velocity must be zero for t>2s .
acceleration must be zero at t = 2 s.
The correct Answer is:B
To solve the problem, we need to analyze the statements given in the context of the velocity of a particle being zero at a specific time, t = 2 seconds.
1. Understanding the Problem:
The velocity of a particle is zero at time t = 2 seconds. We need to evaluate the truth of three statements regarding the particle's motion.
2. Analyzing Statement A:
- Statement A claims that the displacement must be 0 in the interval from t = 0 to t = 2 seconds.
- This statement is false.
- Explanation: A particle can have a non-zero displacement even if its velocity is zero at a certain point in time. For example, it could start from point A, move to point B, and come to rest at point B at t = 2 seconds. Thus, the displacement is not necessarily zero.
3. Analyzing Statement B:
- Statement B states that the acceleration may be zero at time t = 2 seconds.
- This statement is true.
- Explanation: At t = 2 seconds, the particle could be at rest (velocity = 0) and could either have zero acceleration (i.e., it remains at rest) or have some acceleration after that point. Therefore, it is possible for the acceleration to be zero at that instant.
4. Analyzing Statement C:
- Statement C claims that the velocity must be zero for t greater than 2 seconds.
- This statement is false.
- Explanation: The particle can have a velocity greater than zero after t = 2 seconds. For example, it could remain at rest for a while and then start moving, or it could start moving immediately after t = 2 seconds. Thus, the velocity does not have to remain zero.
5. Conclusion:
Based on the analysis of the three statements, the only true statement is Statement B.
Final Answer:
The correct answer is Statement B: The acceleration may be zero at time t = 2 seconds.
---
The velocity of a particle is zero at time t = 2 seconds. We need to evaluate the truth of three statements regarding the particle's motion.
- Statement A claims that the displacement must be 0 in the interval from t = 0 to t = 2 seconds.
- This statement is false.
- Explanation: A particle can have a non-zero displacement even if its velocity is zero at a certain point in time. For example, it could start from point A, move to point B, and come to rest at point B at t = 2 seconds. Thus, the displacement is not necessarily zero.
- Statement B states that the acceleration may be zero at time t = 2 seconds.
- This statement is true.
- Explanation: At t = 2 seconds, the particle could be at rest (velocity = 0) and could either have zero acceleration (i.e., it remains at rest) or have some acceleration after that point. Therefore, it is possible for the acceleration to be zero at that instant.
- Statement C claims that the velocity must be zero for t greater than 2 seconds.
- This statement is false.
- Explanation: The particle can have a velocity greater than zero after t = 2 seconds. For example, it could remain at rest for a while and then start moving, or it could start moving immediately after t = 2 seconds. Thus, the velocity does not have to remain zero.
Based on the analysis of the three statements, the only true statement is Statement B.
The correct answer is Statement B: The acceleration may be zero at time t = 2 seconds.
Similar Questions
Knowledge Check
The velocity of the particle at any time t is given by vu=2t(3−t)ms−1. At what time is its velocity maximum?
A2 sB3 sC2/3 sD3/2 s
The acceleration-time graph of a particle moving in a straight line is as shown in figure. The velocity of the particle at time t=0 is 2m/s. The velocity after 2 seconds will be
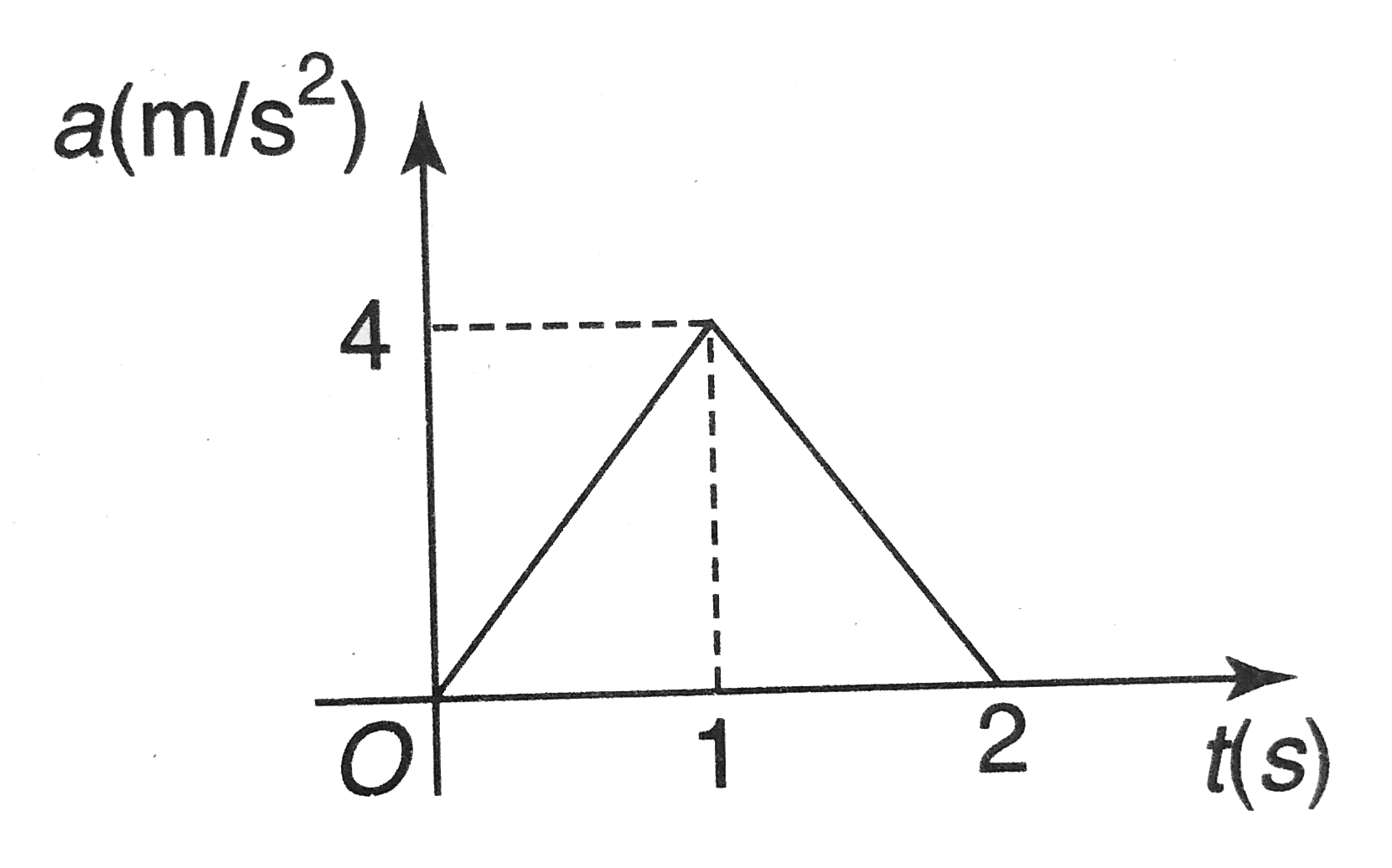
View SolutionThe acceleration-time graph of a particle moving in a straight line is as shown in figure. The velocity of the particle at time t=0 is 2m/s. The velocity after 2 seconds will be

View SolutionThe velocity time relation of a particle is given by v =(3t2−2t−1)m/s Calculate the position and acceleration of the particle when velocity of the particle is zero . Given the initial position of the particle is 5m .
View SolutionPower supplied to a mass 2kg varies with time as P=3t22 watt. Here t is in second . If velocity of particle at t=0isv=0, the velocity of particle at time t=2s will be:
View SolutionPower supplied to a particle of mass 3 kg varies with time as p=4t3 watt, where t in time is seconds. If velocity of particle at t=0s is u=2m/s. The velocity of particle at time t=3s will be :
View SolutionPower supplied to a particle of mass 3 kg varies with time as p=4t3 watt, where t in time is seconds. If velocity of particle at t=0s is u=2m/s. The velocity of particle at time t=3s will be :
View SolutionThe acceleratin of a particle increases linearly with time t as 6t. If the initial velocity of the particles is zero and the particle starts from the origin, then the distance traveled by the particle in time t will be
View Solution