A flywheel of moment of inertia 0.4 kg m2 and radius 0.2 m is free to rotate about a central axis. If a string is wrapped around it and it is pulled with a force of 10N. Then its angular velocity after 4s will be
10rads−1
5rads−1
20rads−1
None of these
The correct Answer is:C
To solve the problem step by step, we will use the concepts of torque, moment of inertia, and angular acceleration.
Step 1: Identify the given values
- Moment of inertia (I) = 0.4 kg·m²
- Radius (r) = 0.2 m
- Force (F) = 10 N
- Time (t) = 4 s
Step 2: Calculate the torque (τ) applied to the flywheel
The torque exerted by the force on the flywheel can be calculated using the formula:
τ=F⋅r
Substituting the values:
τ=10N⋅0.2m=2N⋅m
Step 3: Relate torque to angular acceleration (α)
The torque is also related to the moment of inertia and angular acceleration by the formula:
τ=I⋅α
We can rearrange this to find angular acceleration (α):
α=τI
Substituting the values:
α=2N⋅m0.4kg⋅m²=5rad/s²
Step 4: Calculate the final angular velocity (ω) after 4 seconds
Since the flywheel starts from rest, the initial angular velocity (ω₁) is 0. We can use the formula for angular velocity:
ω2=ω1+α⋅t
Substituting the values:
ω2=0+(5rad/s²)⋅(4s)=20rad/s
Final Answer
The angular velocity after 4 seconds is:
ω2=20rad/s
---
- Moment of inertia (I) = 0.4 kg·m²
- Radius (r) = 0.2 m
- Force (F) = 10 N
- Time (t) = 4 s
The torque exerted by the force on the flywheel can be calculated using the formula:
τ=F⋅r
Substituting the values:
τ=10N⋅0.2m=2N⋅m
The torque is also related to the moment of inertia and angular acceleration by the formula:
τ=I⋅α
We can rearrange this to find angular acceleration (α):
α=τI
Substituting the values:
α=2N⋅m0.4kg⋅m²=5rad/s²
Since the flywheel starts from rest, the initial angular velocity (ω₁) is 0. We can use the formula for angular velocity:
ω2=ω1+α⋅t
Substituting the values:
ω2=0+(5rad/s²)⋅(4s)=20rad/s
The angular velocity after 4 seconds is:
ω2=20rad/s
Similar Questions
A string is wrapped around the rim of a wheel of moment of inertia 0.20 kg-m^2 and radius 20 cm. The wheel is free to rotate about it axis. Initially, the wheel is at rest. The string is now pulled by a force of 20 N. Find the angular velocity of the wheel after 5.0 seconds.
View SolutionA wheel of moment of inertia I and radius r is free to rotate about its centre as shown in figure. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The system is released from rest. Find the speed of the block as it descends through a height h.

View SolutionA fly wheel of 0.4kg(m−2) M.Iand of 0.2 m radius can rotate about its axis.A rope is wrapped around its circumference and pulled with 10 N force. The value of angular acceleration in radsec2will be?
View SolutionA wheel of radius 20 cm and moment of inertia 1 kgm^2 can rotate about its centre.A string is wrapped over its rim and is pulled by a force 'F' tangential to the rim of wheel.If angular acceleration produced is 2 rad/s^2 then the value of force 'F' is
View SolutionA wheel of moment of inertia 0.500kg−m2 and radius 20.0 cm is rotating about its axis at an angular speed of 20.0 rad/s. It picks up a stationary particle of mass 200 g at its edge. Find the new angular speed of the wheel.
View SolutionA solid cylinder of mass 50kg and radius 0.5m is free to rotate about the horizontal axis. A massless string is wound round the cylinder with one end attached to it and other end hanging freely. Tension in the string required to produce an angular acceleration of 2 revolution s−2 is
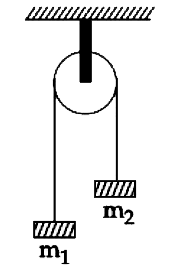
View SolutionA uniformly thick wheel with moment of inertia I and radius R is free to rotate about its centre of mass (see fig). A massless string is wrapped over its rim and two blocks of masses m1andm2(m1>m2) are attached to the ends of the string. The system is released from rest. The angular speed of the wheel when m1 descents by a distance h is :
View SolutionThe top is figure has moment of inertia equal to 4.00×104kgm2 and is initially at rest. It is free to rotate about the stationary axis AA'. A string wrapped around a ped alonng the axis of the top is pulled in such a manners as to maintain a constant tension of 5.57N.If the string does not slip while it is unwound from the peg, what is the angular speed of the top after 80.0CM of string has been pulled of the peg?

View Solutiona body of mass 10 kg and radius of gyration 0.1 m is rotating about an axis. If angular speed is 10 rad/s, then angular momentum will be:
View SolutionA hollow sphere of mass 1 kg and radius 10 cm is free to rotate about its diameter. If a force of 30 N is applied tangentially to it, its angular acceleration is (in rad/s2)
View Solution