A ball reaches a racket at 60m/s along +X direction and leaves the racket in the opposite direction with the same speed. Assuming that the mass of the ball as 50gm and the contact time is 0.02 second the force exerted by the racket on the ball is .
300 N along + X direction
300 N along -X direction
300 kN along +X direction
300 kN along - X direction
The correct Answer is:B
To solve the problem, we need to calculate the force exerted by the racket on the ball using the concepts of momentum and Newton's second law of motion. Here’s a step-by-step solution:
Step 1: Identify the given values
- Initial velocity of the ball, u=60m/s (in the +X direction)
- Final velocity of the ball, v=−60m/s (in the -X direction)
- Mass of the ball, m=50g=0.05kg (convert grams to kilograms)
- Time of contact, t=0.02s
Step 2: Calculate the change in velocity
The change in velocity (Δv) can be calculated as:
Δv=v−u=−60m/s−60m/s=−120m/s
Step 3: Calculate the acceleration
Acceleration (a) is defined as the change in velocity over time:
a=Δvt=−120m/s0.02s=−6000m/s2
Step 4: Calculate the net force using Newton's second law
According to Newton's second law, the net force (F) is given by:
F=m⋅a
Substituting the values:
F=0.05kg⋅(−6000m/s2)=−300N
Step 5: Interpret the result
The negative sign indicates that the force is in the opposite direction to the positive X direction, which is consistent with the ball leaving the racket in the opposite direction.
Final Answer
The force exerted by the racket on the ball is 300N in the negative X direction.
---
- Initial velocity of the ball, u=60m/s (in the +X direction)
- Final velocity of the ball, v=−60m/s (in the -X direction)
- Mass of the ball, m=50g=0.05kg (convert grams to kilograms)
- Time of contact, t=0.02s
The change in velocity (Δv) can be calculated as:
Δv=v−u=−60m/s−60m/s=−120m/s
Acceleration (a) is defined as the change in velocity over time:
a=Δvt=−120m/s0.02s=−6000m/s2
According to Newton's second law, the net force (F) is given by:
F=m⋅a
Substituting the values:
F=0.05kg⋅(−6000m/s2)=−300N
The negative sign indicates that the force is in the opposite direction to the positive X direction, which is consistent with the ball leaving the racket in the opposite direction.
The force exerted by the racket on the ball is 300N in the negative X direction.
Similar Questions
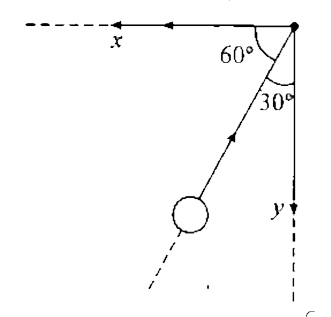
A hockey player receives a corner shot at a speed of 15m/s at angle 30∘ with y-axis and then shoots the ball along x-axis with the speed 30m/s. If the mass of the ball is 150g and it remains in contact with the hockey stick for 0.01s, the force exerted on the ball along x-axis is

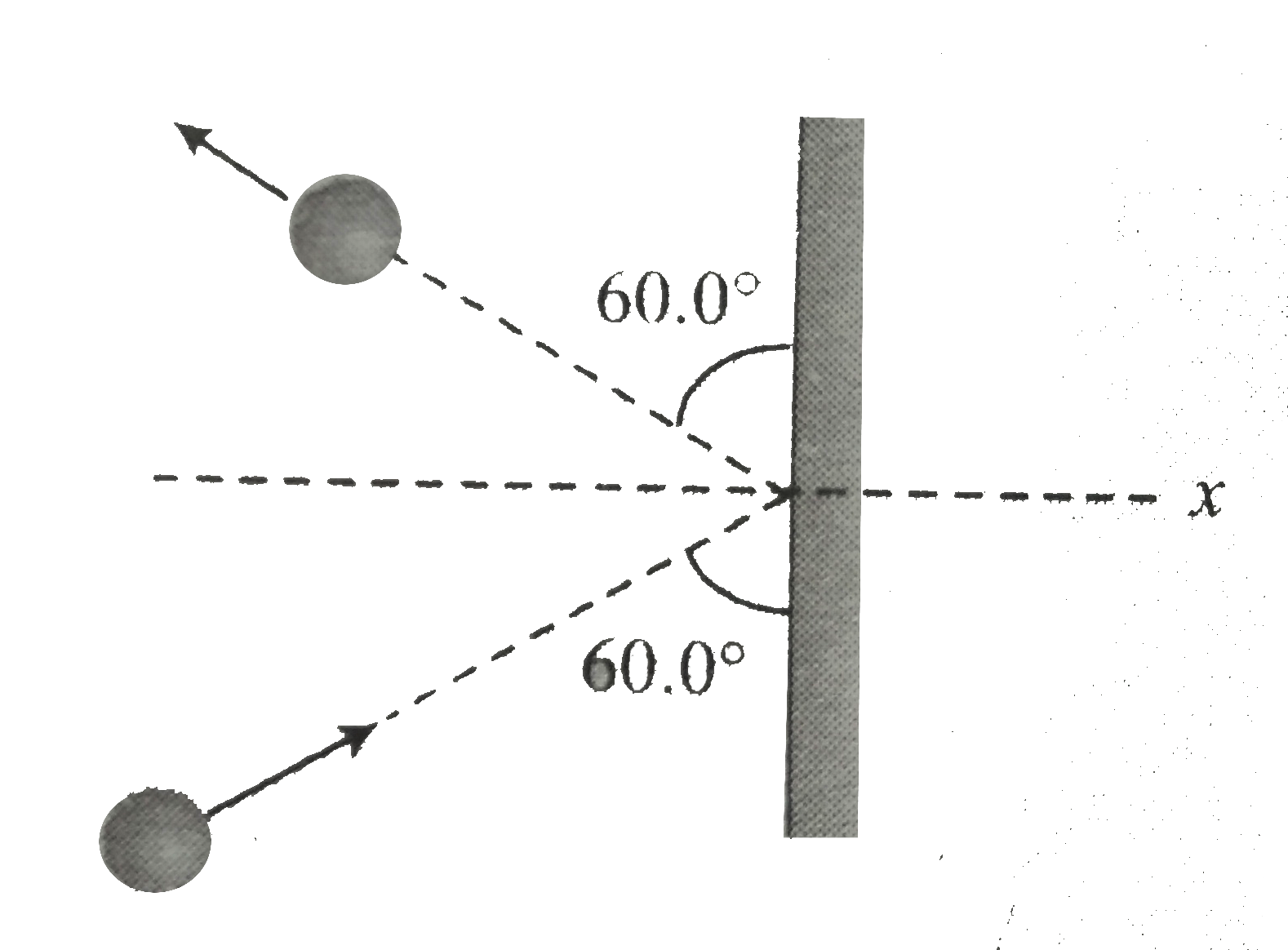
View SolutionA 3-kg steel ball strikes a wall with a speed of 10.0ms−1 at an angle of 60.0∘ with the surfaces of the wall. The ball bounces off with the same speed and same angle. If the ball was in contact with the wall for 0.2s, find the average force exerted by the wall on the ball.
View SolutionA ball weighing 10g hits a hard surface vertically with a speed of 5m/s and rebounds with the same speed The ball remains in contact with the surface speed The ball remains in contact with the surface for 0.01s The average force exerted by the surface on the ball is .
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA ball of mass 0.5kg moving with a velocity of 2m/s strikes a wall normally and bounces back with the same speed . If the time of contact between the ball and the wall is 1 millisecond , the average force exerted by the wall on the ball is
View SolutionA 3.00kg steel hall strikes the wall with a speed of 10.0m/s at an angle of 60∘ with the surface. It bounces off with the same speed and angle. If the ball is in contact with the wall for 0.200s, what is the average force exerted by the wall on the ball?
View SolutionA hockey player receives a corner shot at a speed of 15m/s at an angle of 30∘ with the y-axis and then shoots the ball along the x-axis with a speed of 30m/s If the mass of the ball is 150gm and it remains in contact with the hockey stick for 0.01s, the force imparted to the ball in the x-direction is :
View SolutionA bowler tbrows a ball horizontally along east direction with speed of 144km/hr. The batsman hits the ball such that it deviates from its initial direction of motion by 74∘ north of east direction, without changing its speed. If mass of the ball is 13kg and time of contact between bat and ball is 0.02 s. Average force applied by batsman on ball is:
A. 800 N, 53∘East of North
B. 800N, 53∘ North of East
C. 800 N, 53∘ North of West
D. 800N, 53∘ West of North
View SolutionThe following problems illustrate the effect of a time-dependent force of a large average magnitude which acts on a moving object only for a short duration. Such forces are called 'impulsive' forces. According to the impulse-momentum theorem, impulse delivered to a body is equal to the change of linear momentum of the body.
A ball of mass 250g is thrown with a speed of 30m/s. The ball strikes a bat and it is hit straight back along the same line at a speed of 50m/s. Variation of the interaction force, as long as the ball remains in contact with the bat, is as shown in Fig.
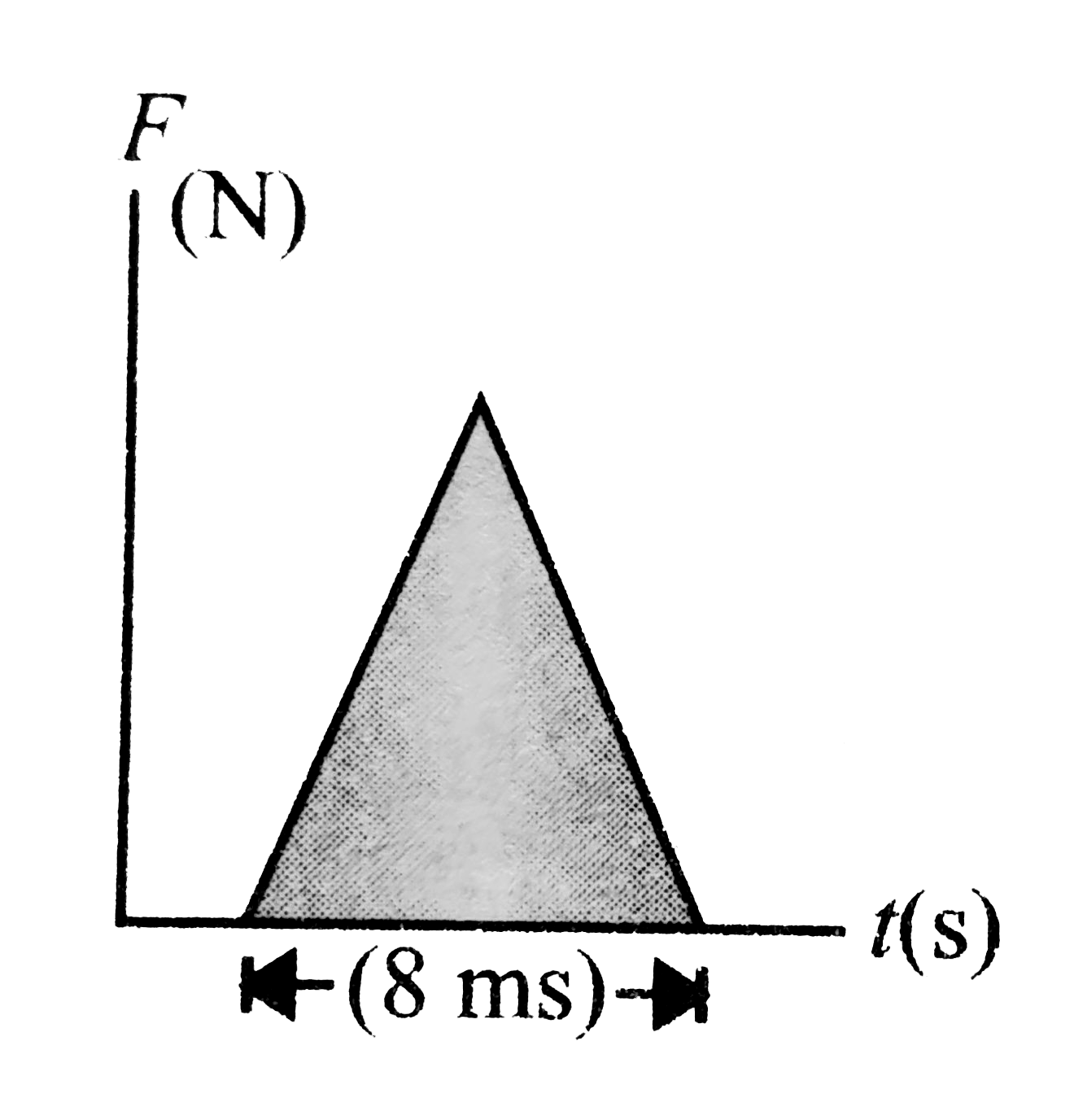
Maximum force exerted by the bat on the ball is
View SolutionA 150 g ball, moving horizontally at 20 m/s was hit straight back to bowler at 35 m/s. If contact with bat lasted for 0.04sec, than average force exerted by the bat on the ball is:

View Solution
