If ∣∣ ∣ ∣∣a+1a+2a+pa+2a+3a+qa+3a+4a+r∣∣ ∣ ∣∣=0,thenp,q,r are in:
To solve the problem, we need to analyze the determinant of the given matrix and derive the relationships between p,q, and r.
Step 1: Set up the determinant
We have the determinant of the matrix:
∣∣
∣∣a+1a+2a+pa+2a+3a+qa+3a+4a+r∣∣
∣∣=0
Step 2: Perform row operations
We can simplify the determinant by performing row operations. Let's subtract the first row from the second and third rows:
R2→R2−R1R3→R3−R1
This gives us:
∣∣
∣∣a+1a+2a+p11q−p22r−p∣∣
∣∣=0
Step 3: Further simplify the determinant
Now, we can simplify the second column by subtracting the first column from the second:
C2→C2−C1
This results in:
∣∣
∣∣a+11a+p10q−p20r−p∣∣
∣∣=0
Step 4: Expand the determinant
Now we can expand the determinant along the second column:
=1⋅∣∣∣1q−p2r−p∣∣∣
Calculating the 2x2 determinant:
=1⋅(1⋅(r−p)−2⋅(q−p))=(r−p)−2(q−p)
This simplifies to:
r−p−2q+2p=0
or
r+p−2q=0
Step 5: Rearranging the equation
Rearranging gives us:
2q=r+p⇒q=r+p2
Step 6: Identify the relationship
The equation q=r+p2 indicates that p,q,r are in Arithmetic Progression (AP) since q is the average of p and r.
Conclusion
Thus, we conclude that p,q,r are in Arithmetic Progression (AP).
---
We have the determinant of the matrix:
∣∣
∣∣a+1a+2a+pa+2a+3a+qa+3a+4a+r∣∣
∣∣=0
We can simplify the determinant by performing row operations. Let's subtract the first row from the second and third rows:
R2→R2−R1R3→R3−R1
This gives us:
∣∣
∣∣a+1a+2a+p11q−p22r−p∣∣
∣∣=0
Now, we can simplify the second column by subtracting the first column from the second:
C2→C2−C1
This results in:
∣∣
∣∣a+11a+p10q−p20r−p∣∣
∣∣=0
Now we can expand the determinant along the second column:
=1⋅∣∣∣1q−p2r−p∣∣∣
Calculating the 2x2 determinant:
=1⋅(1⋅(r−p)−2⋅(q−p))=(r−p)−2(q−p)
This simplifies to:
r−p−2q+2p=0
or
r+p−2q=0
Rearranging gives us:
2q=r+p⇒q=r+p2
The equation q=r+p2 indicates that p,q,r are in Arithmetic Progression (AP) since q is the average of p and r.
Thus, we conclude that p,q,r are in Arithmetic Progression (AP).
Topper's Solved these Questions
Similar Questions
∣∣ ∣ ∣∣a+1a+2a+pa+2a+3a+qa+3a+4a+r∣∣ ∣ ∣∣=0 , then p, q r are in
(A) AP
(B) GP
(C) HP
(D) none of these
View SolutionThe vertices of a triangle are (pq,1pq),(pq)),(qr,1qr), and (rq,1rp), where p,q and r are the roots of the equation y3−3y2+6y+1=0 . The coordinates of its centroid are (1,2) (b) (2,−1) (c) (1,−1) (d) (2,3)
View SolutionLet A={p,q,r}. Which of the following is an equivalence relation on A? (a) R1={(p,q),(q,r),(p,r),(p,q)} (b) R2={(r,q),(r,p),(r.r),(q,r)} (c) R3={(p,p),(q,q),(r,r),(p,q)} (d) one of these
View SolutionIf a1,a2,a3,…. are in A.P., then ap,aq,ar are in A.P. if p,q,r are in
View SolutionIf the roots of the equation px2+qx+r=0, where 2p,q,2r are in G.P, are of the form α2,4α−4. Then the value of 2p+4q+7r is :
View SolutionShow that ∣∣ ∣ ∣∣11+p1+p+q23+2p4+3p+2q36+3p10+6p+3q∣∣ ∣ ∣∣=1.
View SolutionFind the value of :
2p + 3q + 4r + por when p =-1,q = 2 and r = 3
View SolutionIf p(q−r)x2+q(r−p)x+r(p−q)=0 has equal roots, then show that p, q, r are in H.P.
View SolutionMatch the following lists (where [x] represents the greatest integer function) and then choose the correct code.
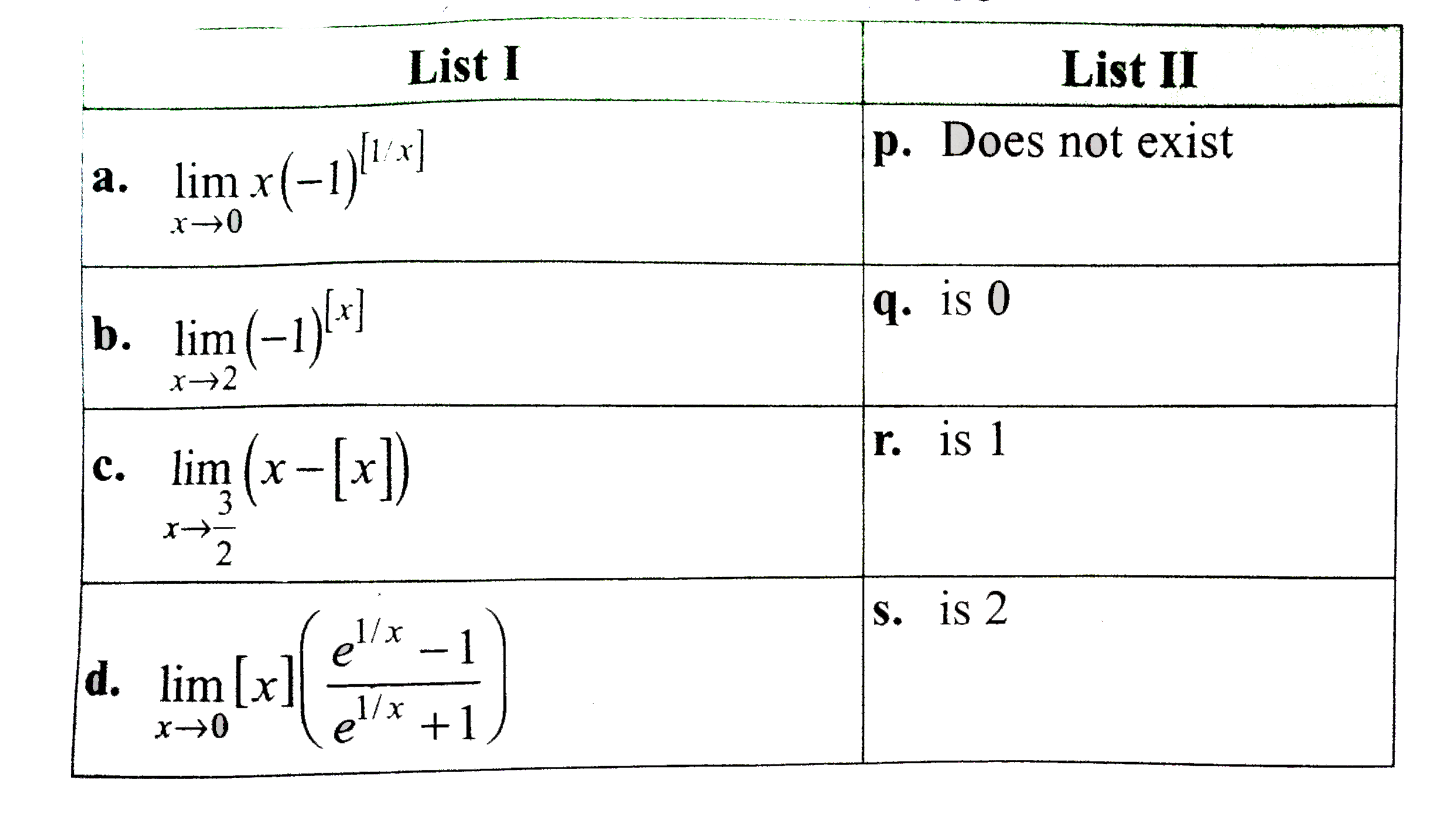
Codes :
a b c d(1)s r q p(2)q p s p(3)s r p q(4)p p q r
View SolutionShow that the points (−2,3,5), (1,2,3)and (7,0,−1)are collinear.
View Solution
