If G be the centroid of a triangle ABC, prove that,Â AB2+BC2+CA2=3(GA2+GB2+GC2)

Video Solution

|

Answer

Step by step video & image solution for If G be the centroid of a triangle ABC, prove that,Â AB2+BC2+CA2=3(GA2+GB2+GC2) by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS STRAIGHT LINES

Similar Questions

If G be the centroid of a triangle ABC, prove that, $A B^{2} + B C^{2} + C A^{2} = 3 (G A^{2} + G B^{2} + G C^{2})$
View Solution
AD is a median of triangle ABC. Prove that : $A B + B C + A C > 2 A D$
View Solution
AD is a median of triangle ABC. Prove that : $A B + A C > 2 A D$
View Solution
In a tetrahedron OABC, the edges are of lengths, $| O A | = | B C | = a, | O B | = | A C | = b, | O C | = | A B | = c .$ Let $G_{1} and G_{2}$ be the centroids of the triangle ABC and AOC such that $O G_{1} ⊥ B G_{2},$ then the value of $\frac{a^{2} + c^{2}}{b^{2}}$ is
View Solution
lf G be the centroid of a triangle ABC and P be any other point in the plane prove that $P A^{2} + P B^{2} + P C^{2} = G A^{2} + G B^{2} + G C^{2} + 3 G P^{2}$
View Solution
If G is the centroid of a $Δ A B C$ , then $G A^{2} + G B^{2} + G C^{2}$ is equal to
View Solution
In the following figure, OP, OQ and OR are drawn perpendiculars to the sides BC, CA and AB repectively of triangle ABC. Prove that :
$A R^{2} + B P^{2} + C Q^{2} = A Q^{2} + C P^{2} + B R^{2}$

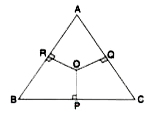
View Solution
If G is the centroid of $△ A B C,$ prove that $- - \to G A + - - \to G B + - - \to G C$ =0. Further if $G_{1}$ bet eh centroid of another $△ P Q R$ , show that $- - \to A P + - - \to B Q + - - \to C R = 3 - -- \to G G_{1}$
View Solution
In any triangle ABC, prove that $A B^{2} + A C^{2} = 2 (A D^{2} + B D^{2})$ , where D is the midpoint of BC.
View Solution
If G is the centroid of triangle with vertices $A (a, 0), B (- a, 0)$ and $C (b, c)$ then $\frac{A B^{2} + B C^{2} + C A^{2}}{G A^{2} + G B^{2} + G C^{2}} =$
View Solution

If G be the centroid of a triangle ABC, prove that,Â AB2+BC2+CA2=3(GA2+GB2+GC2)

Similar Questions

Recommended Questions