The set of all real numbers x for which x2−|x+2|+x>0 is Solution in Tamil
We have x2−|x+2|+x>0
or x2+x>|x+2| (i)
To solve the inequality, we draw the graph of y=x2+xandy=|x+2| as shown in the following figure.
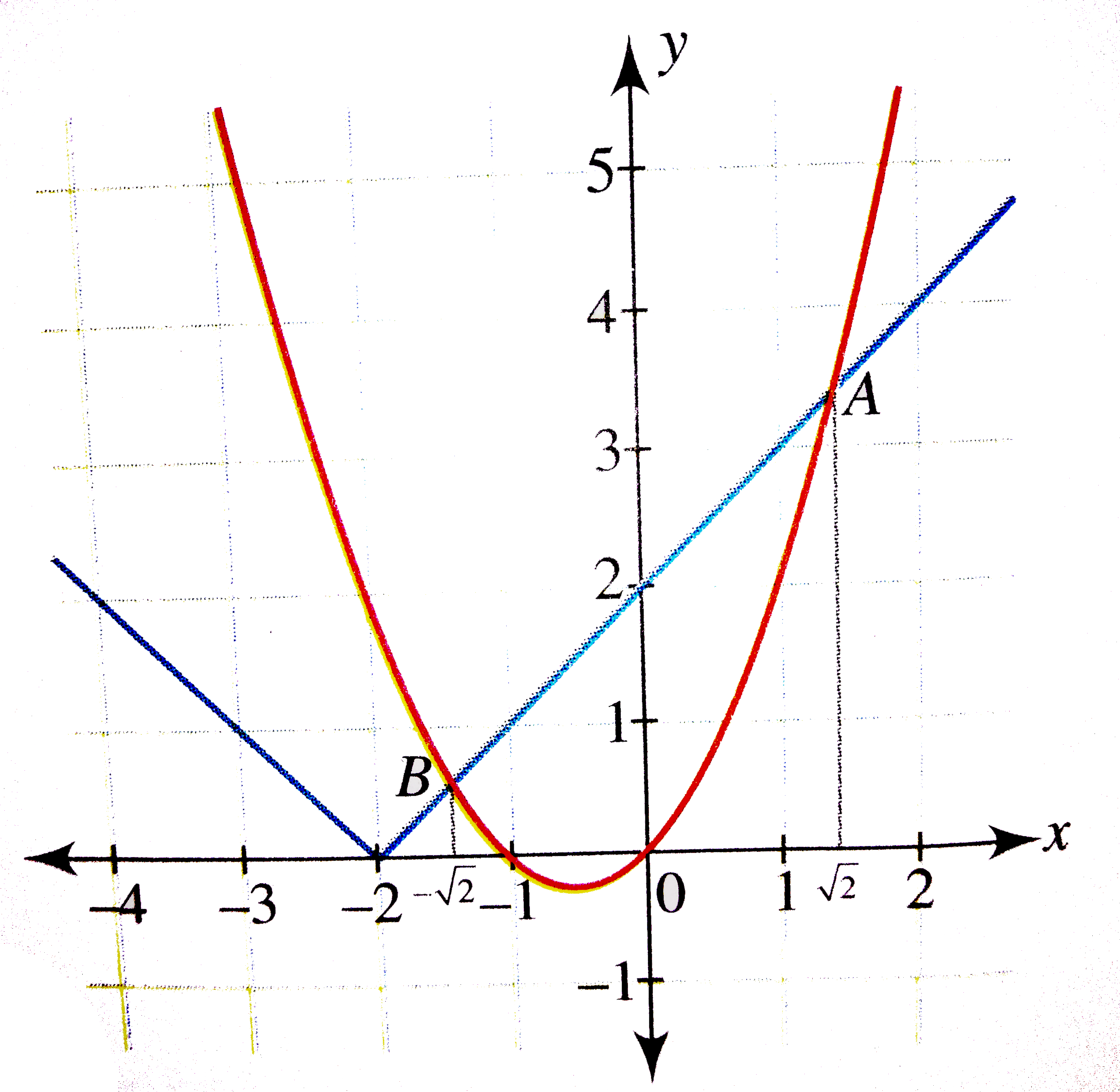
Solving y=|x+2|andy=x2+x for their points of intersection A and B, we have x+2=x2+x⇒x2=2⇒x=±√2
The solution set of (i) is the set of those values of x for which the graph of y=x2+x lies above the graph of y=x+2.
From the graph, the solution is (−∞,−√2)∪(√2,∞).
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise34videos
GRAPHS OF ELEMENTARY FUNCTIONS
- BOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises4videos
GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONS
- BOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
Similar Questions
Draw the graph of y=1(1/x).
04:37View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=006:58View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
07:12View SolutionThe set of all real numbers x for which x^(2)-|x+2|+x>0 is
03:08View SolutionThe set of all real numbers x satisfying x^(2)-|x+2|+x>0 is
03:25View SolutionIf |x^(2)-2x-8|+|x^(2)+x-2|=3|x+2| then the set of all real values of x is
02:22View SolutionIf |x^(2)-2x-8|+|x^(2)+x-2|=3|x+2| then the set of all real values of x is
05:09View SolutionWhich of the following is the empty/non-empty set
(i){x:xis a real number andx2−1=0}
{x:xis a real number andx2+1=0}
(iii){x:xis a real number andx2−9=0}
(iv){x:xis a real number and x2=x+2}07:27View SolutionAssertion (A) : {x∈R∣x2<0} is not a set. Here R is the correct of real numbers.
Reason (R ) : For every real number x,x2>0.06:10View SolutionThe set of all real numbers x, for which x2−|x+2|+x>0 is always true, is-
04:17View SolutionWhich of the following is the solution set for x(x−3)(x+2)>0?
03:42View SolutionThe set of all real values of x satisfying the inequalities x2−4x+3>0 and x2−2x−8≤0, is
05:58View Solutionসব বাস্তব সংখ্যা x এর সেট যার জন্য সর্বদা সত্য তা হল-x2−|x+2|+x>0
06:00View SolutionIf ∣∣x2−2x−8∣∣+∣∣x2+x−2∣∣=3|x+2|, then the set of all real values of x is
03:31View Solutionसभी वास्तविक संख्याओं x का वह समुच्चय जिसके लिये x2−|x+2|+x>0, होगा
02:08View SolutionThe set of all real numbers x for which x2−|x+2|+x>0 is
02:44View Solution

