The eccentricity of the ellipse 9x2+25y2−18x−100y−116=0 is 25/16 b. 4/5 c. 16/25 d. 5/4Solution in Tamil
We have 9x2+25y2−18x−100y−116=0.
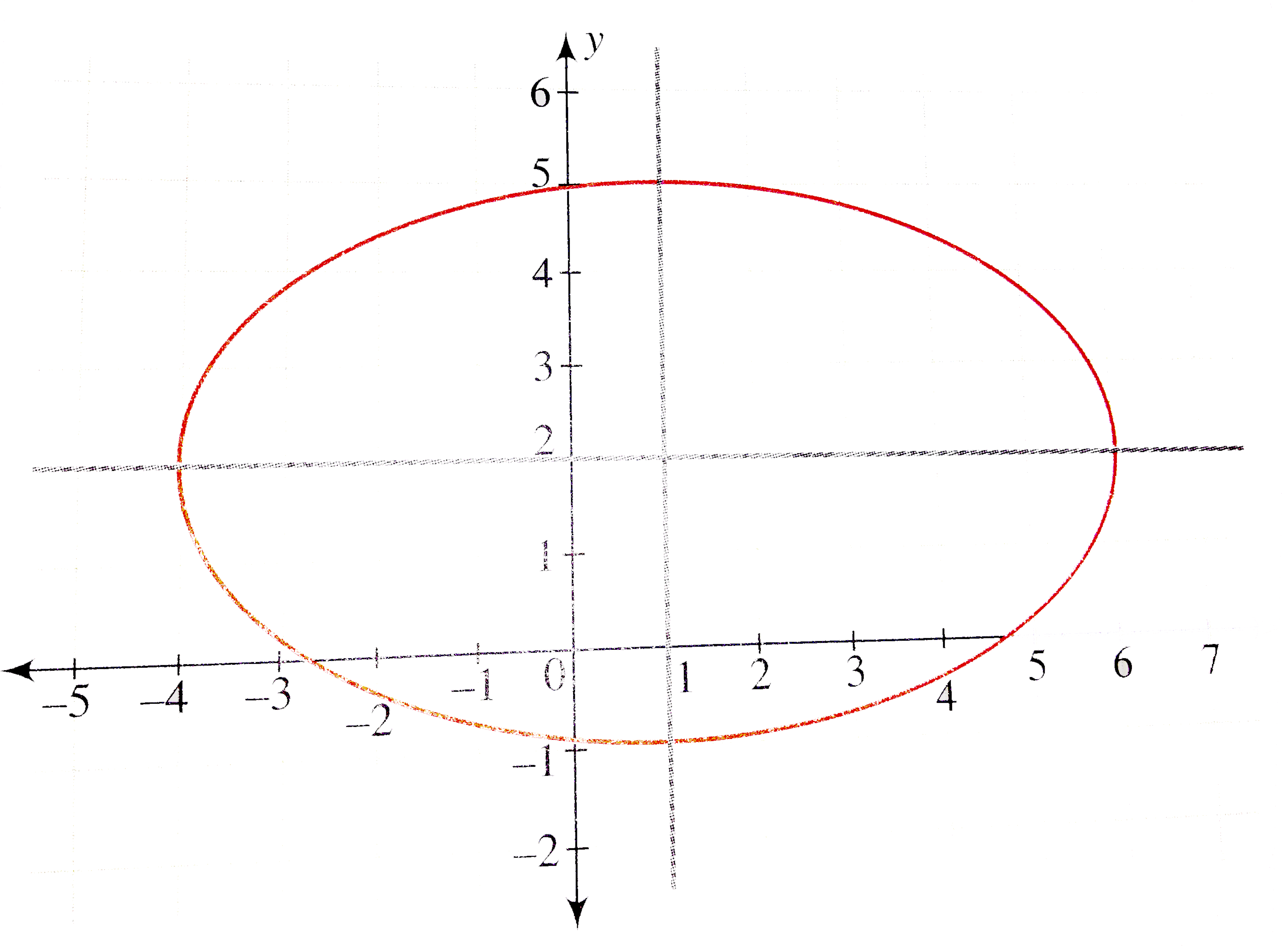
We can rewrite this as (x−)225+(y−2)29=1.
Which is an ellipse having centre at (1, 2), foci at (±4,2).
Major axis y=2 and minor axis as x=1.
The graph of the relation is as follows.
Topper's Solved these Questions
- GRAPHS OF ELEMENTARY FUNCTIONS BOOK - CENGAGECHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - Exercise
34 Videos
- GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises
4 Videos
- GRAPHS OF TRIGONOMETRIC FUNCTIONSBOOK - CENGAGECHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercise
22 Videos
Similar Questions
Draw the graph of y=1(1/x).
View SolutionSketch the graph of an example of a rational function f that satisfies all the given conditions.
(i) f(0)=0,f(1),limx→∞f(x)=0,f is odd
(ii) limx→0+f(x)=∞,limx→0−f(x)=−∞,limx→∞f(x)=1,limx→−∞f(x)=1
(iii) limx→−2f(x)=∞,limx→−∞f(x)=3,limx→∞f(x)=3,f(0)=0
View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
View SolutionThe eccentricity of the ellipse 9x2+25y2−18x−100y−116=0 is
a.25/16 b. 4/5 c. 16/25 d. 5/4
View SolutionAngle between the tangents drawn from point (4, 5) to the ellipse x216+y225=1 is
View SolutionThe equation of the ellipse having a vertex at (6,1) a focus at (4,1) and the eccentricity 35 is
1) (x−1)216+(y−1)225=1 (2) (x−1)225+(y−1)216=1
3) (x+1)225+(y+1)216=1 (4) (x+1)216+(y+1)225=1
View SolutionThe eccentricity of the ellipse x216+y225 =1 is
View SolutionIf the mid-point of a chord of the ellipse x216+y225=1 (0, 3), then length of the chord is (1) 325 (2) 16 (3) 45 12 (4) 32
View SolutionThe eccentricity of the ellipse 9x2+25y2−18x−100y−116=0 is a.2516 b. 45 c. 1625 d. 54
View SolutionThe eccentricity of the ellipse 9x2+25y2−18x−100y−116=0 is a.25/16 b. 4/5 c. 16/25 d. 5/4
View SolutionThe eccentricity of the conic 9x2−16y2=144 is a.54 b. 43 c. 45 d. √7
View Solutionदीर्घवृत्त x225+y216=1 और अतिपरवलय x225−y216=1 में उभयनिष्ठ है
View SolutionThe eccentricity of the ellipse
9x2+5y2−18x−20y−16=0 is
View SolutionThe curve x225+y216=2 touches the line x/5 + y/4 = 2 at the point
View SolutionThe eccentricity of the ellipse represented by 25x2+16y2−150x−175=0 is
View SolutionThe centre of the ellipse 9x2+25y2−18x−100y−116=0 is : a)(1,1) b)(−1,2) c)(−1,1) d)(1,2)
View Solution
