Question

If ${\displaystyle f:R\overrightarrow{N}\cup \left\{0\right\},}$
where ${\displaystyle f}$
(area of triangle joining points ${\displaystyle P(5,0),Q(8,4)andR(x,y)}$
such that angle PRQ is a right angle ${\displaystyle =}$
number of triangles, then which of the following is true?
${\displaystyle f\left(5\right)=4}$
(b) ${\displaystyle f\left(7\right)=0}$

${\displaystyle f(6,25)=2}$
(d) ${\displaystyle f\left(x\right)is\neg }$

Options

A. `f(5)=4`
B. `f(7)=0`
C. `f(6.25)=2`
D. `f(4.5)=4`

Answer

A::B::C::D

Explanation

Hence` angle PRQ=pi//2` and points P, Q, R lie on the circle with PQ as diameter.
Also, `PQ=5.`

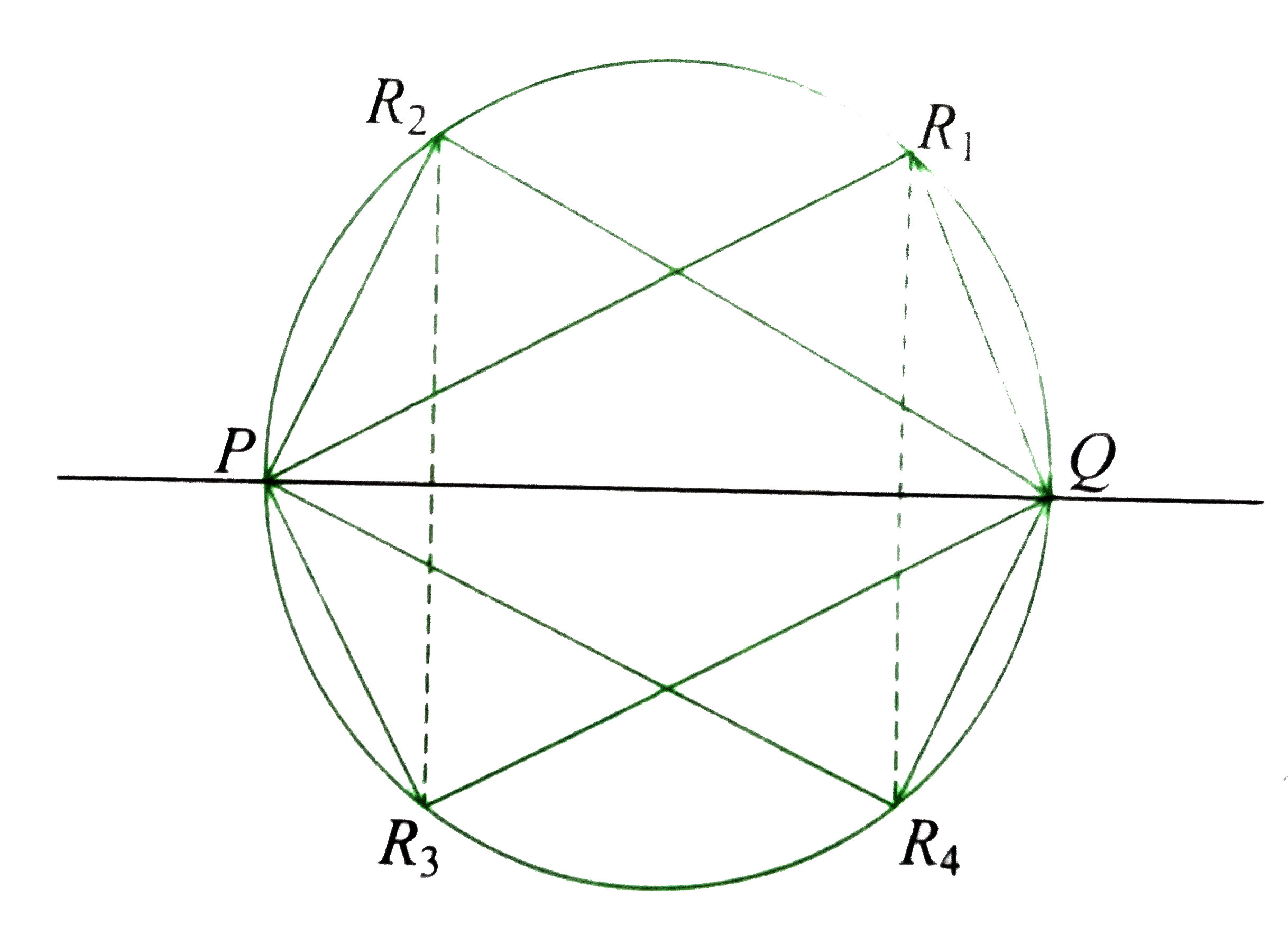
Now, the maximum area of the triangle is
`Delta_(max)=(1)/(2)(5)((5)/(2))=6.25`
For area = 5, we have four symmetrical positions of point R (shown as `R_(1), R_(2),R_(3), R_(4)`).
For area = 6.25, we have exactly two points.
For area = 7, no such points exist.