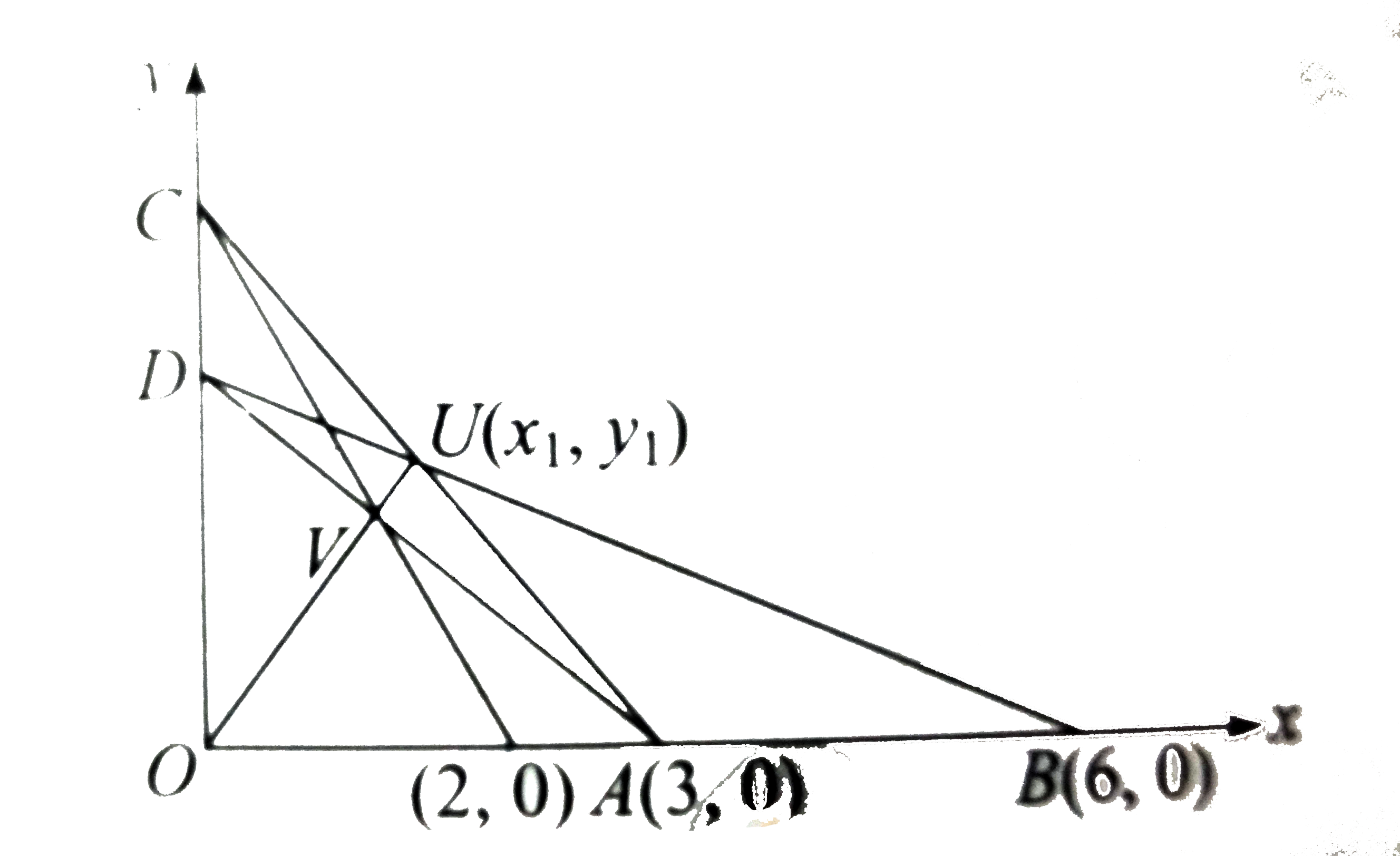
A(3,0) and B(6,0) are two fixed points and U(x1,y1) is a variable point of the plane .AU and BU meets the y axis at C and D respectively and AD meets OU at V. Then for any position of U in the plane CV passes through fixed point (p,q) whose distance from origin is____units
1units
2 units
3 units
4 units
The correct Answer is:B
Equation of AU is
y−y1=0−y13−x1(x−x1)
So that the coordinates of C are (0,3y1/(3−x1))
Similarly, the coordinates of D are (0,6y1/(6−x1))
Now, equation of AD is x3+y(6−x1)6y1=1 (i)
and equation of OU is y1x=x1y (ii)
Solving (i) and (ii), we get
x1y3y1+y(6−x1)6y1=1
⇒y(2x1+6−x1)=6y1
⇒y=6y16+x1⇒x=6x16+x1
Hence, the coordinates of V are (6x16+x1,6y16+x1)
Therefore, equation of CV is y−3y13−x1=6y16+x1−3y13−x16x16+x1−0(x−0)
⇒y=3y13−x1−9x1y16x1(3−x1)x⇒y=3y13−x1(1−x2)
which passes through the point (2,0) for all values of (x1,y1)
Topper's Solved these Questions
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Comprehension Type
3 Videos
- STRAIGHT LINEBOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type
8 Videos
- STATISTICSBOOK - CENGAGECHAPTER - STATISTICSEXERCISE - JEE Previous Year
10 Videos
- STRAIGHT LINESBOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - JEE Advanced Previous Year
4 Videos
Similar Questions
A(3,0) and B(6,0) are two fixed points and U (α,β) is a variable point on the plane ,AU and BU meet the y - axis at C and D respectively and Ad meets OU at V. Prove that CV passes through (2,0) for any position of U in the plane .
View SolutionFind the equation of the plane passing through the points (1,0,0) and (0,2,0) and c at a distance 6/7 units from the origin
View Solution
Knowledge Check
An equation of the plane through the points (1,0,0), (0,2,0) and at a distance 6/7 units from the origin, is
A6x+3y+z−6=0B6x+3y+2z−6=0C6x+3y+z+6=0D6x+3y+2z+6+0If a,b,c are in H.P then the straight line xa+yb+1c=0 always passes through the fixed point whose coordinates are
A(-1,-2)B(-1,2)C(1,-2)D(1,−1/2)A variable plane passes through a fixed point (1,−2,3) and meets the coordinate axes at points A, B, C then the point of intersection of the planes through A, B, C parallel to the coordinate planes lies on:
Axy−(1/2)yz+(1/3)zx=6Byz−2zx+3xy=xyzCxy−2yz+3zx=3xyzDxy+(1/2)yz−(1/3)zx=6
A tangent to the ellipes x225+y216=1 at any points meet the line x=0 at a point Q Let R be the image of Q in the line y=x, then circle whose extremities of a dameter are QandR passes through a fixed point, the fixed point is
View SolutionA variable plane passes through a fixed point (a,b,c) and meets the axes at A,B,andC. The locus of the point commom to the planes through A,BandC parallel to the coordinate planes is
View SolutionIf from a variable point 'P' on the line 2x−y−1=0 pair of tangent's are drawn to the parabola x2=8y then prove that chord of contact passes through a fixed point, also find that point.
View SolutionA variable plane passes through a fixed point (1,−2,3) and meets the coordinate axes at point A, B, C then the point of intersection of the planes through A, B, C parallel to the coordinate planes lies on
View SolutionA variables plane passes through the point (1,2,3) and meets the coordinate axis is P,Q,R. Then the locus of the point common to the planes P,Q,R parallel to coordinates planes
View Solution
