Question

Let ${\displaystyle A(a,0)\text{and}B(b,0)}$ be fixed distinct points on the x-axis, none of which coincides with the ${\displaystyle O(0,0)}$, and let C be a point on the y-axis. Let L be a line through the ${\displaystyle O(0,0)}$ and perpendicular to the line AC. The locus of the point of intersection of the lines L and BC if C varies along is (provided ${\displaystyle c^2+ab\ne 0}$)

Options

A. `(x^(2))/(a)+(y^(2))/(b) =x`
B. `(x^(2))/(a)+(y^(2))/(b) =y`
C. `(x^(2))/(b)+(y^(2))/(a)=x`
D. `(x^(2))/(b)+(y^(2))/(a) =y`

Answer

C

Explanation

Equation of the line L is `y = (a)/(c)x`
As (h,k) lies on it, hence
`k = (a)/(c )h` (i)
Now equation of BC
`(x)/(b)+(y)/(c ) =1`
(h,k) lies on it
`:. (h)/(b) +(k)/(c ) = 1`

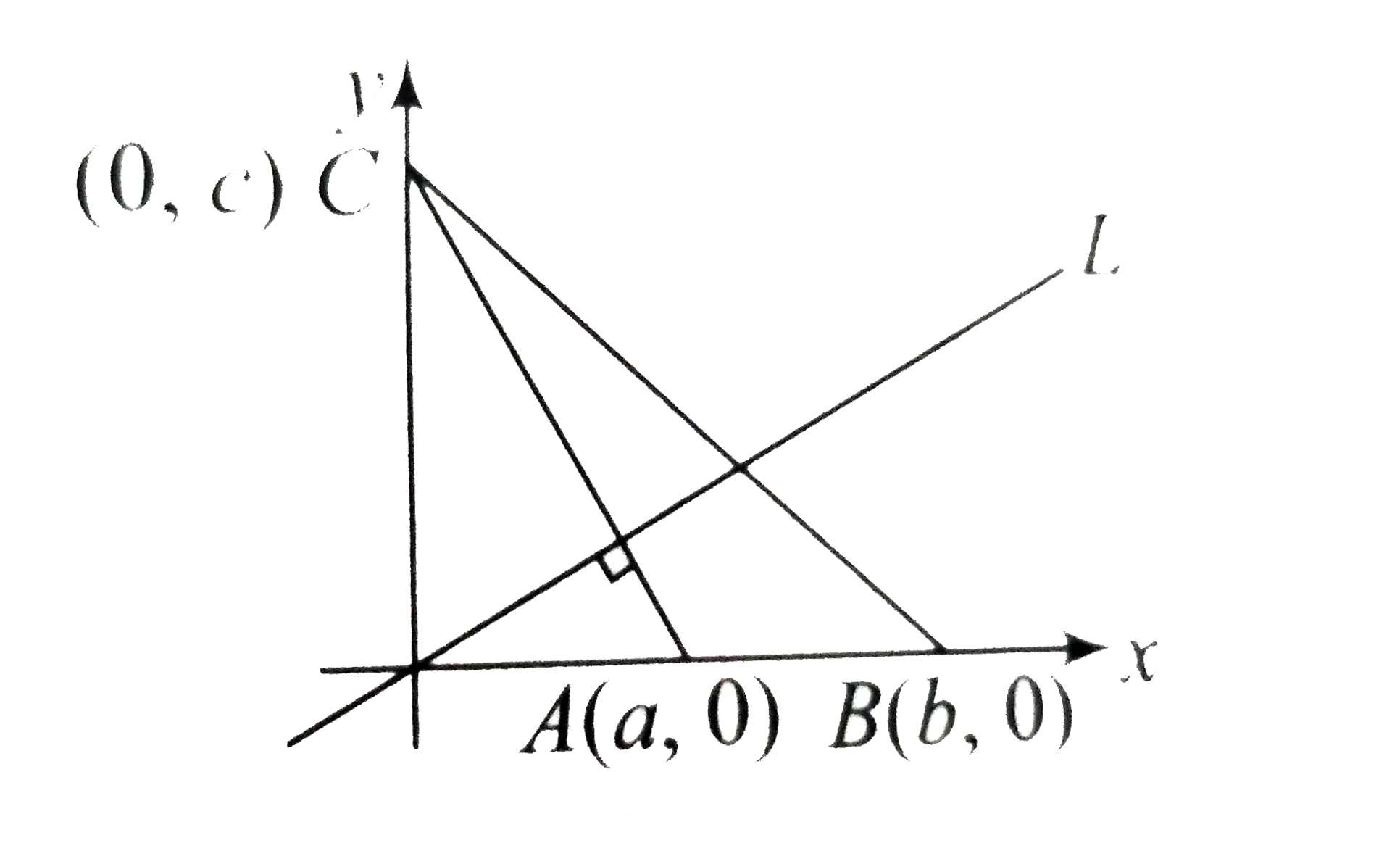
Substituting `c = (ah)/(k)` in (ii) from (i)
`(h^(2))/(hb) +(k^(2))/(ah) =1`
`:.` Locus of P is `(x^(2))/(b) +(y^(2))/(a) = x`