Suppose a parabola y=x2−ax−1 intersects the coordinate axes at three points A,B and C, respectively. The circumcircle of ΔABC intersects the y-axis again at the point D(0,t). Then the value of t is Solution in Tamil
1/2
1
3/2
2
The correct Answer is:B
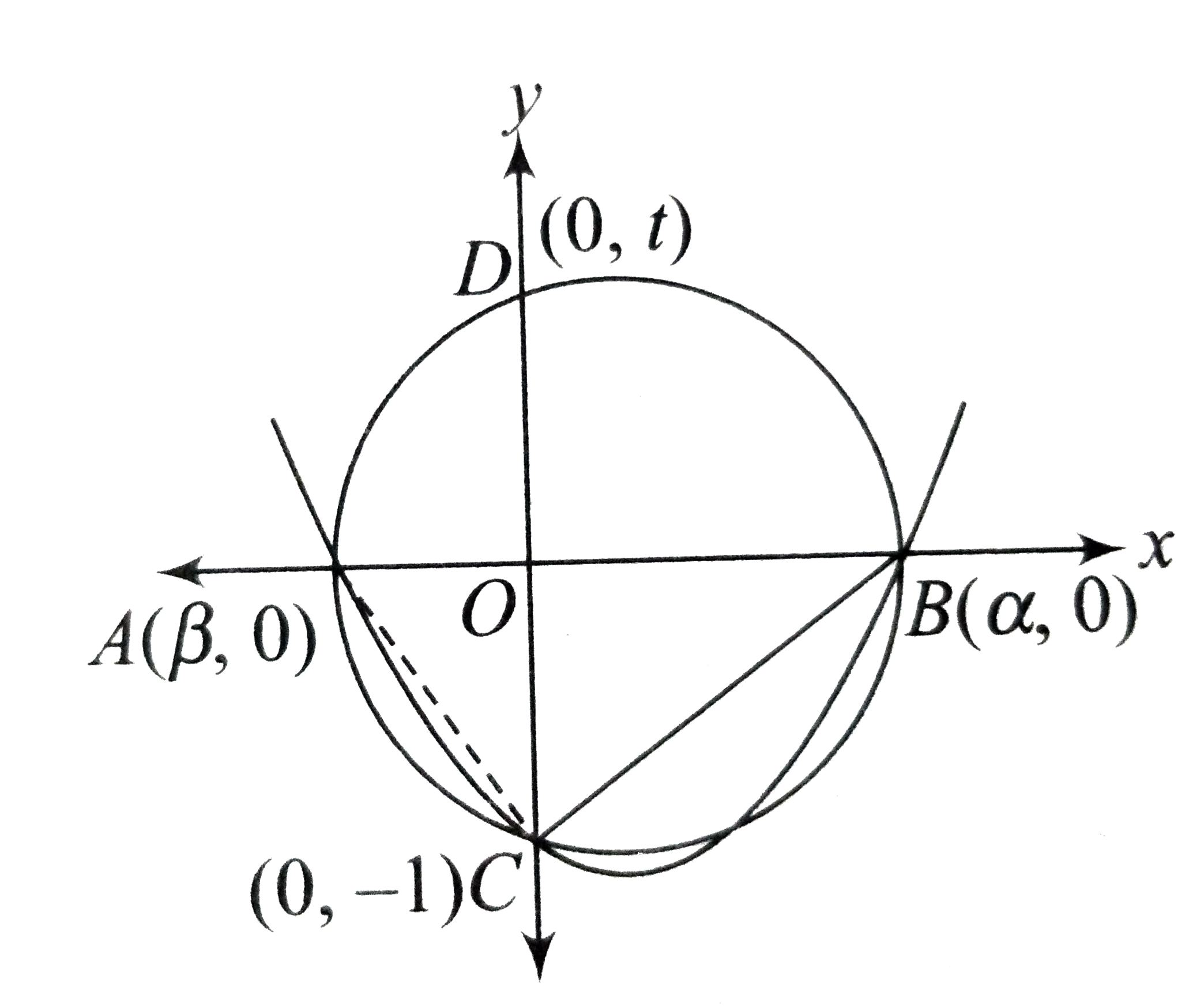
We have x=a±√a2+42
∴α=a+√a2+42,β=a−√a2+42
Equation of family of circles through A and B is
(x−α)(x−β)+y2+λy=0
As it passes through C(0,−1) we have
αβ+1−λ=0 (But αβ=−1)
⇒−1+1−λ=0
⇒λ=0
∴ Equation of circle through A,B and C is
(x−α)(x−β)+y2=0
It cuts the y-axis when x=0, so
αβ+y2=0
y2=1 (Put αβ=−1)
⇒y=1 or −1
Hence t=1
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Multiple Correct Answers Type10videos
PARABOLA
- BOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Comprehension Type1videos
PARABOLA
- BOOK - CENGAGECHAPTER - PARABOLAEXERCISE - JEE Advenced Single Answer Type18videos
PARABOLA
- BOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise (Numerical)5videos
PAIR OF STRAIGHT LINES
- BOOK - CENGAGECHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Question Bank4videos
PERMUTATION AND COMBINATION
Similar Questions
A family of curve S is given by S≡x2+2xy+y2−4x(1−λ)−4y(1+λ)+4, then S=0 represents
04:07View SolutionA parabola is drawn through two given points A(1,0) and B(−1,0) such that its directrix always touches the circle x²+y2=4. Then, The locus of focus of the parabola is=
06:30View Solutionabout to only mathematics
04:41View SolutionThe distance between the lines (x+7y)2+4√2(x+7y)−42=0 is_____________
06:47View SolutionIf the number of ways in which a selection of 100 balls can be made out of 100 identical red balls, 100 identical blue balls and 100 identical white balls is
07:03View SolutionSuppose that three points on the parabola y=x^(2) have the property that their normal lines intersect at a common point (a,b).The sum of their x-coordinates is
03:35View SolutionAn ellipse with axes parallel to coordinate axes cuts the parabola y^(2)=4x at (1,-2) and touches itat (4,4) then the coordinate of other point of intersection is
01:40View SolutionLine x−y=1 intersect the parabola y2=4x at A and B. Normals at A and B intersect at C. If D is the point at which line CD is normal to the parabola, then coordinate of point D is
03:14View SolutionTangent to the parabola y=x2+ax+1 at the point of intersection of the y-axis also touches the circle x2+y2=r2. Also, no point of the parabola is below the x-axis.
The minimum area bounded by the tangent and the coordinate axes is05:43View SolutionSuppose a parabola y=x2−ax−1 intersects the coordinate axes at three points A,B and C, respectively. The circumcircle of ΔABC intersects the y-axis again at the point D(0,t). Then the value of t is
06:01View SolutionDraw the graphs of 2x + y = 6 and y = 2x + 2 intersecting X and Y axes in the points A, B and C, D respectively. The point of intersection is E. Find the area of (a) ΔAEC (b) ΔEDB
06:59View Solutionमान लीजिये रेखा y = x परवलय y=ax2+c की स्पर्श रेखा है।
यदि (1, 1) प्रतिच्छेद बिंदु हो, तो a का मान होगाView Solutionमान लीजिये रेखा y = x परवलय y=ax2+c की स्पर्श रेखा है।
यदि c=2 तब प्रतिच्छेद बिंदु होगा01:53View SolutionThe locus of point of intersection of lines xta−yb+t=0 and xat+yb=1t
05:35View SolutionIf (xa)+(yb)=1 and (xc)+(yd)=1 intersect the axes at four concylic points and a2+c2=b2+d2, then these lines can intersect at, (a,b,c,d>0)
08:43View Solution

