Construct a quadrilateral BEAR in which BE = 6 cm, EA = 7 cm, RB = RE = 5 cm and BA = 9 cm. Measure its fourth side.
To construct the quadrilateral BEAR with the given dimensions, follow these steps:
1. Draw the Base BE:
- Start by drawing a line segment BE that measures 6 cm.
- Label the endpoints as B and E.
2. Construct Point R:
- Place the compass point on B and open it to a radius of 5 cm.
- Draw an arc above or below the line segment BE. This arc represents all possible locations for point R that is 5 cm away from B.
3. Construct Point A:
- Now, place the compass point on E and open it to a radius of 7 cm.
- Draw another arc from point E. This arc represents all possible locations for point A that is 7 cm away from E.
4. Construct Point A with BA:
- Next, place the compass point back on B and open it to a radius of 9 cm.
- Draw an arc from point B. This arc represents all possible locations for point A that is 9 cm away from B.
5. Locate Point A:
- The intersection of the two arcs (from steps 3 and 4) gives you the position of point A. Label this intersection as A.
6. Construct Point R:
- Now, to find point R, place the compass point on E and open it to a radius of 5 cm.
- Draw an arc from point E. The intersection of this arc with the arc drawn from point B (step 2) gives you the position of point R. Label this intersection as R.
7. Connect the Points:
- Finally, connect the points B, E, A, and R to form the quadrilateral BEAR.
- Ensure that the lengths of the sides are as follows: BE = 6 cm, EA = 7 cm, RB = 5 cm, RE = 5 cm, and BA = 9 cm.
8. Measure the Fourth Side:
- To find the length of side AR, measure the distance between points A and R using a ruler.
- Start by drawing a line segment BE that measures 6 cm.
- Label the endpoints as B and E.
- Place the compass point on B and open it to a radius of 5 cm.
- Draw an arc above or below the line segment BE. This arc represents all possible locations for point R that is 5 cm away from B.
- Now, place the compass point on E and open it to a radius of 7 cm.
- Draw another arc from point E. This arc represents all possible locations for point A that is 7 cm away from E.
- Next, place the compass point back on B and open it to a radius of 9 cm.
- Draw an arc from point B. This arc represents all possible locations for point A that is 9 cm away from B.
- The intersection of the two arcs (from steps 3 and 4) gives you the position of point A. Label this intersection as A.
- Now, to find point R, place the compass point on E and open it to a radius of 5 cm.
- Draw an arc from point E. The intersection of this arc with the arc drawn from point B (step 2) gives you the position of point R. Label this intersection as R.
- Finally, connect the points B, E, A, and R to form the quadrilateral BEAR.
- Ensure that the lengths of the sides are as follows: BE = 6 cm, EA = 7 cm, RB = 5 cm, RE = 5 cm, and BA = 9 cm.
- To find the length of side AR, measure the distance between points A and R using a ruler.
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - APPLICATIONS, GAMES AND PUZZLES
3 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYBOOK - NCERT EXEMPLARCHAPTER - UNDERSTANDING QUADRILATERALS AND PRACTICAL GEOMETRYEXERCISE - THINK AND DISCUSS
2 Videos
- SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTBOOK - NCERT EXEMPLARCHAPTER - SQUARE - SQUARE ROOT AND CUBE-CUBE ROOTEXERCISE - Exercise
52 Videos
- VISUALISING SOLID SHAPES BOOK - NCERT EXEMPLARCHAPTER - VISUALISING SOLID SHAPES EXERCISE - EXERCISE
91 Videos
Similar Questions
Construct a quadrilateral PQRS in which PQ=6cm, QR=7.5cm , PR=10.5cm, PS=4.5cm and QS=9cm.
View SolutionConstruct a quadrilateral ABCD in which AB=3.8cm, BC=5cm,CD=5cm, DA=5.5cm and AC=8cm.
View SolutionConstruct a quadrilateral ABCD in which AB = AD = 5cm, BC = CD = 7cm and BD = 6cm. What type of quadrilateral is this?
View SolutionConstruct a quadrilateral ABCD in which AB=5cm, Ac=6cm, AD=8cm and ∠ABC=∠ACD=90∘.
View SolutionIs it possible to construct a quadrilateral ABCD in which AB = 3 cm, BC = 4 cm, CD = 5.4 cm, DA = 5.9 cm and diagonal AC = 8 cm? If not, why?
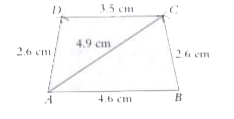
View SolutionConstruct a quadrilateral ABCD in which AB = 4 . 6 cm, BC = 2.6 cm, CD = 3.5 cm AD = 2 . 6 cm, and the diagonal AC = 4 . 9 cm
View SolutionConstruct a quadrilateral ABCD in which AB=2.9cm,BC=3.7cm, CD=4.2cm, AD=6.1 and ∠B=90∘.
View SolutionConstruct a quadrilateral ABCD in which AB=4cm, BC=6cm, CD=6cm, ∠B=120∘ and ∠C=90∘.
View SolutionConstruct a quadrilateral NEWS in which NE = 7cm, EW = 6 cm, ∠N = 60°, ∠E = 110° and ∠S = 85°.
View SolutionConstruct a rhombus CLUE in which CL = 7.5 cm and LE = 6 cm.
View Solution
