Question

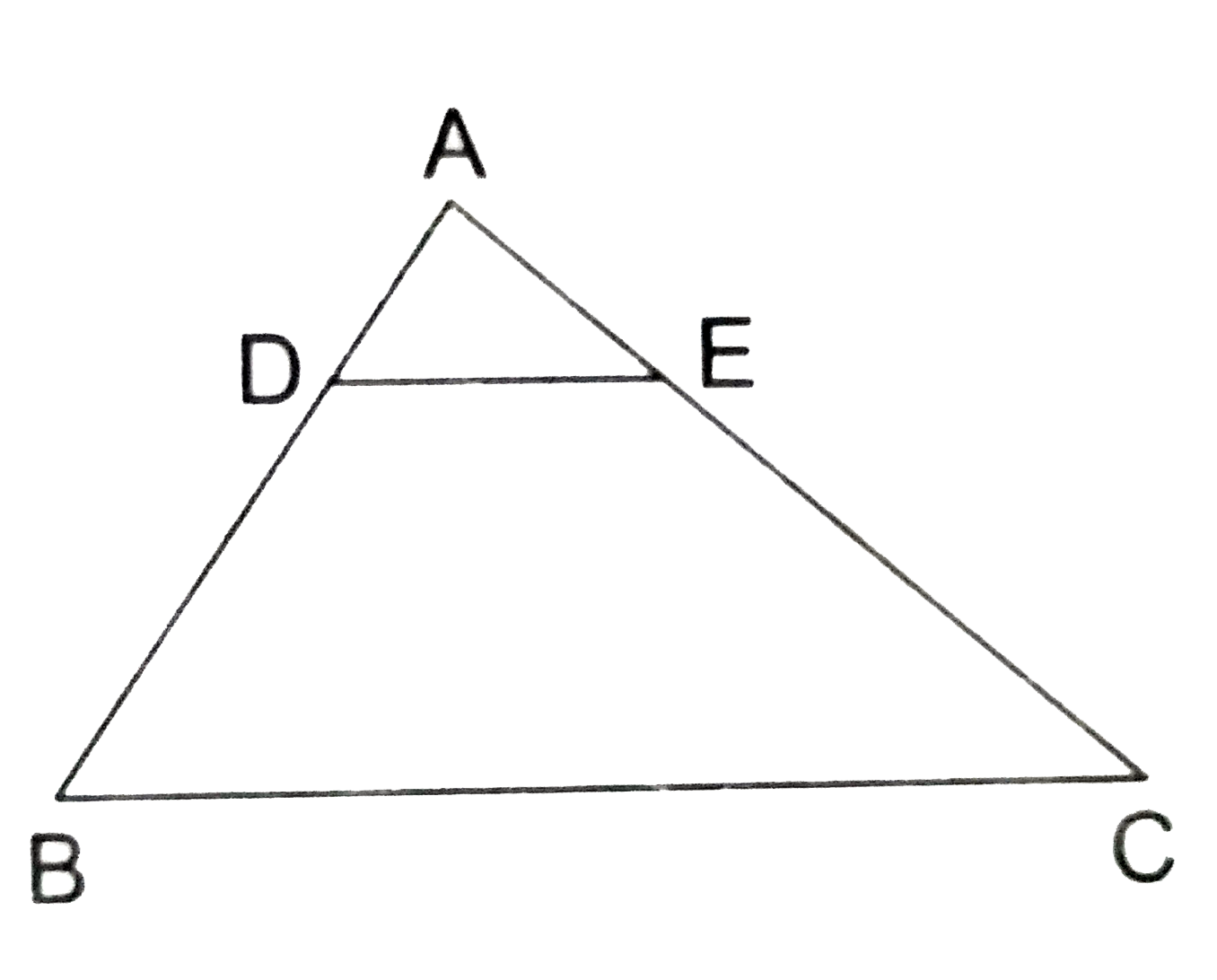
In ${\displaystyle △ABC}$ ,D and E are the points on sides AC and AB, respectively, such that ${\displaystyle \angle ADE=\angle B}$. If AD = 7.6 cm, AE = 7.2 cm, BE = 4.2 cm and BC = 8.4 cm, then DE is equal to:
त्रिभुज ABC में, D तथा E भुजा AC और AB पर स्थित ऐसे बिंदु ${\displaystyle \angle ADE=\angle B}$ है| यदि AD =7.6 सेमी, AE = 7.2 सेमी, BE = 4.2 सेमी तथा BC = 8.4 सेमी है, तो DE किसके बराबर है ?

Options

A. 6.3 cm
B. 5.8 cm
C. 7.4 cm
D. 5.6 cm

Explanation

To solve the problem, we will use the concept of similar triangles. Given that $$\angle ADE=\angle B$$, we can conclude that triangles $$ADE$$ and $$ABC$$ are similar.

1. Identify the triangles and given values:
- We have triangle \( ABC \) and points \( D \) and \( E \) on sides \( AC \) and \( AB \) respectively.
- Given values:
- \( AD = 7.6 \, \text{cm} \)
- \( AE = 7.2 \, \text{cm} \)
- \( BE = 4.2 \, \text{cm} \)
- \( BC = 8.4 \, \text{cm} \)

2. Calculate \( AB \):
- Since \( AB = AE + BE \):
\( AB = 7.2 + 4.2 = 11.4 \, \text{cm} \)

3. Set up the proportion using similar triangles:
- From the similarity of triangles \( ADE \) and \( ABC \):
\( \frac{AD}{AB} = \frac{DE}{BC} \)
- Substituting the known values:
\( \frac{7.6}{11.4} = \frac{DE}{8.4} \)

4. Cross-multiply to solve for \( DE \):
- Cross-multiplying gives us:
\( 7.6 \times 8.4 = 11.4 \times DE \)

5. Calculate \( 7.6 \times 8.4 \):
- Performing the multiplication:
\( 7.6 \times 8.4 = 63.84 \)

6. Now solve for \( DE \):
- Rearranging the equation:
\( DE = \frac{63.84}{11.4} \)

7. Calculate \( DE \):
- Performing the division:
\( DE = 5.6 \, \text{cm} \)

Final Answer:
Thus, the length of \( DE \) is \( 5.6 \, \text{cm} \).

---