For a particle in uniform circular motion the acceleration a at a pont P(R,θ) on the circle of radius R is (here θ is measured from the x-axis)
−v2Rcosθˆi+v2Rsinθˆj
−v2Rsinθˆi+v2Rsinθˆj
−v2Rcosθˆj−v2Rsinθˆj
v2Rˆi+v2Rˆj
The correct Answer is:C
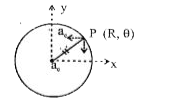
For a particle in uniform circular motion
a=v2R towards centre of circle a=v2R(−cosθˆi−sinθˆj),a=−v2Rcosθˆi−v2Rsinθˆj
Similar Questions
For a particle in uniform circular motion the acceleration →a at a point P(R,θ) on the circle of radius R is : ( here θ is measured from the x- axis )
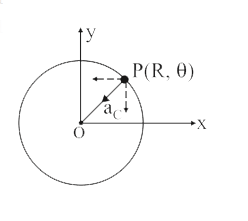
View SolutionFor a particle in uniform circular motion , the acceleration →a at a point p(R,θ) on the circle of radiu R is ( Here θ is measured from the x−aξs )
View Solution
Knowledge Check
For a particle in uniform circular motion the acceleration →a at a point P(R,θ) on the circle of radius R is : ( here θ is measured from the x- axis )
 A−v2Rcosθˆi+v2RsinθˆjB−v2Rsinθˆi+v2RcosθˆjC−v2Rcosθhai−v2RsinθˆjDv2Rˆi+v2Rˆj
A−v2Rcosθˆi+v2RsinθˆjB−v2Rsinθˆi+v2RcosθˆjC−v2Rcosθhai−v2RsinθˆjDv2Rˆi+v2RˆjFor a particle in uniform circular motion , the acceleration →a at a point p(R,θ) on the circle of radiu R is ( Here θ is measured from the x−aξs )
A−v2Rcosθˆi+v2RsinθˆjB−v2Rsinθˆi+v2RcosθˆjC−v2Rcosθˆi−v2RsinθˆjD−v2Rˆi+v2RˆjFor a particle performing a uniform circular motion the acceleration is
Aconstant in directionBconstant in magnitude but not in directionCconstant in magnitude and directionDconstant neither in magnitude nor in direction.
For a particle in uniform circular motion, the acceleration →a at a point P(R,θ) on the circle of radius R (Here is measured from the x-axis) is
View SolutionFor a particle in uniform circular motion the acceleration a at a point P(R,θ) on the circle of radius R is (here 'v' is the speed of the particle and θ is measured from the x-axis)
View SolutionIn a non uniform circular motion, the acceleration on the particle is
View SolutionFor a particle in a non-uniform accelerated circular motion
View SolutionThe area of a sector of angle θ∘ of a circle with radius R is
View Solution
