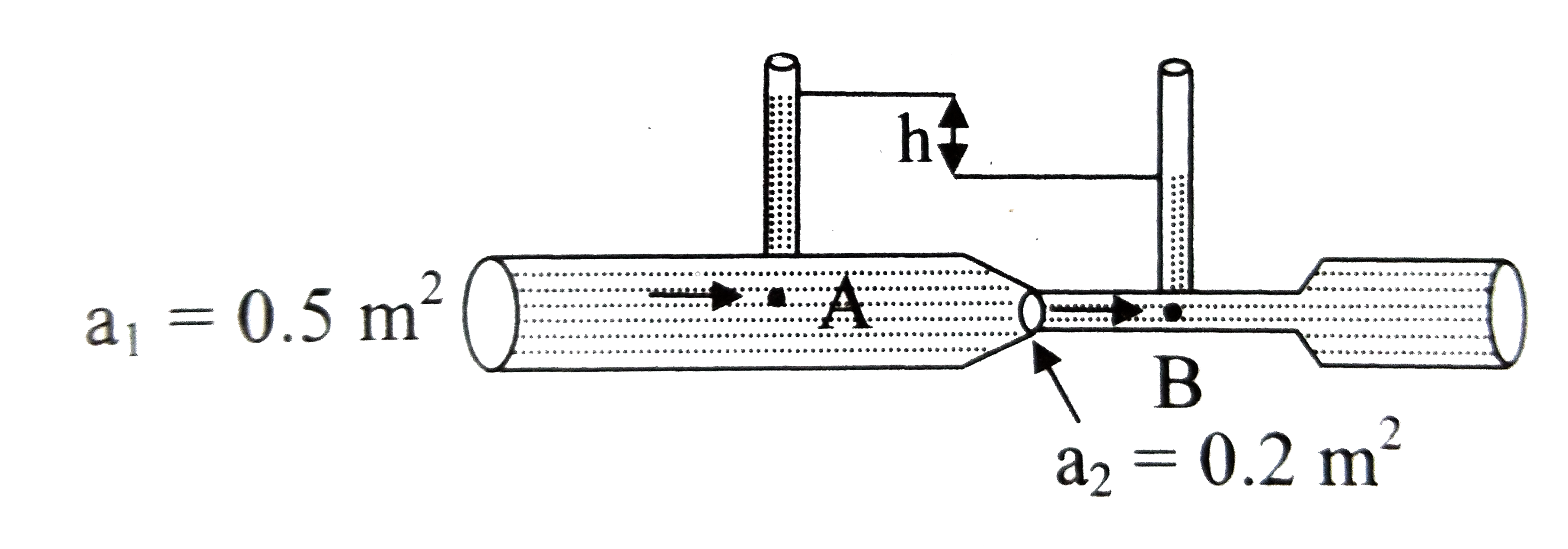
A liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross section A1 and A2 are v1 and v2 respectively. The difference in the levels of the liquid in the two vertical tubes is h. Then
 Solution in Gujrati
Solution in Gujrati
The volume of the liquid flowing through the tube in unit time is A1v1
v2−v1=√2gh
v22−v21=2gh
The energy per unit mass of the liquid is the same in both sections of the tube.
The correct Answer is:A, C, D
Step by step video & image solution for A liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross section A_(1) and A_(2) are v_(1) and v_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h. Then by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 3 (Assertion & Reason)32videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 3 (Comprehension based questions)23videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 1 (Fluid Statics)40videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B19videos
CENTRE OF MASS
- BOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)22videos
ERROR AND MEASUREMENT
Similar Questions
Statement-1: A solid sphere and a hollow sphere of same material are floating in a liquid radius of both the spheres are same percentage of volume immersed of both the spheres will be same.
Statement-2: Upthust acts on volume of liquid displaced. It has nothing to do whether the body is solid or hollow.04:54View SolutionIf the container filled with liquid gets accelerated horizontally or vertically, pressure in liquid gets changed. In liquid (ay) for calculation of pressure, effective g is used. A closed box horizontal base 6m by 6m and a height 2m is half filled with liquid. It is given a constant horizontal acceleration g/2 and vertical downward acceleration g/2.
The angle of the free surface with the horizontal is equal to08:10View SolutionA crown made of gold and copper weights 210g in air and 198 g in water the weight of gold in crown is
[Given, Density of gold =19.3g/cm2 and density of copper =8.5g/cm3]04:31View SolutionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
03:10View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
01:59View SolutionFigure shows a liquid flowing through a tube at the rate of 0.1 m^(3)//s . The tube is branched into two semicircular tubes of cross-sectional area A//3 and 2A//3 . The velocity of liquid at Q is (the cross section of the main tube is A =10^(-2) m^(2) and v_(p) = 20 m//s )
02:46View SolutionA horizontal tube has different cross sections at points A and B. The areas of cross section are a1 and a2 respectively, and pressures at these points are p1=ρgh1 and p2=ρgh2 where ρ is the density of liquid flowing in the tube and h1 and h2 are heights of liquid columns in vertical tubes connected at A and B. If h1−h2=h, then the flow rate of the liquid in the horizontal tube is
01:59View SolutionA liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross section A_(1) and A_(2) are v_(1) and v_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h . Then
02:28View SolutionA liquid flows through a horizontal tube as shown in figure. The velocities of the liquid in the two sections, which have areas of cross-section A1 and A2 and v1 and v2 respectively. The difference in the levels of the liquid in the two vertical tubes is h, then
 02:09View Solution
02:09View SolutionA liquid flows in a tube from left to right as shown in figure. A_(1) and A_(2) are the cross-section of the portions of the tube as shown. Then the ratio of speeds v_(1)//v_(2) will be
View SolutionFrom a horizontal tube with area of cross-section A_(1) and A_(2) as shosn in fiugre liquid is flowing in the level of the liauid in the two veritcal tunes is h.
View SolutionIf cross- sectional area of limb I is A1 and that of limb II is A2 then velocity of the liquid in the tube will be, (cross- sectional area of tube is very small)
 03:42View Solution
03:42View SolutionA liquid flows through a pipe of non-uniform cross-section. If A_(1) and A_(2) are the cross-sectional areas of the pipe at two points, the ratio of velocities of the liquid at these points will be
03:12View SolutionAn incompressible, non-viscous liquis of density r flows through a horizontal tube as shown below. The flow condition is steady. Area of cross section at A and B are a1anda2 respectively. The height difference of liquid in two tubes inserted at A and B is h.
 03:32View Solution
03:32View SolutionA liquid flows through a horizontal tube. The velocities of the liquid in the two sections, which have areas of cross - section A_(1) and A_(2) are upsilon_(1)andupsilon_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h. a) The volume of the liquid flowing through the tube in unit time is A_(1)upsilon_(1) . b) upsilon_(2)-upsilon_(1)=sqrt(2gh) c) upsilon_(2)^(2)-upsilon_(1)^(2)=2gh d) The energy per unit mass of the liquid is the same in both sections of the tube.
04:00View SolutionA liquid flows through a horizontal tube. The velocities of the liquid ind the two sections, which have areas of cross -section A_(1) and A_(2) are v_(1) and v_(2) respectively. The difference in the levels of the liquid in the two vertical tubes is h. a. The volume of the liquid flow through the tube in unit tims is A_(1)v_(1) b. v_(2)-v_(1)=sqrt(2gh) c. v_(2)^(2)-v_(1)^(2)=2gh d. The energy per unit mass of the liquid is the same in both sections of the tube.
02:35View Solution

