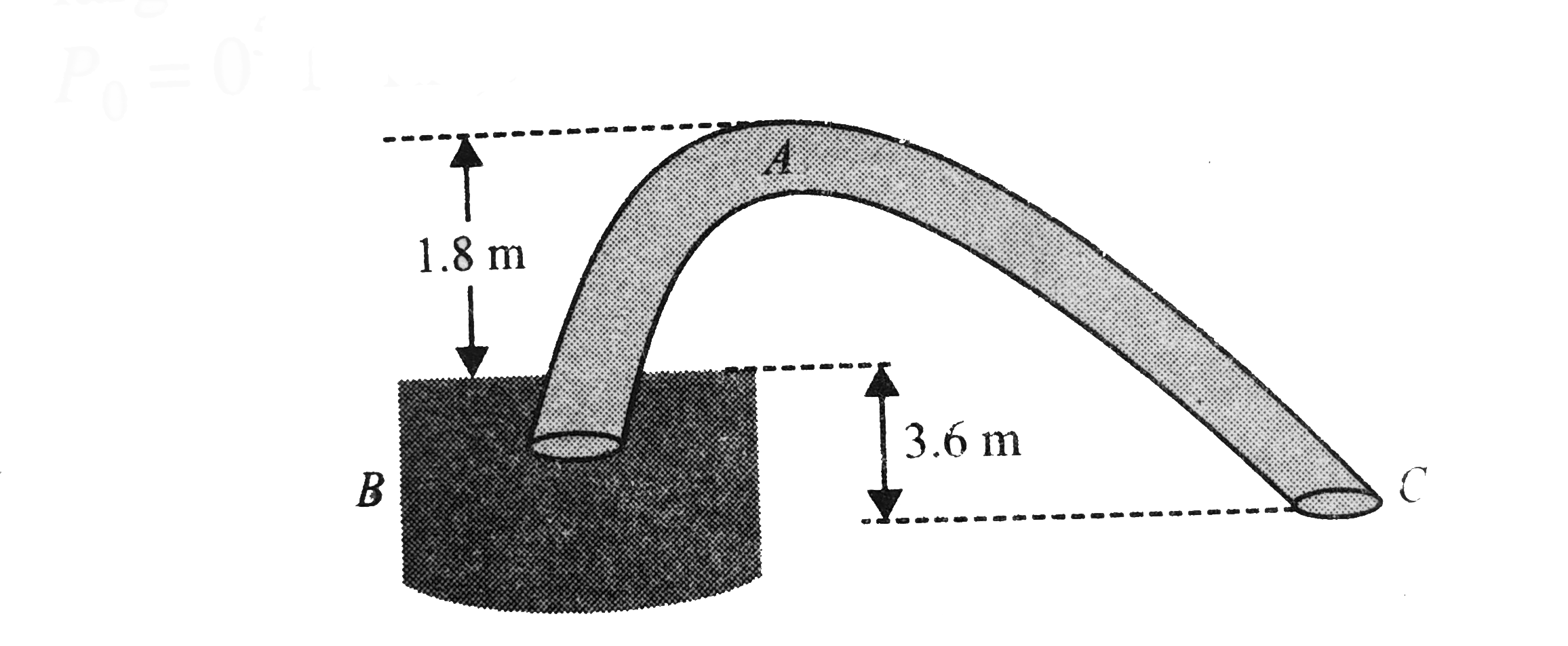
A siphon has a uniform circular base of diameter 8/√πcm with its crest A,1.8m above the water level vessel B is of large cross section (g=10m/s2 and atmospheric pressure P0=105N/m2).
 Solution in Gujrati
Solution in Gujrati
The correct Answer is:(i). 6√2m/s,(ii).9.6√2×10−3m3/s,(iii).4.6×104N/m2
Step by step video & image solution for A siphon has a uniform circular base of diameter 8//sqrt(pi) cm with its crest A, 1.8 m above the water level vessel B is of large cross section (g= 10 m//s^(2) and atmospheric pressure P_(0) = 10^(5) N//m^(2)). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 A (Viscosity)3videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 B (Bran storming Subjective Exercise)11videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - ELASTICITY, SURFACE TENSION AND FLUID MECHANICSEXERCISE - Exercise 4 A (Fluid Statics)13videos
ELASTICITY, SURFACE TENSION AND FLUID MECHANICS
- BOOK - ALLEN CHAPTER - CENTRE OF MASSEXERCISE - EXERCISE-V B19videos
CENTRE OF MASS
- BOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)22videos
ERROR AND MEASUREMENT
Similar Questions
A flask contains glycerine and the other contains water. Both are stirred vigorously and placed on the table. In which flask will the liquid come to rest earlier and why?
06:07View SolutionA container of large uniform cross-sectional area A resting on a horizontal surface, holes two immiscible, non-viscon and incompressible liquids of densities d and 2d each of height H/2 as shown in the figure. The lower density liquid is open to the atmosphere having pressure P0. A homogeneous solid cylinder of length L(L<H/2) and cross-sectional area A/5 is immeresed such that it floats with its axis vertical at the liquid-liquid interface with length L/4 in the denser liquid,
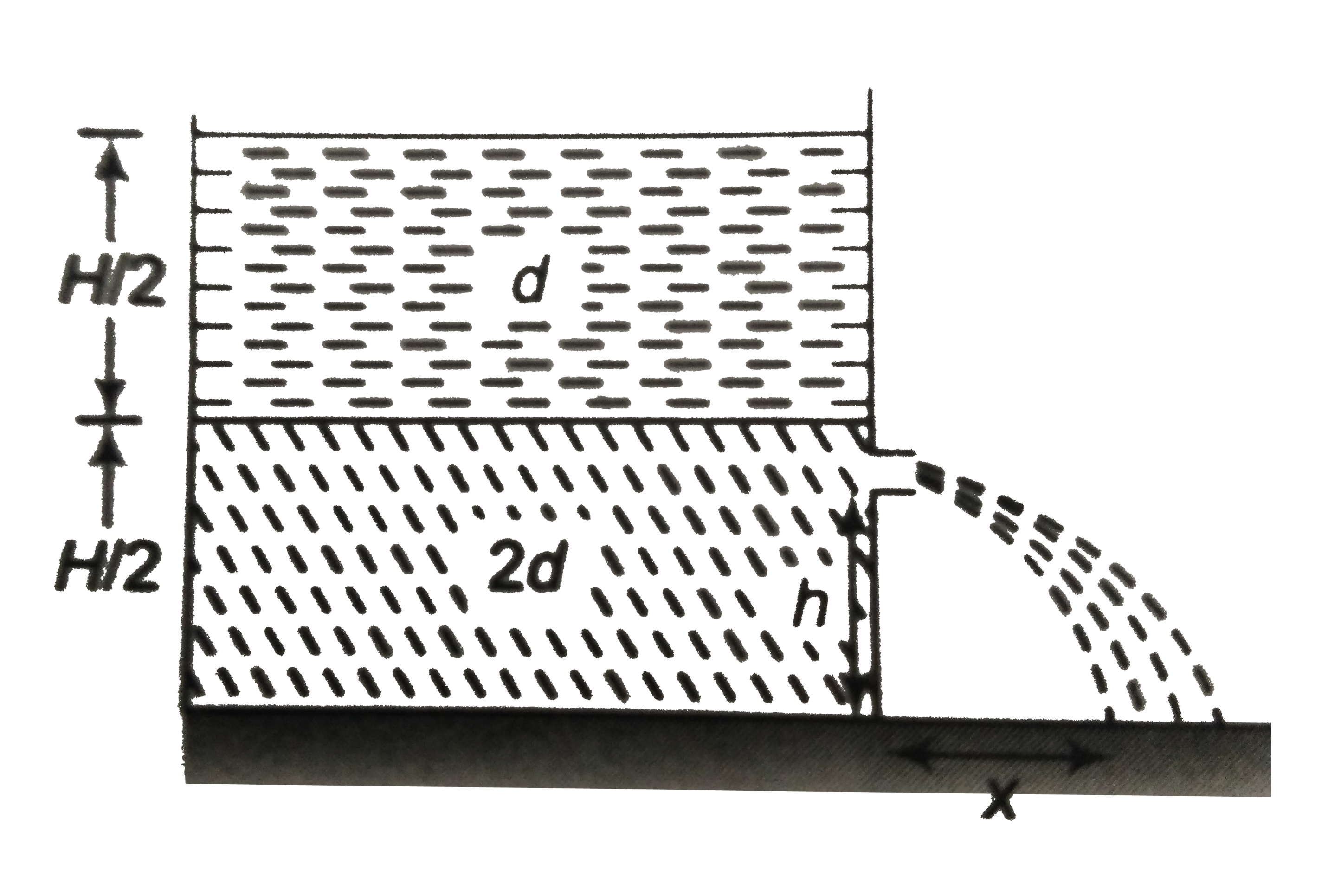
The cylinder is then removed and the original arrangement is restroed. a tiny hole of area s(s<<A) is punched on the vertical side of the container at a height h(h<H/2). As a result of this, liquid starts flowing out of the hole with a range x on the horizontal surface.
The total pressure with cylinder, at the bottom of the container is04:08View SolutionA hydraulic automobile lift is designed to lift cars with a maximum mass of 3000Kg. The area of cross section of the piston carrying the load is 425cm2. What maximum pressures would the smaller piston have to bear?
05:12View SolutionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
10:40View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
10:40View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01xx 10^(5)N//m^(2),g=9.8 m//s^(2) and density of water =10^(3)kg//m^(3) .
04:03View SolutionA glass capillary tube (closed from tap) of inner diameter 0.28mm is lowered vertically into water in a vessel. The pressure in the capillary tube so that water level in the tube is same as that in the vessel is N/m2 is (surface tension of water =0.7N/m and atmospheric pressure =105N/m2.
04:20View SolutionA siphon has a uniform circular base of diameter 8//sqrt(pi) cm with its crest A, 1.8 m above the water level vessel B is of large cross section ( g= 10 m//s^(2) and atmospheric pressure P_(0) = 0^(5) N//m^(2)) .
03:42View SolutionA tube of uniform cross section is used to siphon water from a vessel V as shown in the figure. The pressure over the open end of water in the vessel is atmospheric pressure (P0). The height of the tube above and below the water level in the vessel are h1 and h2, respectively.
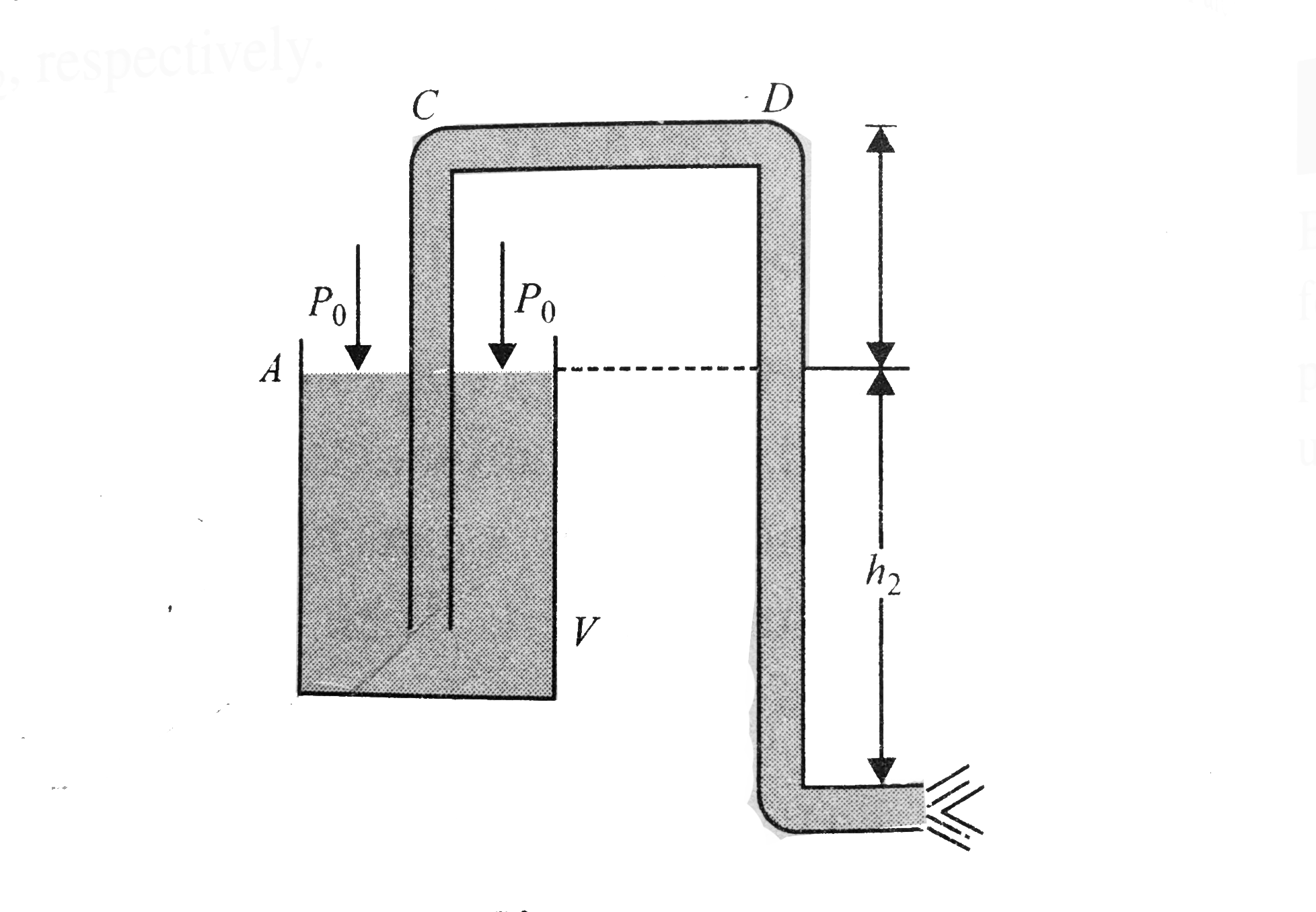
If h1=h2=3.0m, the maximum value of h1, for which the siphon will work will be03:58View SolutionA tube of uniform cross section is used to siphon water from a vessel V as shown in the figure. The pressure over the open end of water in the vessel is atmospheric pressure (P0). The height of the tube above and below the water level in the vessel are h1 and h2, respectively.
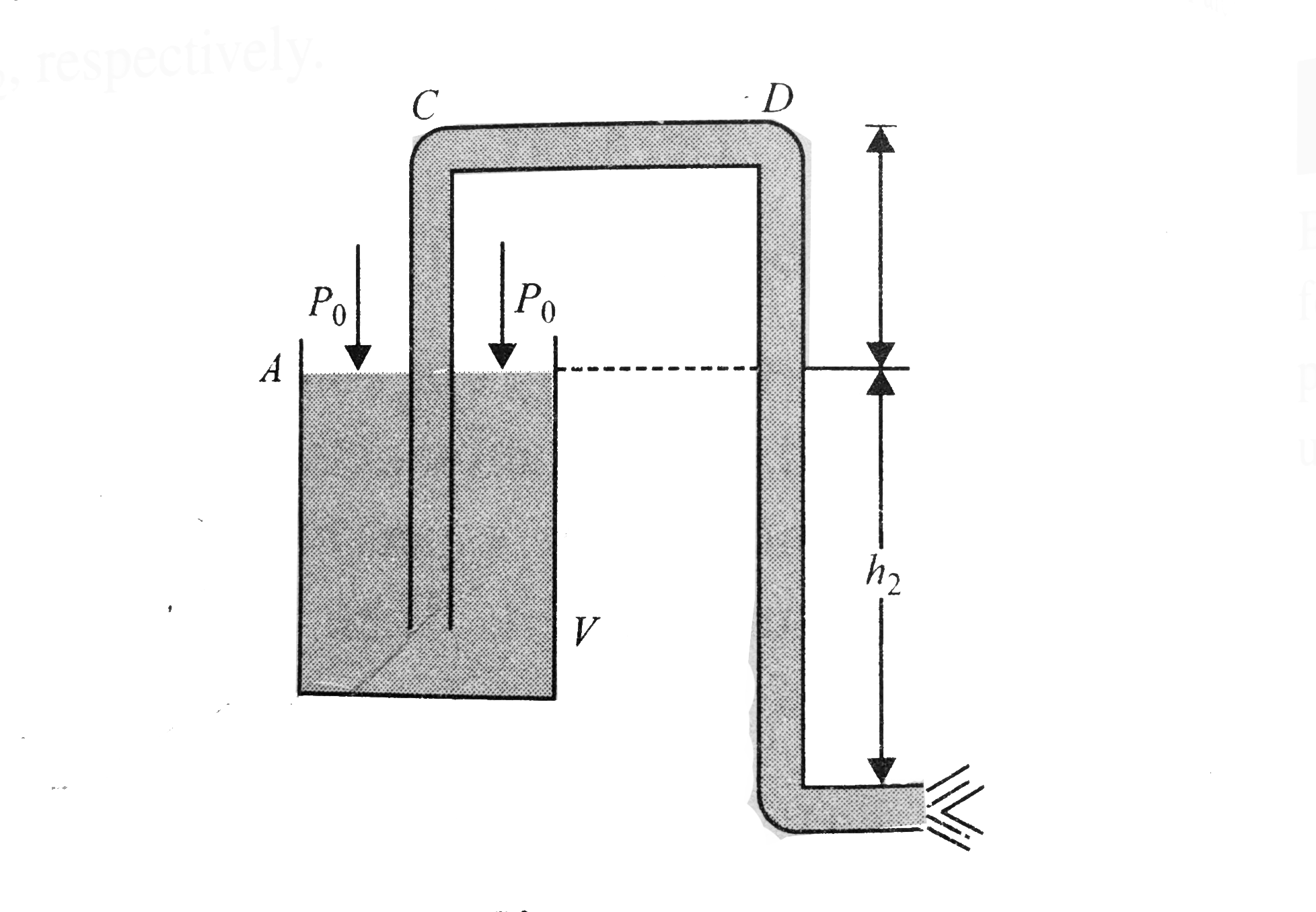
Given h1=h2=3.0m, the gauge pressure of water in the highest level CD of the tube will be02:34View SolutionA glass capillary tube of inner diameter 0.28 mm is lowered vertically into water in a vessel. The pressure to be applied on the water in the capillary tube so that water level in the tube is same as the vessel in Nm2 is (surface tension of water =0.07Nm atmospheric pressure =105Nm2
05:57View SolutionA tube of small uniform cross section is used to siphon the water from the vessel. Then choose the correct alternative (s) :
(ρwater=103kg/m3,g=10m/s2,Patm=105Pa)
 05:04View Solution
05:04View SolutionTen litre of water is compressed by an increase in pressure of 10 atmosphere. If the compressibility of water is 5×10−10m2/N, then the change in volume of water will be (one atmosphere =105N/m2).
04:32View SolutionThe cross-sectional area of water pipe entering the basement is 4 x 10−4m2. The pressure at this point is 3 x 105Nm−2 and the speed of water is 2 ms−1. This pipe tapers to a cross-sectional area of 2 x 10−4m2 when it reaches the second floor 8 m above. Calculate the speed and pressure at the 2nd floor
03:31View SolutionA capillary tube of diameter 0.20 mm is lowered vertically into water in a vessel. What should be the pressure in N//m^(2) to be applied on the water in the capillary tube so that water level in the tube is same as that in the vessel ? (surface tension of water is 0.07 N/m and atm pressure is 10^(5)N//m^(2))
01:31View SolutionTen litre of water is compressed by an increase in pressure of 10 atmosphere. If the compressibility of water is 5xx10^(-10)m^(2)//N, then the change in volume of water will be (one atmosphere =10^(5)N//m^(2)).
09:47View Solution

