Minimum thickness of a mica sheet having μ=32 which should be placed in front of one of the slits in YDSE is required to reduce the intensity at the centre of screen to half of maximum intensity is Solution in Gujrati
λ/4
λ/8
λ/2
λ/3
The correct Answer is:C
Let t be the thickness so corresponding
Δx=μt=32t
also Imax=4I
so I'=2I
We know IR=I1+I2+2√I1I2cosϕ
⇒2I=I+I+2√I2cos(3πtλ)⇒cos(3πtλ)=0
⇒cos(3πtλ)=cos(π2)
or cos(3π2) or 5π2
⇒3πtλ=π2⇒t=λ6,3πtλ=3π2j⇒t=λ2
and 3πtλ=5π2⇒t=5λ6
Topper's Solved these Questions
- WAVE OPTICSBOOK - ALLEN CHAPTER - WAVE OPTICSEXERCISE - Exercise 2 (Brain Teasures)
22 Videos
- WAVE OPTICSBOOK - ALLEN CHAPTER - WAVE OPTICSEXERCISE - Exercise 3 (Miscellaneous type Questions)
27 Videos
- WAVE OPTICSBOOK - ALLEN CHAPTER - WAVE OPTICSEXERCISE - Example 15
1 Videos
- UNIT & DIMENSIONS, BASIC MATHS AND VECTOR BOOK - ALLEN CHAPTER - UNIT & DIMENSIONS, BASIC MATHS AND VECTOR EXERCISE - Exercise (J-A)
7 Videos
Similar Questions
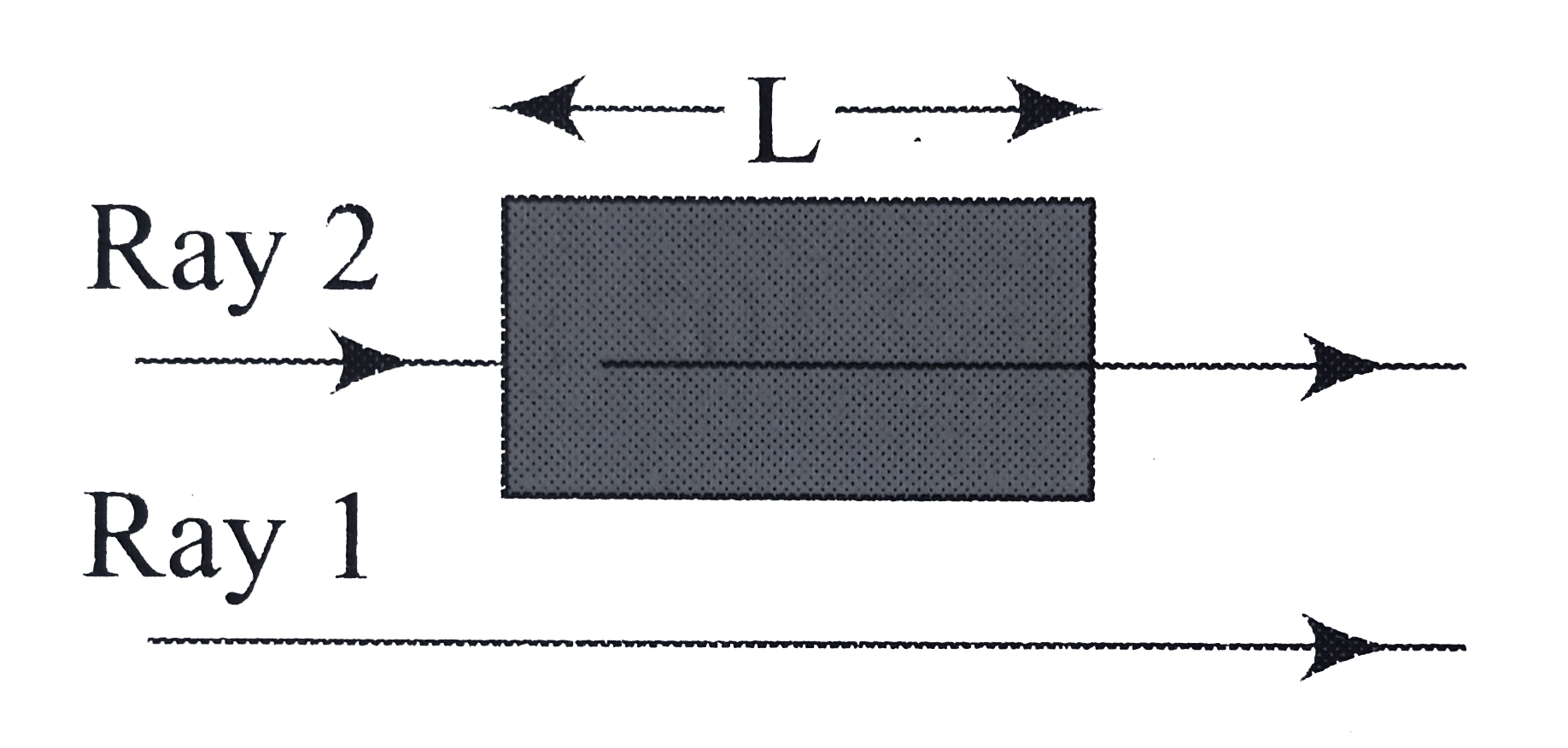
As shown in figure waves with identical wavelengths and amplitudes and which are intially in phase travel through difference media. Ray 1 travels through air and Ray 2 through a transparent medium for equal length L, in four different situations. Rays reach a common point on the screen. The number of wavelengths in length L is N2 for Ray 2 and N1 for Ray 1. In the following table, values of N1 and N2 are given for all four situations. The order or the situations according to the intensity of the light at the common point in descending order is Situations
1 2 3 4
N1 2.75 1.80 3.00 3.25
N2 2.75 2.80 3.25 4.00
View SolutionStatement-1: if white light is used in YDSE, then the central bright fringe will be white.
Statement-2: In case of white light used in YDSE all the wavelengths produce their zero order maxima at the same position.
View Solution_S01_033_Q01.png)
In a typical young's double slit experiment a point source of monochromatic light is kept as shown in the figure. If the source is given an instantaneous velocity v=1 mm per second towards the screen, then the instantaneous velocity of central maxima is given as α×10−βcm/s upward in scientific notation find the value of α+β
View SolutionMatch List I with List II and select the correct answer using the codes given below the lists :

View SolutionIn YDSE ratio of width of slit is 4:1, then ratio of maximum to minimum intensity
View SolutionIn YDSE, both slits produce equal intensities on the screen. A 100% transparent thin film is placed in front of one of the slits. Now, the intensity on the centre becomes 75% of the previous intensity. The wavelength of light is 6000Å and refractive index of glass is 1.5. Thus, minimum thickness of the glass slab is
View SolutionIn a YDSE with two identical slits, when the upper slit is covered with a thin, perfectly tranparent sheet of mica, the intensity at the centre of screen reduces ro 75% of the initial value second minima is observed to the above this point and third maxima below it which of the following can not be a possible value of phase difference caused by the mica sheet
View SolutionMinimum thickness of a mica sheet having μ=32 which should be placed in front of one of the slits in YDSE is required to reduce the intensity at the centre of screen to half of maximum intensity is
View SolutionA thin glass plate of thickness t and refractive index μ is inserted between screen and one of the slits in a young's experiment. If the intensity at the centre of the screen is I, what was the intensity at the same point prior to the introduction of the sheet.
View SolutionA plate of thickness t made of material of refractive index μ is placed in front of one of the slits in a double slit experiment . What should be the minimum thickness t which will make the intensity at the centre of the fringe pattern zero ?
View SolutionIn the above problem, minimum value of t for which the intensity at point P on the screen exactly in front of the upper slit becomes maximum.
View SolutionIn YDSE ratio of width of slit is 4:1 , then ratio of maximum to minimum intensity
View SolutionIn YDSE ratio of width of slit is 4:1, then ratio of maximum to minimum intensity
View SolutionA plate of thickness t made of a material of refractive index mu is placed in front of one of the slits in a double slit experiment. What should be the manimum thickness t which will make the intensity at the centre of the fringe pattern zero?
View SolutionIn a YDSE experiment if a slab whose refractive index can be varied is placed in front of one of the slits then the variation of resultant intensity at mid - point of screen with μ will be best represented by [Assume slits of equal width and there is no absortion by slab ]
View Solution
