In a YDSE with two identical slits, when the upper slit is covered with a thin, perfectly tranparent sheet of mica, the intensity at the centre of screen reduces ro 75% of the initial value. The second minima is observed to above this point and third maxima below it. which of the following can not be a possible value of phase difference caused by the mica sheet
π3
7π2
10π3
11π3
The correct Answer is:A
To solve the problem, we need to analyze the situation described in the Young's Double Slit Experiment (YDSE) after a mica sheet is placed over one of the slits. The key points to consider are the intensity reduction and the conditions for minima and maxima.
1. Understanding Initial Conditions:
- Let the initial intensity at the center of the screen (without the mica sheet) be I0.
- When the mica sheet is placed, the intensity reduces to I′=0.75I0.
2. Intensity Formula:
- The intensity in a YDSE is given by:
I=4I0cos2(ϕ2)
- Here, ϕ is the phase difference introduced by the mica sheet.
3. Setting Up the Equation:
- For the new intensity after placing the mica sheet:
0.75I0=4I0cos2(ϕ2)
- Dividing both sides by I0:
0.75=4cos2(ϕ2)
- Rearranging gives:
cos2(ϕ2)=0.754=316
4. Finding Phase Difference:
- Taking the square root:
cos(ϕ2)=√316=√34
- Therefore, the angle corresponding to this cosine value is:
ϕ2=cos−1(√34)
- This gives two possible values for ϕ2: π6 or 5π6.
5. Calculating Possible Values of ϕ:
- Thus, the possible values of ϕ are:
ϕ=π3,5π3
- Since the phase difference can be expressed in multiples of 2π, we can also consider:
ϕ=2nπ+π3orϕ=2nπ+5π3(n∈Z)
6. Identifying Minima and Maxima:
- The second minima occurs at:
ϕ=(2n−1)πfor n=2⇒ϕ=3π
- The third maxima occurs at:
ϕ=2nπfor n=3⇒ϕ=6π
7. Conclusion:
- The phase difference ϕ must lie between 3π and 6π.
- Possible values of ϕ include 11π3,13π3,17π3, etc.
- Values like π3 do not lie in this range and thus cannot be a possible value of the phase difference caused by the mica sheet.
Final Answer:
The value that cannot be a possible value of phase difference caused by the mica sheet is π3.
- Let the initial intensity at the center of the screen (without the mica sheet) be I0.
- When the mica sheet is placed, the intensity reduces to I′=0.75I0.
- The intensity in a YDSE is given by:
I=4I0cos2(ϕ2)
- Here, ϕ is the phase difference introduced by the mica sheet.
- For the new intensity after placing the mica sheet:
0.75I0=4I0cos2(ϕ2)
- Dividing both sides by I0:
0.75=4cos2(ϕ2)
- Rearranging gives:
cos2(ϕ2)=0.754=316
- Taking the square root:
cos(ϕ2)=√316=√34
- Therefore, the angle corresponding to this cosine value is:
ϕ2=cos−1(√34)
- This gives two possible values for ϕ2: π6 or 5π6.
- Thus, the possible values of ϕ are:
ϕ=π3,5π3
- Since the phase difference can be expressed in multiples of 2π, we can also consider:
ϕ=2nπ+π3orϕ=2nπ+5π3(n∈Z)
- The second minima occurs at:
ϕ=(2n−1)πfor n=2⇒ϕ=3π
- The third maxima occurs at:
ϕ=2nπfor n=3⇒ϕ=6π
- The phase difference ϕ must lie between 3π and 6π.
- Possible values of ϕ include 11π3,13π3,17π3, etc.
- Values like π3 do not lie in this range and thus cannot be a possible value of the phase difference caused by the mica sheet.
The value that cannot be a possible value of phase difference caused by the mica sheet is π3.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced Only one option is correct22videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - For JEE Advanced B. More than one option is correct4videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE OPTICSEXERCISE - medical entrances42videos
WAVE OPTICS
- BOOK - DC PANDEY ENGLISHCHAPTER - SOLVED PAPERS 2018EXERCISE - JIPMER22videos
SOLVED PAPERS 2018
Similar Questions
In YDSE using monochromatic light, the fringe pattern shifts by a certain distance on the screen when a mica sheet of refractive index 1.5 and thickness 2 microns is introduced in the path of one of the interfering waves. The mica sheet is then removed and the distance between the plane of slits and the screen is doubled. It is found that the distance between successive maxima (or minima) now is the same as the observed fringe shift upon the introduction of the mica sheet. Calculate the wavelength of the light.
View SolutionYoung's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
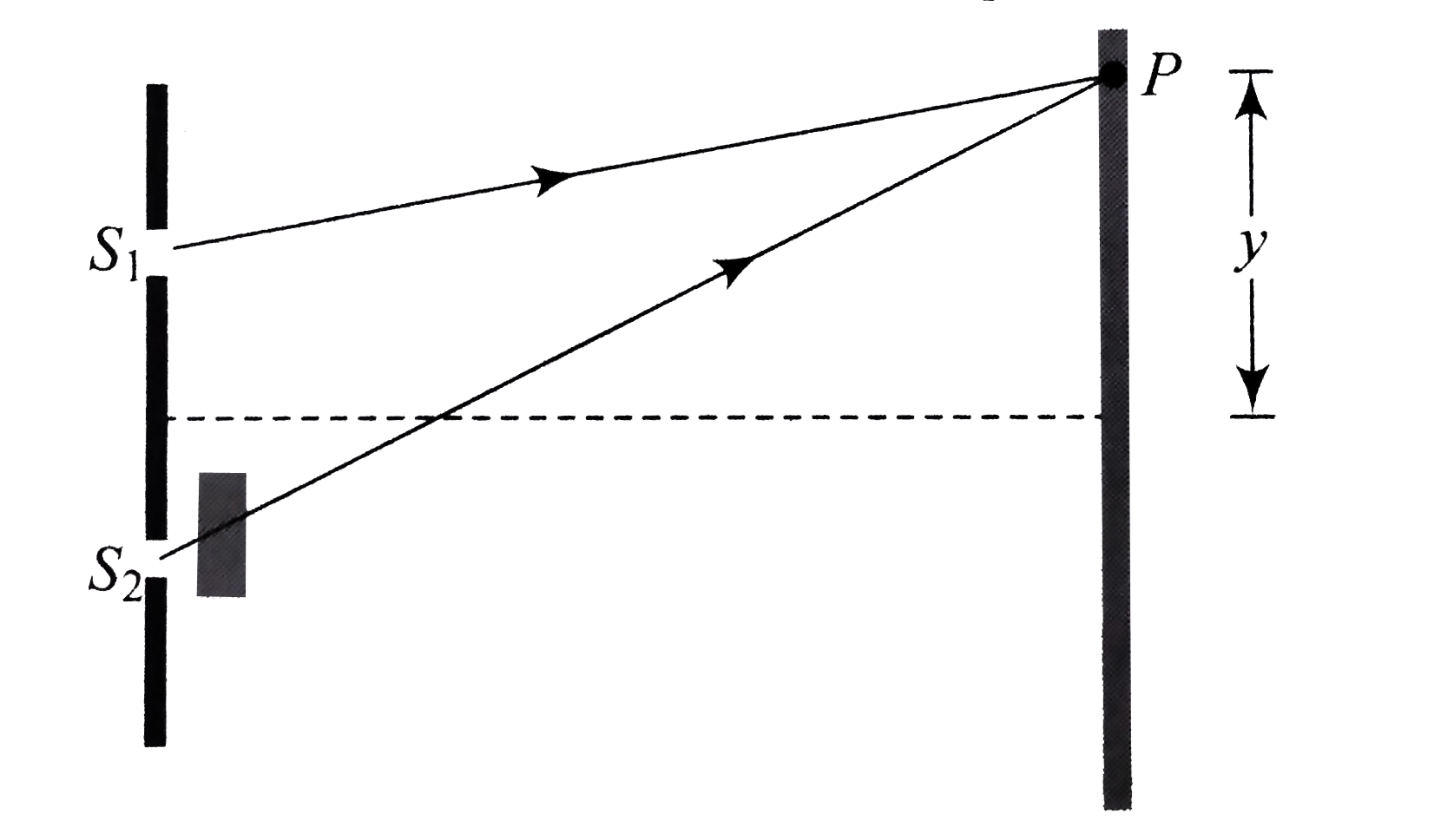
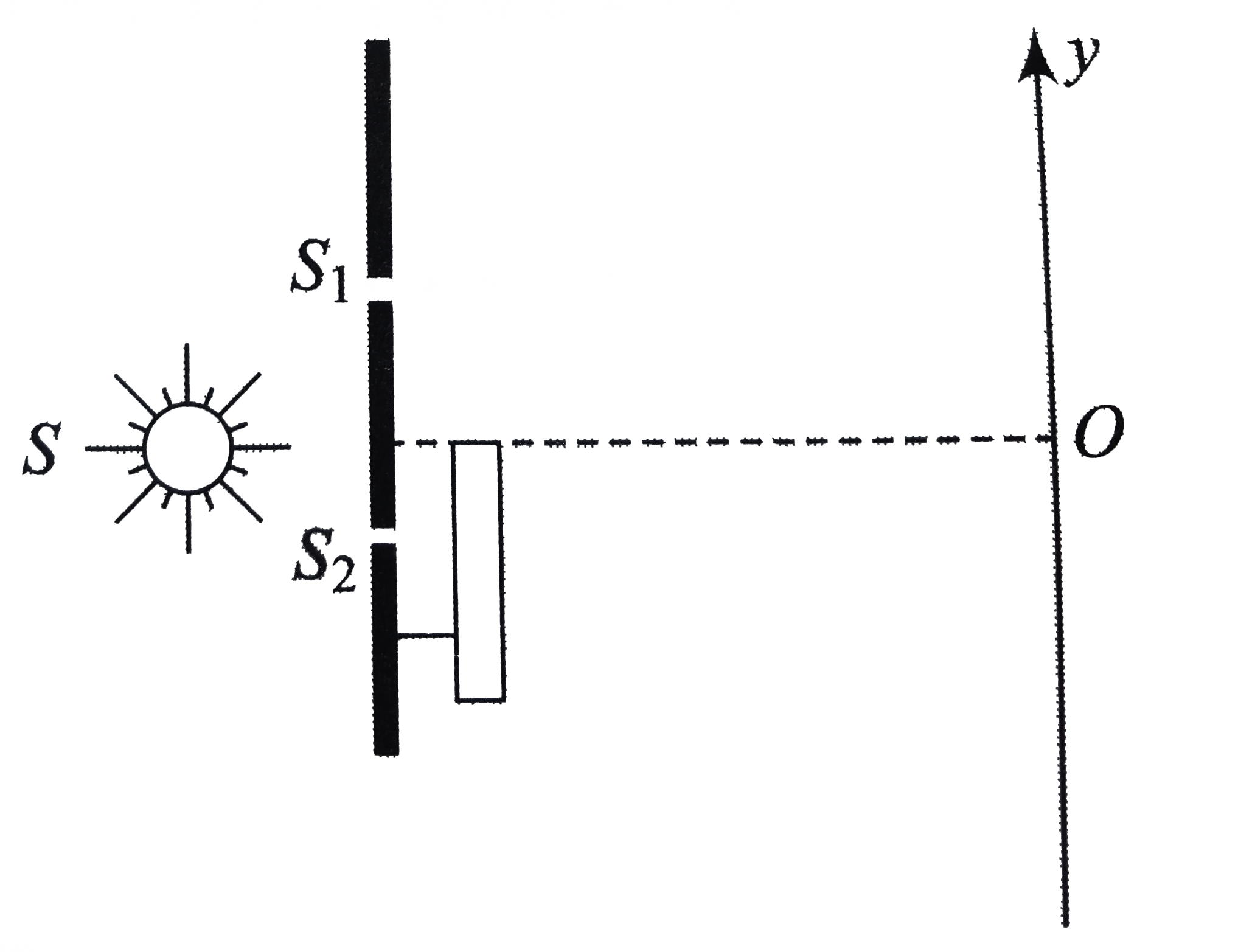
The phase difference at point P without inserting the slab isView SolutionThe Young's double-slit experiment is done in a medium of refractive index 4/3. A light of 600 nm wavelength isfalling on the slits having 0.45 mm separation. The lower shift S2 is covered by a thin glass sheet of refractive index. 1.5. The interference pattern is observed on a screen placed 1.5 m from the slits as shown in Figure
a. Find the location of central maximum (bright fringe with zero path difference) on the y-axis.
b. Find the light intensity of point O relative to the maximum fringe intensity.
c. Now , if 600 nm light is replaced by white light of range 400 - 700 nm, find the wavelengths of the light that from maxima exaclty at point O.
(All wavelength in the problem are for the given medium of refractive index 4/3 Ignoe dispersion.)
 View Solution
View SolutionMinimum thickness of a mica sheet having μ=32 which should be placed in front of one of the slits in YDSE is required to reduce the intensity at the centre of screen to half of maximum intensity is
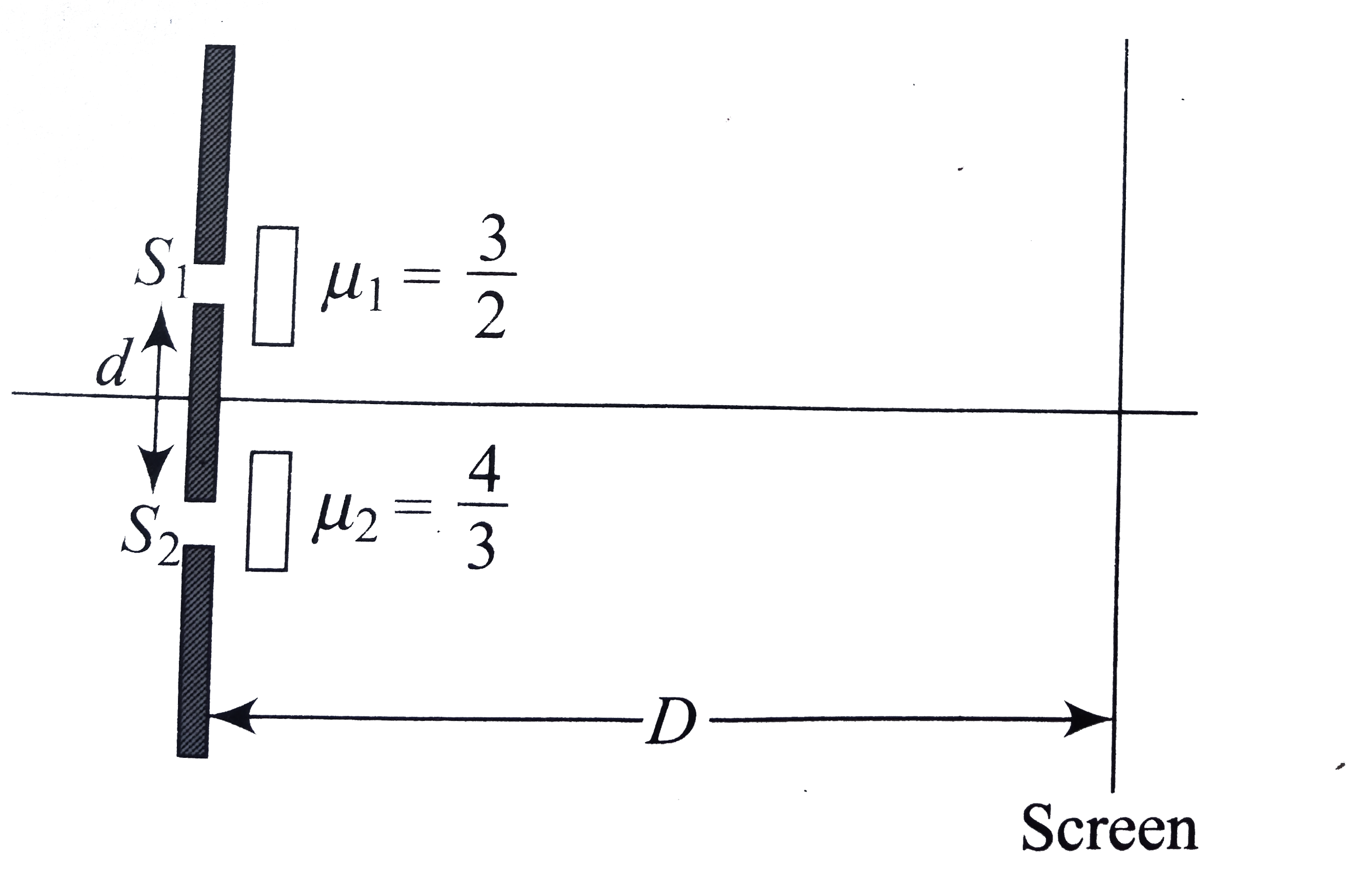
View SolutionA monochromatic beam of light of 6000 Å is used in YDSE set-up. The two slits are covered with two thin films of equal thickness t but of different refractive indices as shown in figure. Considering the intensity of the incident beam on the slits to be I0, find the point on the screen at which intensity is I0 and is just above the central maxima. (Assume that there is no change in intensity of the light after passing through the films.) Consider t=6μm,d=1mm, and D=1m, where d and D have their usual meaning. Give your answer in mm.
 View Solution
View SolutionIn a YDSE using monochromatic visible light, the distance between the plate of slits and the screen is 1.7 m. At point P on the screen which is directly in front of the upper slit, maximum path is observed. Now, the screen is moved 50 cm closer to the plane of slits. Point P now lies between third and fouth minima above the central maxima and the intensity at P in one-fourth of the maxima intensity on the screen.
Find the value of n.View SolutionIn a YDSE using monochromatic visible light, the distance between the plate of slits and the screen is 1.7 m. At point P on the screen which is directly in front of the upper slit, maximum path is observed. Now, the screen is moved 50 cm closer to the plane of slits. Point P now lies between third and fouth minima above the central maxima and the intensity at P in one-fourth of the maxima intensity on the screen. ltbr> Find the wavelength of light if the separation of slits is 2 mm.
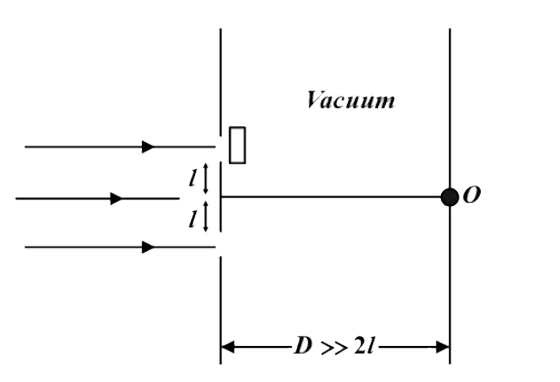
View SolutionIn young's double-slit experiment, both the slits produce equal intensities on a screen. A 100% transparent thin film of refractive index μ=1.5 is kept in front of one of the slits, due to which the intensity at the point O on the screen becomes 75% of its initial value. If the wavelength of monochromatic light is 720 nm, then what is the minimum thickness (in nm) of the film?
 View Solution
View SolutionIn YDSE, light of wavelength λ=5000Å is used, which emerges in phase from two a slits distance d=3×10−7m apart. A transparent sheet of thickness t=1.5×10(−7)m, refractive indes n=1.17, is placed over one of the slits. Where does the central maxima of the interference now appear from the center of the screen? (Find the value of y?)
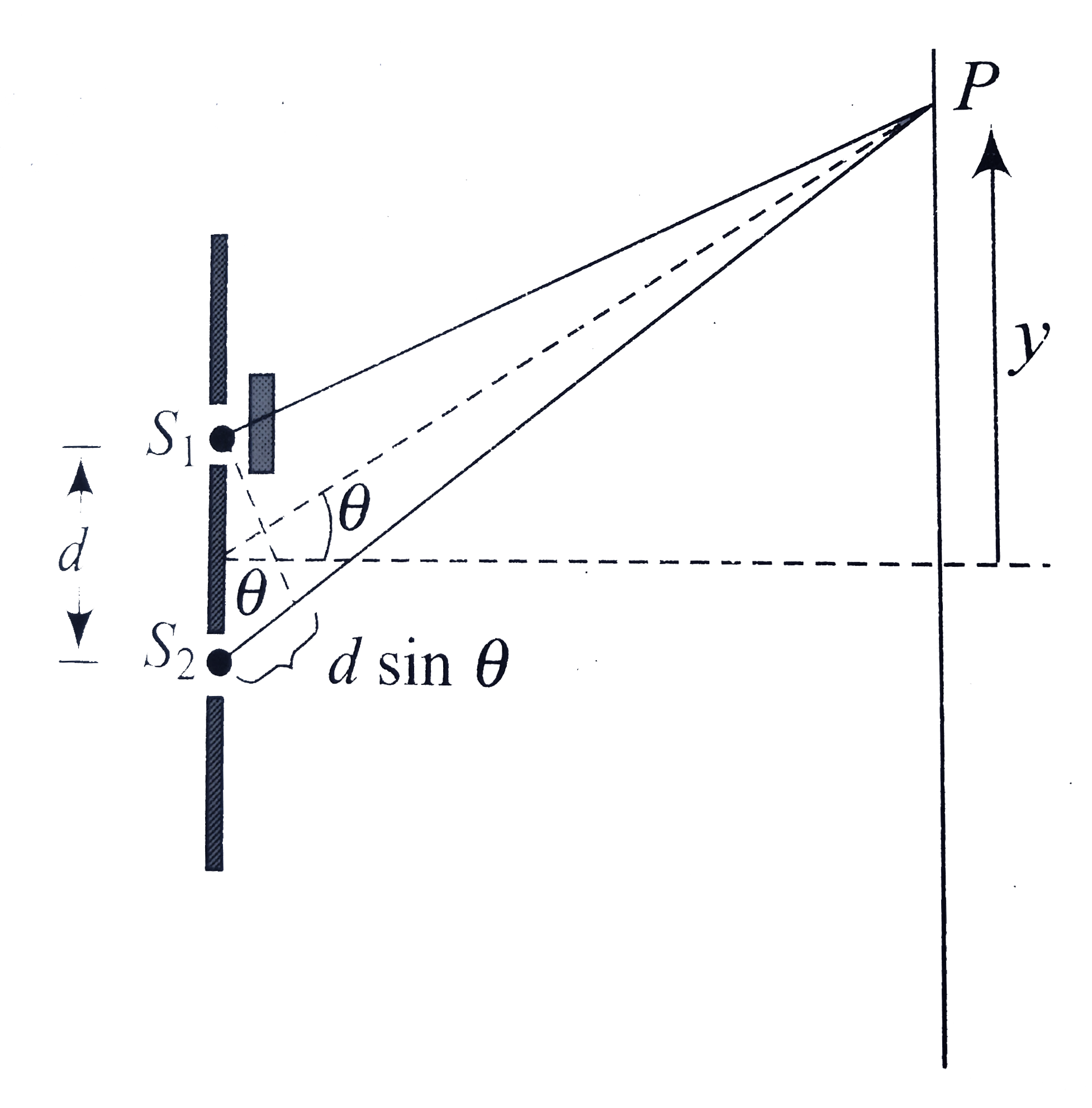 View Solution
View SolutionYoung's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
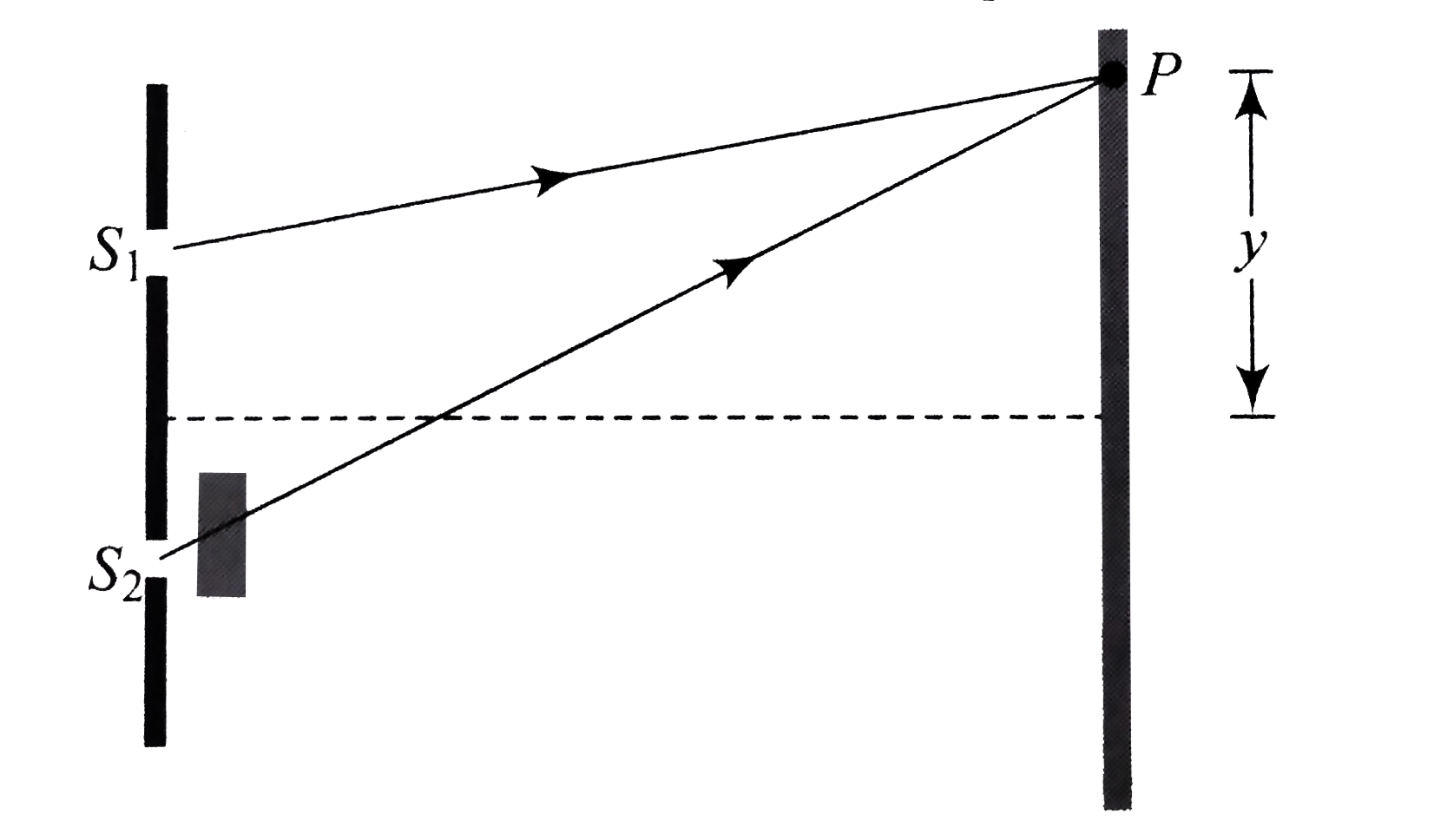
The value of η isView Solution

