Two successive resonance frequencies in an open organ pipe are 1944 Hz and 2592 Hz. Find the length of the tube. The speed of sound in air is 324ms−1.Solution in Gujrati
The correct Answer is:0.25m
Let the pipe resonates in nth&(n+1)th harmonic
⇒(n+1)1944=n(2592)
⇒n=4∴L=3241296=0.25m
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[B]
13 Videos
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-05)[A]
28 Videos
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-03)
31 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
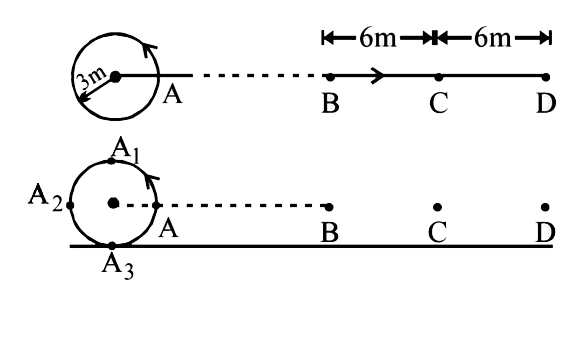
A source of sound is moving along a circular orbit of radius 3meter with an angular velocity of 10rad/s. A sound detector located far away from the source is executing linear simple harmonic motion along the line BD with an amplitude BC=CD=6meters. The frequency of oscillation of the detector is 5π per second. The source is at the point A when the detector is at the point B. If the source emits a continuous sound wave of frequency 340Hz, Find the maximum and the minimum frequencies recorded by the detector.
View SolutionTube A has both ends open while tube B has one closed, otherwise they are identical. The ratio of fundamental frequency of tube AandB is
View SolutionStatement-1 : Sound travels faster in moist air
Statement-2 : The density of moist air is less then density of dry air.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionA closed organ pipe can vibrate at a minimum frequency of 500 Hz. Find the length of the tube. Speed of sound in air =340ms−1.
View SolutionAn ideal organ pipe resonates at successive frequencies of 50Hz,150Hz,250Hz , etc. ( speed of sound =340m/s) The pipe is
View Solution(a) A cylindrical metal tube has a length of 50cm and is open at both ends. Find the frequencies between 1000Hz and 2000Hz at which the air is 340m/s.
(b) Find the greatest length of an organ pipe open at both ends that will have its fundamental frequency in the normal hearing range (20−20000Hz). Speed of sound in air =340m/s.
(c) Two successive resonance frequencies in an open organ pipe are 1944Hz and 2592Hz. Find the length of the tube. The speed of sound in air is 324m/s.
View SolutionTwo adjacent resonance frequency of an open organ pipe are 1800 and 2100Hz. Find the length of the tube. The speed of sound in air is 330m/s.
View SolutionAn open pipe of length 33 cm resonates with frequency of 100 Hz . If the speed of sound is 330 m/s , then this frequency is
View SolutionFor a certain organ pipe three successive resonance frequencies are observed at 425Hz, 595 Hz and 765Hz respectively. If the speed of sound air is 340m/s, then the length of the pipe is
View SolutionFor a certain pipe, three successive resonant frequencies are observed at 300 Hz, 420 Hz and 540 Hz.
The speed of sound in air is 340 m/s. The pipe is a
View SolutionAn open organ pipe sounds a fundamental note of frequency of 230 Hz . It the speed of sound in air is 330 m//s ., then the length of the pipe is nearly-
View SolutionFor a certain organ pipe three successive resonance frequencies are observed at 425 Hz , 595 Hz and 765 Hz respectively . If the speed of sound in air is 340m/s, then the length of pipe is ,
View SolutionThe one-way open resonant tube is resonated with a tune of 264 Hz frequency. Speed of sound 330 m*s^-1 What will be the length of the air column?
View SolutionThe two consecutive resonance frequencies of an open argon pipe are 1944 Hz and 2592 Hz. If the speed of sound in the air is 324 m / s,
View SolutionA closed organ pipe can vibrate at a minimum frequency of 500 Hz. Find the length of the tube. Speed of sound in air =340ms−1.
View Solution