A string 25cm long and having a mass of 2.5gm is under tension. A pipe closed at one end is 40cm long. When the string is set vibrating in its first overtone and the air in the pipe in its fundamental frequency, 8 beats per second are heard. It is observed that decreasing the tension in the string decreases beat frequency. If the speed of sound in air is 320m/s, find the tension in the string.Solution in Gujrati
The correct Answer is:27.04N
Fstring>Fpipe
Fstring=22L√Tμ=4√T10−2=40√T
Fpipe=vλ=3204×0.4=200Hz
⇒40√T=200=8⇒T=27.04N
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[B]
13 Videos
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-05)[A]
28 Videos
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-03)
31 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
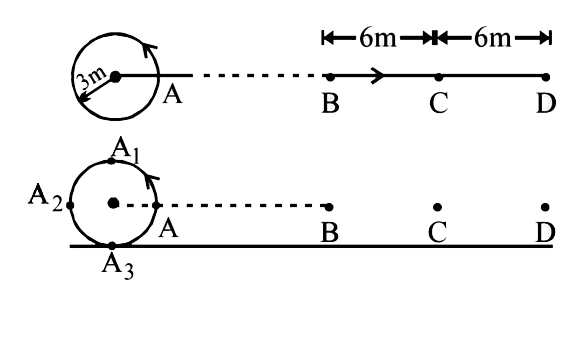
A source of sound is moving along a circular orbit of radius 3meter with an angular velocity of 10rad/s. A sound detector located far away from the source is executing linear simple harmonic motion along the line BD with an amplitude BC=CD=6meters. The frequency of oscillation of the detector is 5π per second. The source is at the point A when the detector is at the point B. If the source emits a continuous sound wave of frequency 340Hz, Find the maximum and the minimum frequencies recorded by the detector.
View SolutionTube A has both ends open while tube B has one closed, otherwise they are identical. The ratio of fundamental frequency of tube AandB is
View SolutionStatement-1 : Sound travels faster in moist air
Statement-2 : The density of moist air is less then density of dry air.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionA string 25cm long and having a mass of 2.5 gm is under tension. A pipe closed at one end is 40cm long. When the string is set vibrating in its first overtone and the air in the pipe in its fundamental frequency, 8 beats per second are heard. It is observed that decreasing the tension in the string decreases beat frequency. if the speed of sound in air is 320m//s , find the tension in the string.
View SolutionA string 1m long and having linear mass density 10g/m is sounded with a closed pipe of length 75cm. When string is vibrating in its first overtone and the air in the pipe in its fundamental frequency, 5beats/s are heard. It is observed that decreasing the tension in the string decreases the beat frequency. If the speed of sound in air is 315m/s, Find the tension in the string.
View SolutionA string of length 50 cm and mass 12.5 g is fixed at both ends. A pipe closed at one end has a length 85 cm . When the string vibrates in its second overtone and the air column in the pipe in its first. Overtone, they produce a beats frquency of 6 Hz . It is also observed that decreasing teh tension in the string decreaes beats frequency. Neglect the end correction in the pipe and velocity of sound in air is 340 m//s . then the tension in the string is
View SolutionA string 25cm long fixed at both endds and having a mass of 2.5g is under tension. A pipe closed from one end is 40cm long. When the strin is set vibrating in its first overtone and the air in the pipe in its fundamental frequency, 8 beats per second are heard. If is observed that decreasing the tension in the string decreases the beat frequency. If the speed of sound in air is 320m/s. Find tension in the string.
View Solution25 सेमी लम्बी एक डोरी जिसका द्रव्यमान 2.5 ग्राम है, तनी है। एक सिरे पर बन्द एक पाइप की लम्बाई 40 सेमी है। जब डोरी को उसके प्रथम अधिस्वरक (first overtone) में तथा पाइप की वायु को उसकी मूल आवृत्ति में कम्पित कराया जाता है, तो प्रति सेकण्ड 8 विस्पन्द सुनाई पड़ते है। यह देखा जाता है कि डोरी पर तनाव घटने पर विस्पन्द-आवृत्ति घटती है। यदि वायु में ध्वनि की चाल 320 मीटर/सेकण्ड हो, तो डोरी में तनाव ज्ञात कीजिए।
View SolutionA hollow pipe of length 0. 8 m is closed at one end. At its open end a 0 . 5 m long uniform string is vibrating in its second harmonic and it resonates with the fundamental frequency of the pipe. It the tension in the wire is 50 N and the speed of sound is 320 m* s^(-1) . the mass of the string is
View SolutionA string 25 cm long and having a mass of 2.5 g is under tension. When the string is set vibrating in its first overtone and the air in the pipe in its fundamental frequency , 8 beats per second are heard. It is observed that decreasing in the string, decreases the beat frequency . If the velocity of sound in air be 320 m*s^(-1) find the tension in the string .
View SolutionA hollow pipe of length 0.8 m is closed at one end. At its open end a 0.5 m long uniform string as vibrating in its second harmonic and it resonates with the fundamental frequency of te pipe. If the tension in the wire is 50 N and the speed of sound is 320 ms^(-1) the mass of the string is :
View SolutionA string 25 cm long and having a mass of 2.5g is under tension. A pipe closed at one end is 40 cm long. When the string is set vibrating in its firsy overtone and the air in the pipe in its fundamental frequency, 8 beats per sec are heard. It is observed that decreasing the tension in the string decreases the beat frequency. If the speed of sound in air is 320 m/s, find the tension in the string.
View SolutionA string 25 cm long and having a mass of 2.5g is under tension. A pipe closed at one end is 40 cm long. When the string is set vibrating in its firsy overtone and the air in the pipe in its fundamental frequency, 8 beats per sec are heard. It is observed that decreasing the tension in the string decreases the beat frequency. If the speed of sound in air is 320 m/s, find the tension in the string.
View SolutionA string 25 cm long and having a mass of 2.5 g is under tension. A pipe closed at one end is 40 cm long. When the string is set vibrating in its first overtone and the air in the pipe in its fundamental frequency, 8 beats per second are heard. It is observed that decreasing the tension in the string decreases the beat frequency. If the speed of sound in air is 320 m/s, find the tension in the string
View SolutionA closed organ pipe is sounded near a guitar, causing one of the string to vibrate with large amplitude . We vary the tension of the string until we find the maximum amplitude. The string is 80% as long as the closed pipe. If both the pipe and the string vibrate at their fundamental frequency, calculate the ratio of the wave speed on the string to the speed of sound in air.
View Solution
