The displacement y of a wave travelling in the x-direction is given by
y=10−4sin((600t−2x+π3)meters
where x is expressed in meters and t in seconds. The speed of the wave-motion, in ms−1, is Solution in Gujrati
300
600
1200
200
The correct Answer is:A
y=104sin(600t−2x+π3)
On comparing the given equation with general equation of wave, we get
y=y0sin(ωt−kx+ϕ)
ω=600,k=2
Wave speed =ω2=6002=300m/s.
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-05)[B]
42 Videos
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[B]
13 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
A string of length 0.4m and mass 10−2kg is clamped at one end . The tension in the string is 1.6N. The identical wave pulses are generated at the free end after regular interval of time , Δt . The minimum value of Δt , so that a constructive interference takes place between successive pulses is
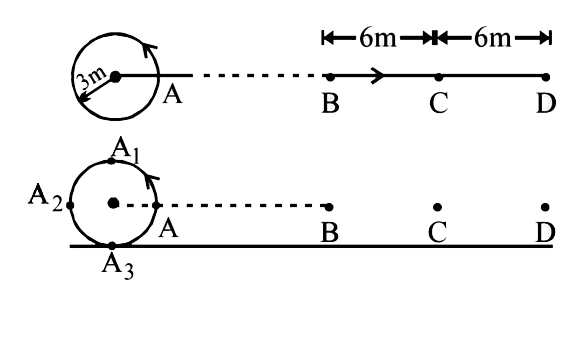
View SolutionA source of sound is moving along a circular orbit of radius 3meter with an angular velocity of 10rad/s. A sound detector located far away from the source is executing linear simple harmonic motion along the line BD with an amplitude BC=CD=6meters. The frequency of oscillation of the detector is 5π per second. The source is at the point A when the detector is at the point B. If the source emits a continuous sound wave of frequency 340Hz, Find the maximum and the minimum frequencies recorded by the detector.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionThe displacement y of a wave travelling in the x-direction is given by
y=1004sin((600t−2x+π3)meters
where x is expressed in meters and t in seconds. The speed of the wave-motion, in ms−1, is
View SolutionThe displacement y of a wave travelling in the x-direction is given by y = 10^(-4) sin (600t - 2x + (pi)/(3)) m Where x is expressed in metre and t in seconds. The speed of the wave motion in m/s is
View SolutionThe displacement y of a wave travelling in the x direction is given by y=(2×10−3)sin(300t−2x+π4), where x and y are measured in metres and t in second. The speed of the wave is
View SolutionThe displacement y of a wave travelling in the x direction is given by y=(2×10−3)sin(600t−2x+π3), where x and y are measured in metres and t in second. The speed of the wave is
View Solutionx दिशा में चलने वाली तरंग का विस्थापन समीकरण निम्न है -
y=10−4sin(600t−2x+π2)मीटर
जहाँ x मीटर में तथा t सेकंड में है। तरंग की चाल मी/से में होगी -
View SolutionThe displacement y of a wave travelling in the x direction is given by y=(2×10−3)sin(300t−2x+π4), where x and y are measured in metres and t in second. The speed of the wave is
View SolutionThe displacement y of a wave travelling in the x direction is given by y=(2×10−3)sin(300t−2x+π4), where x and y are measured in metres and t in second. The speed of the wave is
View SolutionThe displacement y of a wave travelling in the x direction is given by y=(2×10−3)sin(300t−2x+π4), where x and y are measured in metres and t in second. The speed of the wave is
View SolutionThe displacement y of a wave travelling in the x- direaction is given by y=10−4sin(600t−2x+π/3)
Where x is expreseend in metres and t is seconds. What is the speed of the wave motion (in ms−1) ?
View SolutionThe displacement y of a wave travelling in the x direction is given by y=(2×10−3)sin(300t−2x+π4), where x and y are measured in metres and t in second. The speed of the wave is
View Solutionx திசையில் இயங்கிக் கொண்டுள்ள அலை ஒன்றின் இடப்பெயர்ச்சி y இதற்கான சமன்பாடு y = (2 * 10−3) sin (300t - 2x + (π4)), இங்கு x, y மீட்டரிலும் t வினாடியிலும் அளக்கப்பப்பால், அலையின் வேகம்
View SolutionThe equation for the displacement of a wave on the x axis of y is y = 10^(-4) sin (600t - 2x + pi/3) x is denoted in meters and t in seconds. The speed of oscillation
View SolutionTravel in the direction of x The displacement of a wave y = 10^(-4) sin (600t - (2x + pi/3)) If x is in meters and t is in seconds then the speed of wave motion
View Solution
