A tuning fork of known frequency 256Hz makes 5 beats per second with the vibrating string of a piano. The beat frequency decreases to 2 beats per second when the tension in the piano string is slightly increased. The frequency of the piano string before increasing the tension wasSolution in Gujrati
(256+2)Hz
(256−2)Hz
(256−5)Hz
(256+5)Hz
The correct Answer is:C
To frequency of the vibrating string with respect to tuning fork is either (256+5)Hzor(256−5)Hz
(256+5)Hzand(256−5)Hz
fwire=12l√Tμ
On increasing tension , the beat frequency decreases to 2 Hz, so probable frequency of the wire with respect to fork none is
But on increasing the tension of wire, the frequency of the wire must have to increase. So, if the original frequency of the wire is assumed to be 261 then it readuces to 258 whereas if it is assumed to be 251 it has increased to 254. As we were expecting increase, so the correct frequency of the piano wire is (256 -5)Hz.
_E01_151_S01.png)
Topper's Solved these Questions
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-05)[B]
42 Videos
- WAVES AND OSCILLATIONSBOOK - ALLEN CHAPTER - WAVES AND OSCILLATIONSEXERCISE - Part-1(Exercise-04)[B]
13 Videos
- SEMICONDUCTORSBOOK - ALLEN CHAPTER - SEMICONDUCTORSEXERCISE - Part-3(Exercise-4)
50 Videos
Similar Questions
A string of length 0.4m and mass 10−2kg is clamped at one end . The tension in the string is 1.6N. The identical wave pulses are generated at the free end after regular interval of time , Δt . The minimum value of Δt , so that a constructive interference takes place between successive pulses is
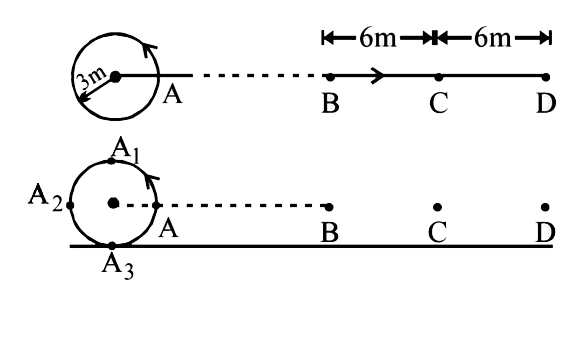
View SolutionA source of sound is moving along a circular orbit of radius 3meter with an angular velocity of 10rad/s. A sound detector located far away from the source is executing linear simple harmonic motion along the line BD with an amplitude BC=CD=6meters. The frequency of oscillation of the detector is 5π per second. The source is at the point A when the detector is at the point B. If the source emits a continuous sound wave of frequency 340Hz, Find the maximum and the minimum frequencies recorded by the detector.
View SolutionAt absolute zero temperature Si acts as ……..
View SolutionA tuning fork of known frequency 256Hz makes 5 beats per second with the vibrating string of a piano. The beat frequency decreases to 2 beats per second when the tension in the piano string is slightly increased. The frequency of the piano string before increasing the tension was
View SolutionA tuning fork of frequency 512 Hz makes 4 beats//s with the vibrating string of a piano. The beat frequency decreases to 2 beats//s when the tension in the piano string is slightly increased.The frequency of the piano string before increasing the tension was
View SolutionA tuning fork of frequency 256 Hz gives 5 beats // s with a vibrating string of a piano. The beat frequency decreases to 2, when tension in the string is slightly increased. What was the frequency of the piano string before increasing the tension ?
View SolutionA tuning fork of known frequency 256 Hz makes 5 beats per second with the vibreting string of a piano.The beat frequency decreases to 2 beats per second when the tension in the piano string is slightly increased.The frequency of the piano string before increasing the tension was
View SolutionA tuning fork of frequency 512 Hz makes 4 beats//s with the vibrating string of a piano. The beat frequency decreases to 2 beats//s when the tension in the piano string is slightly increased.The frequency of the piano string before increasing the tension was
View SolutionA tuning fork of known frequency 256 Hz makes 5 beats per second with the vibrating string of a piano . The beat frequency decreases to 2 beats/s when the tension in the piano string is slightly increased. The frequency of the piano string before increasing the tension was :
View SolutionA tuning fork of frequency 256 Hz is producing 5 beats/sec with the vibrating string of a sitar. On increasing the tension in the string, the beat frequency becomes 3 beats/sec. The initial frequency of sitar before increasing the tension is
View SolutionA tuning fork of frequency 512 Hz makes 4 beats per second with the vibrating string of a piano. The beat frequency decreases to 2 beats per sec. when the tension in the piano string is slightly increased. The frequency of the piano string before increasing the tension was
View SolutionA tuning fork of known frequency 256 Hz makes 5 beats per second with the vibrating string of a piano.The beat frequency decreases to 2 beats per second when the tension in the piano string is slightly increased.The frequency of the piano string before increasing the tension was
View SolutionA tuning fork of frequency 512 Hz makes 4 beats//s with the vibrating string of a piano. The beat frequency decreases to 2 beats//s when the tension in the piano string is slightly increased.The frequency of the piano string before increasing the tension was
View SolutionA tuning fork of frequency 510 Hz is vibrated with a sonometer wire and 4 beats per second are heard. The beat frequency increases if the tension in the string is slightly increased. The original frequency of the string is
View SolutionA piano note is compared to a tuning fork vibrating at 440 Hz. Three beats per second are listened by the piano tuner. When the tension in the string is increased slightly, the beat frequency increases. What was the initial frequency of the piano wire ?
View Solution
