The equation of state of a real gas is given by
where P, V and T are pressure, volume and temperature resperature and R is the universal gas constant. The dimensions of the constant a in the above equation isSolution in Gujrati
The correct Answer is:
Topper's Solved these Questions
- MISCELLANEOUSBook:ALLEN Chapter:MISCELLANEOUSExercise:Exercise-02
Explore 77 Videos
- MISCELLANEOUSBook:ALLEN Chapter:MISCELLANEOUSExercise:Exercise-03
Explore 1 Video
- MISCELLANEOUSBook:ALLEN Chapter:MISCELLANEOUSExercise:EXERCISE-5(A)
Explore 15 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Book:ALLEN Chapter:KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)Exercise:BEGINNER S BOX-7
Explore 8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Book:ALLEN Chapter:PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT Exercise:EXERCISE-IV
Explore 7 Videos
Similar Questions
A ladder 5m long is leaning against a wall. The foot of the ladder is pulled out along the ground away from from the wall at a rate of 2m/s. How fast is the height of ladder on the decreasing at the instant when the foot of the ladder is 4m away from the wall?
View SolutionTwo parallel metallic plates have surface charge densities σ1 and σ2 as shown in figure.
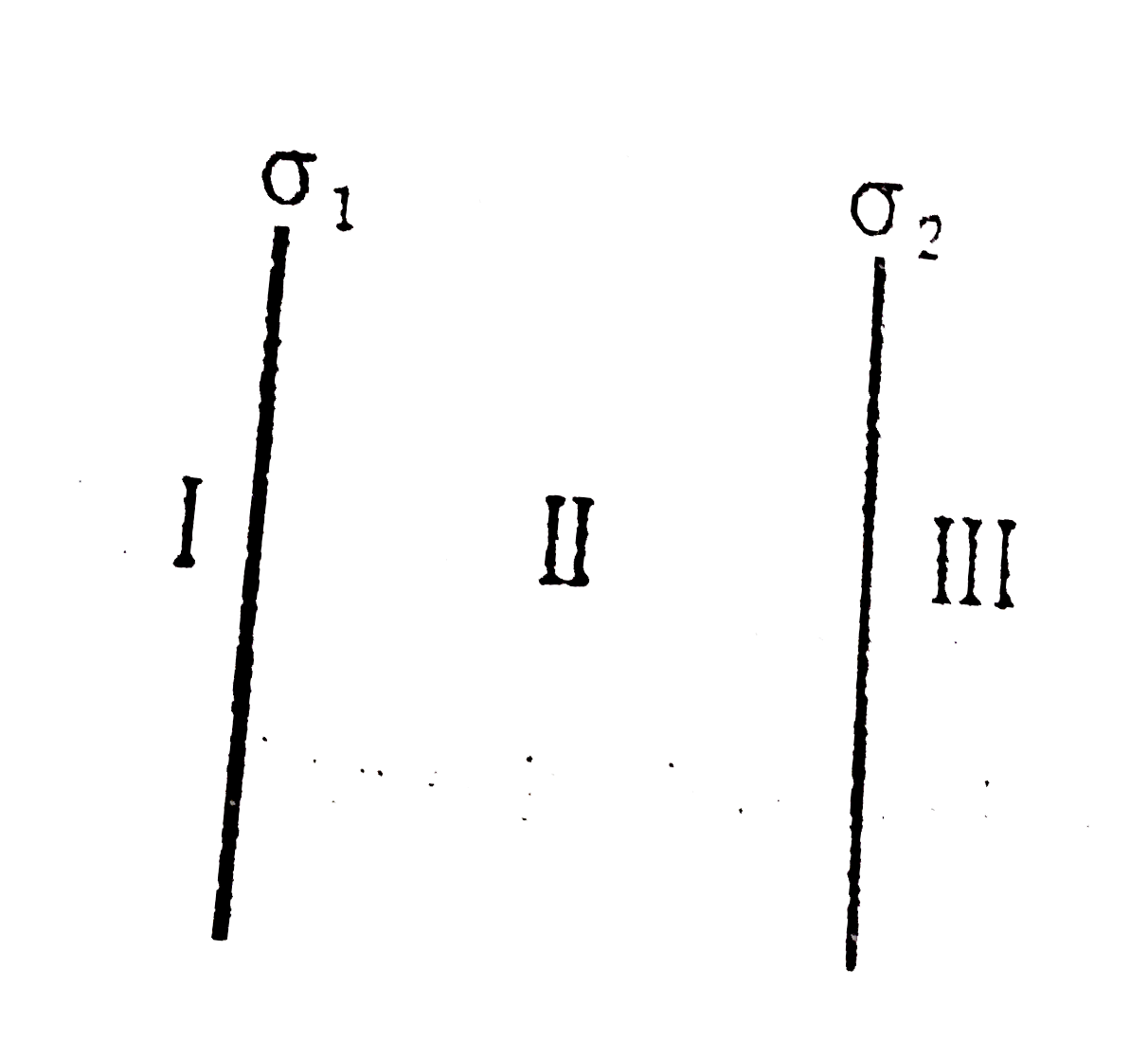
Column-IColumn-II(A)If σ1+σ2=0(p)Electric field in region III is towards right(B)Ifσ1+σ2>0(q)Electric field in region I is zero(C)If σ1+σ2<0(r)Electric field in region I is towards roght(s)Nothing can be said
View SolutionIdentify the pair whose dimensions are equal
View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
View SolutionAssertion : Gravitational forces do not depend on the medium between the masses.
Reason : Mediating particle responsible for gravitational force is graviton.
View SolutionThe equation of state for real gas is given by ((p+aV2(V−b)=RT. The dimension of the constant a is ………………. .
View SolutionFind out the unit and dimensions of the constants aandb in the van der Waal's equation (P+aV2)(V−b)=Rt, where P is pressure , v is volume , R is gas constant , and T is temperature.
View SolutionIn Vander Wall's equation (P +(a)/(V^2))(V - b) = RT What are the dimensions of a and b ? Here, P is pressure, V is volume, T is temperature and R is gas constant.
View SolutionThe equation of a state of a real gas is given by (P+aV2)(V−b)=RT, where T is absolute temperature, P is pressure, V is volume and R is universal gas constant. What are the dimensions of constant a and b ?
View SolutionThe equation of state for a real gas having pressure P, volume V and temperature T is expressed as (P+(a)/(V^(2)))(V-b)=RT where a, b and R are constant. What are the dimensional formula of (a)/(b) ?
View Solutionवाण्डर वाल का वास्तविक गैसों के लिए समीकरण है : (P+aV2)(V−b)=RT जहाँ P = दाब, V = आयतन, T = ताप, R = सार्वत्रिक गैस नियतांक तथा a और b नियतांक है नियतांक a का विमीय सूत्र है :
View Solutionकिसी गैस के दाब P, आयतन V तथा ताप T में वाण्डरवाल समीकरण के अनुसार निम्नलिखित संबंध होता है -
(P+aV2)(V−b)=RT
जहाँ a तथा b नियतांक है| इनकी विमाएँ ज्ञात कीजिए |
View SolutionThe equation of state for a real gas is given by (P+(a)/(v^(2)))(v-b)=RT the dimensions of constant a are
View Solutionवास्तविक गैस के लिए वांडर वाल्स समीकरण
(p+aV2)(V−b)=RT है,
जहाँ p, V तथा T क्रमशः दाब, आयतन तथा ताप है और R सार्वत्रिक गैस नियतांक है। उपरोक्त समीकरण में a की विमाएँ……………..है।
View SolutionThe equation of state of a gas is given by (p+aV3)(V−b2)=cT where p, V and T are pressure volume and temperature respectively and a, b,c are constants. The dimensions of a and b are respectively
View SolutionFor real gases van der Waals equation of state can be expressed as (p+aV2)(V−b)=RT
where p is the pressure V is the molar volume and T is the absolute temperature of the given sample of gas and a,b, and R are constants.
Which of the following does not have the same dimension as that of RT?
View Solution