If E is the electric field intensity ε0 is the permitivity of free space, then find dimensions of quantity ε0E2. Solution in Gujrati
[MLT−1]
[ML2T−2]
[ML−1T−2]
[ML2T−1]
The correct Answer is:C
12∈0E2−[M−1L−3T4I2][M2L2T−6I−2]=[M1L−1T−2]
Topper's Solved these Questions
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Exercise-02
77 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - Exercise-03
1 Videos
- MISCELLANEOUSBOOK - ALLEN CHAPTER - MISCELLANEOUSEXERCISE - EXERCISE-5(A)
15 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
- PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT BOOK - ALLEN CHAPTER - PHYSICAL WORLD, UNITS AND DIMENSIONS & ERRORS IN MEASUREMENT EXERCISE - EXERCISE-IV
7 Videos
Similar Questions
A ladder 5m long is leaning against a wall. The foot of the ladder is pulled out along the ground away from from the wall at a rate of 2m/s. How fast is the height of ladder on the decreasing at the instant when the foot of the ladder is 4m away from the wall?
View SolutionTwo parallel metallic plates have surface charge densities σ1 and σ2 as shown in figure.
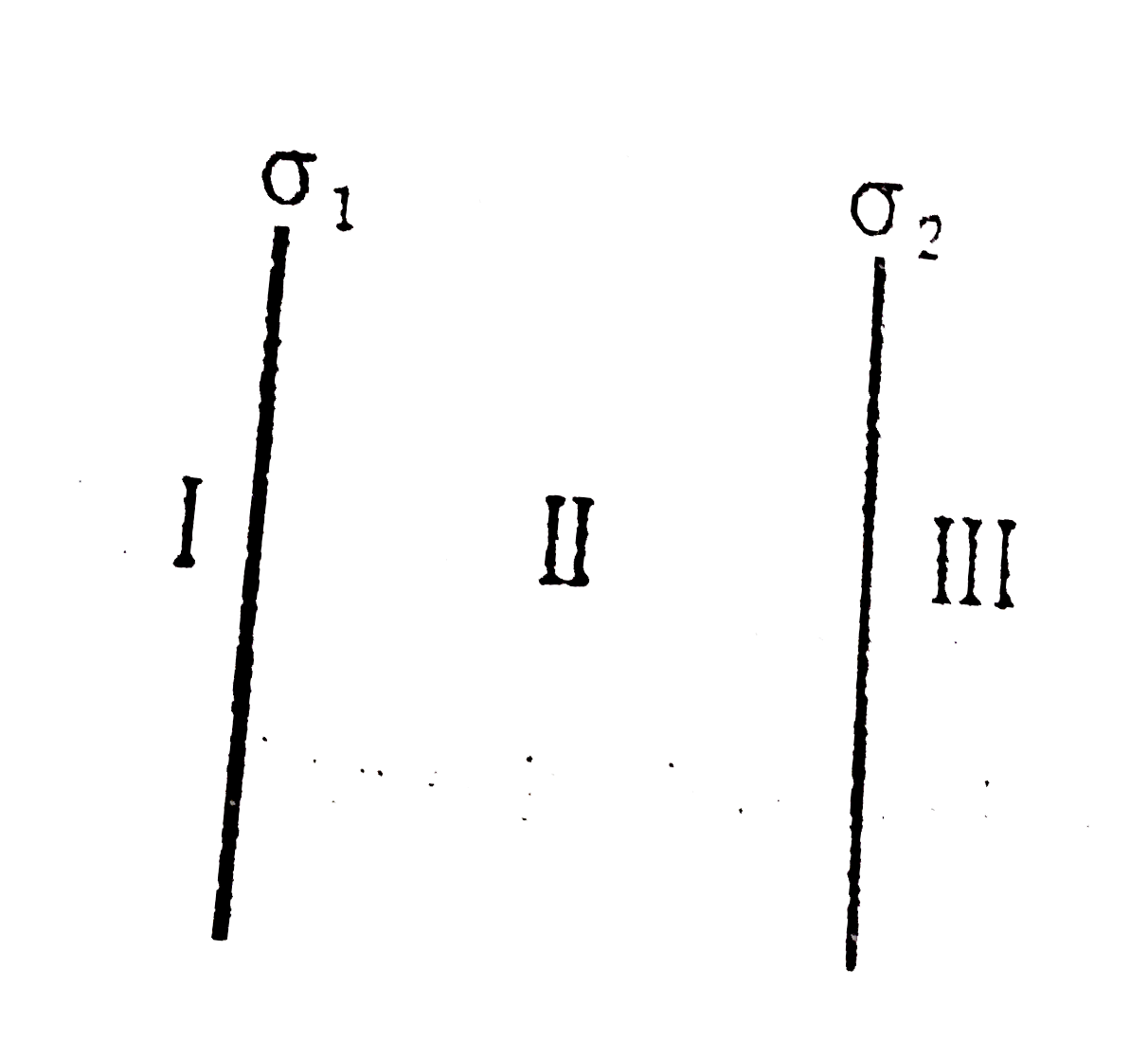
Column-IColumn-II(A)If σ1+σ2=0(p)Electric field in region III is towards right(B)Ifσ1+σ2>0(q)Electric field in region I is zero(C)If σ1+σ2<0(r)Electric field in region I is towards roght(s)Nothing can be said
View SolutionIdentify the pair whose dimensions are equal
View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
View SolutionAssertion : Gravitational forces do not depend on the medium between the masses.
Reason : Mediating particle responsible for gravitational force is graviton.
View SolutionThe dimension of (12)ε0E2 (ε0 : permittivity of free space, E electric field
View SolutionIf L has the dimensions of length, V that of potential and epsilon_(0) is the permittivity of free space then quantity epsilon_(0) LV has the dimensions of
View SolutionThe dimensions of 12ε0E2(ε0= permittivity of free space, E= electric field) is
View Solutionepsilon_(0)E^(2) has the dimensions of ( epsilon_(0)= permittivity of free space, E= electric field) Here k= Boltzmann consant T= absolute temperature R= universal gas constant.
View SolutionFind the dimension of the quantity (1)/(4pi epsilon_(0))(e^(2))/(hc) , the letters have their usual meaning , epsilon_(0) is the permitivity of free space, h, the Planck's constant and c, the velocity of light in free space.
View Solution12ε0E2 की विमा लिखिए (जहाँ ε0 मुक्त स्थाक की वैघुतशीलता तथा E वैघुत क्षेत्र की तीव्रता हैं।
View SolutionThe dimensions of 12∈0E2, where ∈0 is permittivity of free space and E is electric field, is :-
View SolutionThe dimensions of (1)/(2)epsilon_(0)E^(2) (epsilon_(0)= permitivity of free space and E = electric field) are :
View SolutionIf L has the dimensions of length, V that of potential and ε0 is the permittivity of free space then quantity ε0LV have the dimensions of :
View SolutionThe unit of permitivity of free space epsilon_(0) is
View SolutionThe dimension of (1)/(2) epsilon_(0)E^(2) . Where epsilon_(0) is permittivity of free space and E is electric field is
View Solution
