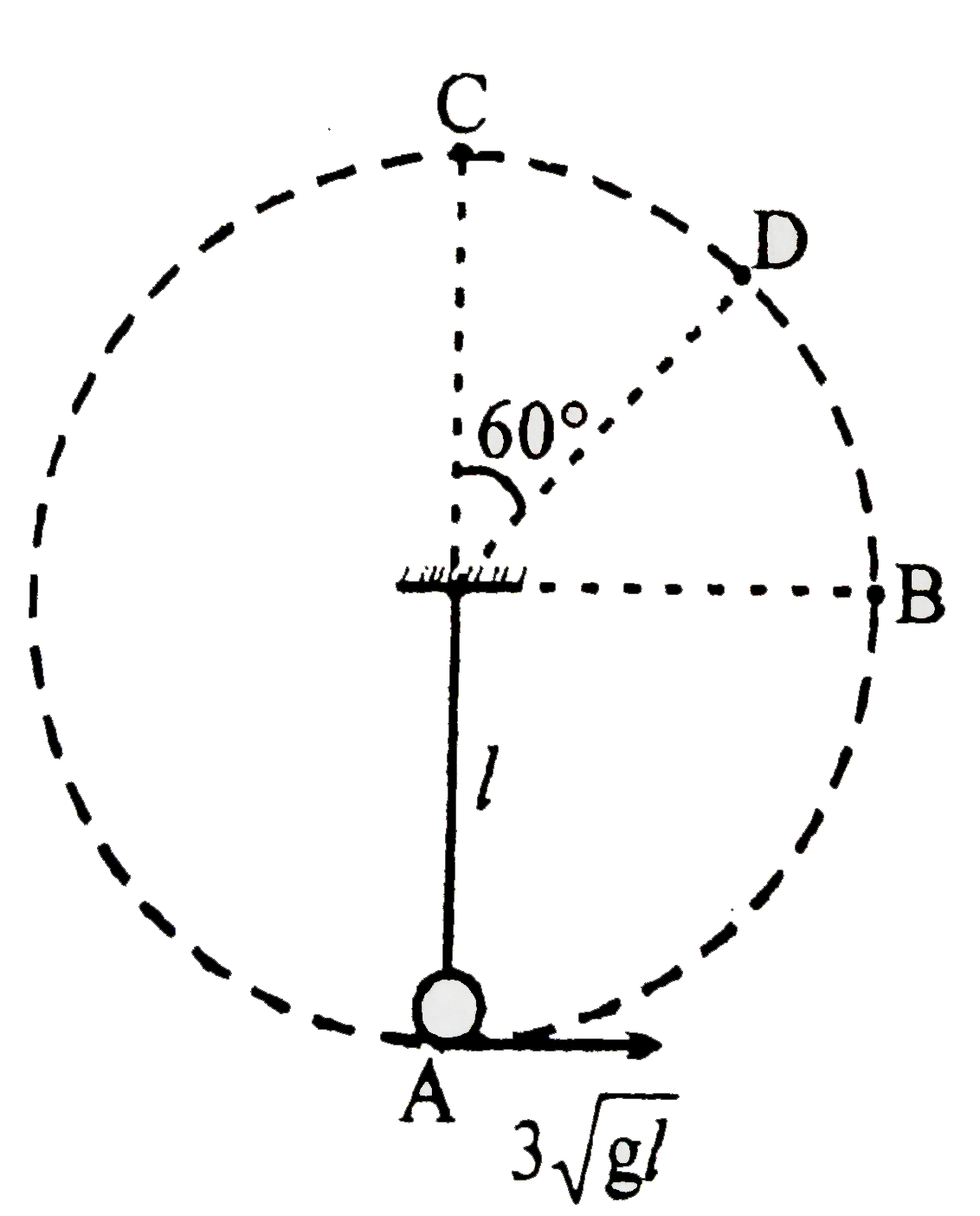
A particle is suspended from a string of length R. It is given a velocity u=3√gR at the lowest point.

column−I column−II(A)Velocity at B (P)7mg(B) Velocity at C (Q)√5qR(C)Tension in string at B(R) (R)√7gR(D)Tension in string atC(S) (S)4mgSolution in Gujrati
A-R, B-Q, C-P, D-S
A-Q, B-R, C-S, D-P
A-R, B-P, C-Q, D-S
A-P, B-S, C-R, D-Q
The correct Answer is:A
Step by step video & image solution for A particle is suspended from a string of length R. It is given a velocity u=3sqrt(gR) at the lowest point. {:(column -I," "column-II),((A) "Velocity at " B," "(P)7mg),((B) " Velocity at "C," " (Q)sqrt(5qR)),((C) "Tension in string at "B(R)," "(R) sqrt(7gR) ),((D) "Tension in string at" C(S)," "(S) 4mg):} by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- TEST PAPERBOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS
4 Videos
- SIMPLE HARMONIC MOTIONBOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Example
1 Videos
- TEST PAPER 1BOOK - ALLEN CHAPTER - TEST PAPER 1EXERCISE - PHYSICS
11 Videos
Similar Questions
Choose the correct statement:
View SolutionChoose the correct statement:
View SolutionA rod with rectangular cross section oscillates about a horizontal axis passing through one of its ends and it behaves like a seconds pendulum, its length will be
View SolutionWhy are electric field lines perpendicular at a point on an equipotential surface of a conductor ?
View SolutionA particle tied to a string describes a vertical circular motion of radius r continually. If it has a velocity √3gr at the highest point, then the ratio of the respective tensions in the string holding it at the highest and lowest points is
View SolutionA particle of mass m is suspended from a string so that it can move in a circular path in vertical plane. It is given v=3√glat the bottom most point. Match the values of tension in string at A,B,C,D
Column IColumn II(A) TA(P) 10 mg ),((B) T_(B),,(Q)7mg),((C) T_(C),,(R)4mg),((D) T_(D),,(S)5mg),(,,(T)5.5mg
View SolutionA particle is suspended from a string of length R . It is given a velocity u=3sqrt(gR) at the lowest point. {:(column -I," "column-II),((A) "Velocity at " B," "(P)7mg),((B) " Velocity at "C," " (Q)sqrt(5qR)),((C) "Tension in string at "B(R)," "(R) sqrt(7gR) ),((D) "Tension in string at" C(S)," "(S) 4mg):}
View SolutionA particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom. Match the following:

Column-1 Column-2(A)Velocity at B(P) 7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5mg(T)None
View SolutionA particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
View SolutionA point mass m is moved in vertical circle of radius r with help of string. Velocity of mass is root(7gr) at lowest point. Tension in string at lowest point is
View SolutionThe velocity of a body revolving in a vertical circle of radius r at the lowest point sqrt(7gr) . The ratio of maximum tensions in the string is
View SolutionThe velocity of a body revolving in a vertical circle of radius .r. at the lowest point sqrt(7gr) . The ratio of maximum to minimum tensions in the string is
View SolutionA particle suspended by a thread of length .l. is projected horizontally with a velocity √3gl at the lowest point. The height from the bottom at which the tension in the string becomes zero is
View SolutionThe velocity of a body revolving in a vertical circle of radius r at the lowest point √7gr. The ratio of maximum tensions in the string is
View SolutionThe velocity of a body revolving in a vertical circle of radius 'r' at the lowest point sqrt(7gr) . The ratio of maximum to minimum tensions in the string is
View SolutionA particle is suspended from a string of length R. It is given a velocity u=3√gR at the bottom

Match the following
Table-1Table-2(A)Velocity at B(P)7 mg(B)Velocity at C(Q)√5gR(C)Tension in string at B(R)√7gR(D)Tension in string at C(S)5 mg(T)None
View Solution
