A particle starts sliding down a frictionless inclined plane. If is the distance travelled by it from time , to , the ratio is Solution in Gujrati
The correct Answer is:C
Topper's Solved these Questions
- KINEMATICSBOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - MCQ with one or more than one correct Question
1 Videos
- KINEMATICSBOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - SUBJECTIVE QUESTION
3 Videos
- KINEMATICSBOOK - ALLEN CHAPTER - KINEMATICSEXERCISE - Exercise-05 [A]
11 Videos
- ERROR AND MEASUREMENTBOOK - ALLEN CHAPTER - ERROR AND MEASUREMENTEXERCISE - Part-2(Exercise-2)(B)
22 Videos
- KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)BOOK - ALLEN CHAPTER - KINEMATICS (MOTION ALONG A STRAIGHT LINE AND MOTION IN A PLANE)EXERCISE - BEGINNER S BOX-7
8 Videos
Similar Questions
The coordinate of a particle moving in a plane are given by x(t)=acos(pt)andy(t)=bsin(pt) where a,b(<a) and P are positive constants of appropriate dimensions . Then
View SolutionA large heavy box is sliding without friction down a smooth plane of inclination θ. From a point P on the bottom of the box, a particle is projected inside the box. The initial speed of the particle with respect to box is u and the direction of projection makes an angle α with the bottom as shown in figure.
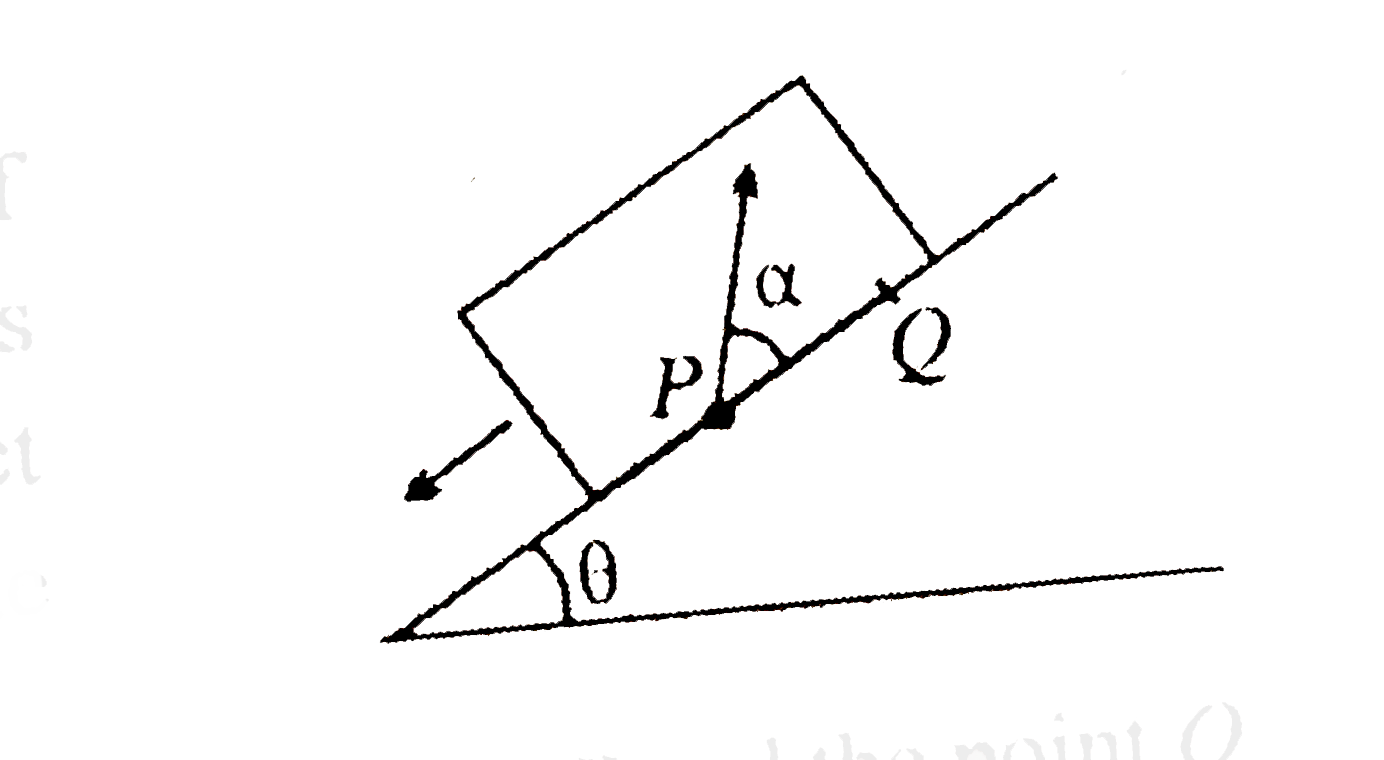
(i) Find the distance along the bottom of the box between the point of projection P and the point Q where the particle lands. (Assume that the particle does not hit any other surface of the box. Neglect air resistance).
(ii) If the horizontal displacement of the particle as seen by an observer on the ground is zero, find the speed of the box with respect to the ground at the instant when the particle was projected.
View SolutionA ball whose kinetic energy is E , is projected at an angle of 45∘ to the horizontal . The kinetic energy of the ball at the highest point of its flight will be
View SolutionA cube has a side of length 1.2×10−2m. Calculate its volume.
View SolutionA football player kicks a ball at an angle of 30∘ with an initial speed of 20 m/s. Assuming that the ball travels in a vertical plane, calculate (a) the time at which the ball reaches the highest point (b) the maximum height reached (c) the horizontal range of the ball (d) the time for which the ball is in the air, (g=10m/s2)
View SolutionLet Sn=1+2+3++n and Pn=S2S2−1⋅S3S3−1⋅S4S4−1⋅⋅⋅SnSn−1 Where n∈N,(n≥2). Then limx→∞Pn=______
View SolutionS_(n)=sum_(n=1)^(n)(n)/(1+n^(2)+n^(4)) then S_(10)S_(20)
View SolutionLet Sn,n=1,2,2.... be the sum of infinite geometric series whose first term is n and the common ratio is . 1n+1.Evaluate Limn→∞S1Sn+S2Sn−1+S3Sn−2+.....+SnS1S21+S22+....+S2n
View SolutionA small block slides without friction down an iclined plane starting form rest. Let Sn be the distance traveled from time t=n−1 to t=n. Then SnSn+1 is:
View SolutionIf Sn denotes the sum of first n terms of an A.P., then
S3n−Sn−1S2n−Sn−1 is equal to
View SolutionIf Sn=1+12+13+…+1n(n∈N), then prove that
S1+S2+..+S(n−1)=(nS((n))−n)or(nS((n−1))−n+1)
View Solutionएक छोटा गुटका एक चिकने आनत तल पर समय t=0 पर विरामावस्था से फिसलता है । माना समय-अन्तराल t=n−1 से t=n में गुटके द्वारा चली गयी दूरी sn है । तब, अनुपात sn/sn+1 है :
View SolutionLet Sn,n=1,2,3,… be the sum of infinite geometric series, whose first term is n and the common ratio is 1n+1. Evaluate limn→∞S1Sn+S2Sn−1+S3Sn−2+...+SnS1S21+S22+......+S2n.
View Solutionएक वस्तु एक घर्षणरहित नत समतल पर प्रारम्भिक विरामावस्था से नीचे ओर सरकती है। यदि sn और sn+1 क्रमशः वस्तु द्वारा n वें और (n+1) वें सेकण्ड में चली गई दूरियाँ हो तो sn+1sn अनुपात है
View Solutionएक वस्तु एक घर्षणरहित नत समतल पर प्रारम्भिक विरामावस्था से नीचे ओर सरकती है। यदि sn और sn+1 क्रमशः वस्तु द्वारा n वें और (n+1) वें सेकण्ड में चली गई दूरियाँ हो तो sn+1sn अनुपात है
View SolutionA small cube falls from rest along a frictionless inclined plane. If this distance travelled between times t = n -1 and t = n be s_(n) then the value of (s_(n))/(s_(n)+1) is
View Solution
