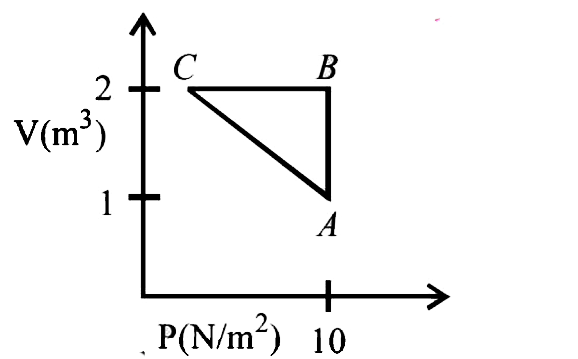
A sample of an ideal non linear triatomic gas has a pressure P0 and temperature T0 taken through the cycle as shown starting from A . Pressure for process C→D is 3 times P0. Calculate heat absorbed in the cycle and work done.
_E01_296_Q01.png)
Solution in Gujrati
The correct Answer is:31P0V0,−5P0V0
For each state n=P0V0T0=P0(7V0/2)TB
=3P0(7V0/2)TC=3P0V0TD
⇒TB=72T0,TC=212T0
∴ Heat absorbed =(nCP.ΔT)A→B+(nCVΔT)B→C
=n4R(52T0)+n3R(7T0)=31.nRT0=31P0V0
Work done = (3P0−P0)(V0−72V0)
=−5P0V0
_E01_296_S01.png)
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 05 (A)
73 Videos
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 05 (B)
58 Videos
- GEOMETRICAL OPTICSBOOK - ALLEN CHAPTER - GEOMETRICAL OPTICSEXERCISE - EXERCISE - 04 (A)
48 Videos
- CURRENT ELECTRICITYBOOK - ALLEN CHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
- GRAVITATIONBOOK - ALLEN CHAPTER - GRAVITATIONEXERCISE - EXERCISE 4
9 Videos
Similar Questions
An aluminium sphere of 20cm diameter is heated from 0∘C to 100∘C. Its volume changes by (given that the coefficient of linear expanison for aluminium (αAl=23×10−6/0C)
View SolutionA vessel contains a mixture of one mole of oxygen and two moles of nitrogen at 300K. The ratio of the average rorational kinetic energy per O2 molecules to that per N2 molecules is
View SolutionTwo rods each of length L2 and coefficient of linear expansion α2 each are connected freely to a third rod of length L1 and coefficient of expansion α1 to form an isoscles triangle. The arrangement is supported on a knife-edge at the midpoint of L1 which is horizontal. what relation must exist between L1 and L2 so that the apex of the isoscles triangle is to remain at a constant height from the knife edge as the temperature changes ?
View SolutionIn the Bohr's model of hy drogen atom, the electron moves around the nucleus in a circular orbit of radius 5×10−11m. Its time period is 1.5×10−16s. The current associated with the electron motion is:-
View SolutionAssertion : The difference in the value of acceleration due to gravity at pole and equator is proportional to square of angular velocity of earth.
Reason : the value of acceleration due to gravity is minimum at the equator and maximum at the pole.
View SolutionAn ideal gas is taken through the cycle A→B→C→A, as shown in the figure, If the net heat supplied to the gas in the cycle is 5J, the work done by the gas in the process CtoA is
View SolutionThermal coefficient of volume expansion at constant pressure for an ideal gas sample of n moles having pressure P_(0) , volume V_(0) , and temperature T_(0) is
View SolutionThe air tight and smooth piston of a cylindrical vessel are connected with a string as shown. Initially pressure and temperature of the gas are P_(0) and T_(0) . The atmospheric pressure is also P_(0) . At a later time, tension in the string is (3)/(8) P_(0)A where A is cthe cross-sectional are of the cylinder. at this time, the temperature of the gas has become.
View SolutionAn ideal monoatomic gas indergoes a pressure pV_(n) =constant. The adiabatic constant for gas is y . During the process, volume of gas increases from V_(0) to rV_(0) and pressure decreases for p_(0) to (p_(0))/(2r) Based on above information, answer the following questios : The molar heat capacity of the gas for the process is
View SolutionPressure versus temperature graph of an ideal gas is shown in figure. Density of the gas at point A is P0. Density at B will be
View Solutionn moles of an ideal gas is taken through a four step cyclic process as shown in the diagram. Calculate work done by the gas in a cycle in terms of temperatures T_(1), T_(2), T_(3) and T_(4)
View SolutionOne mole of an ideal mono atomic gas is at a temperature T0=1000K and its pressure is P0. The gas is adiabatically cooled so that its pressure becomes 23P0. Thereafter, the gas is cooled at constant volume to reduce its pressure to P03 . Calculate the total heat absorbed by the gas during the process.
Take R=253Jmol−1K−1 and (23)2/5=0.85
View SolutionOne mole of an ideal gas is heated at constant pressure from 0^(@)C to 100^(@)C (A) Calculate work done. (B) If the gas were expanded isothermally and reversibly at 0^(@)C from 1 atm to some other pressure P_(1) , water must be the final pressure if the maximum work is equal to the work involned in (a)?
View SolutionOn mole of a monoatomic ideal gas is taken through the cycle shown in figure .
A→B : Adiabatic expansion B→C : Cooling at constant volume
C→D: Adiabatic compression D→A : Heating at constant volume
The pressure and temperature at A,B, etc, are denoted by PA,TA,PB,TB etc., respectively . Given that TA=1000K,PB=(23)PAandPC=(13)PA. Calculate the following quantities: (i) The work done by the gas in the processs A→B (ii) The heat lost by the gas in the process B→C (iii) The temperature TD.(Given:(23)2/5=0.85)
_E01_271_Q01.png)
View SolutionOne mole of a monatomic ideal gas is taken through the cycle shown in figure
The pressures and temperatures at A,B etc, are denoted by PA,TA,PB,TBetc respectively.Given TA=1000K,PB=(2/3)PAandPC=(1/3)PA Then choose the incorrect option.
View Solutionएकपरमाणुक आदर्श गैस का 1 मोल चित्र में दिखाए चक्रीय प्रक्रम में ले जाया जाता है |
A→B : रुद्धोष्म प्रसार
B→C : नियत आयतन पर शीतलन
C→D : रुद्धोष्म संपीडन
D→A : नियत आयतन पर गर्म करना
बिन्दुओं A, B... पर दाब तथा ताप क्रमशः pA,TA,pB,TB... द्वारा प्रदर्शित किए गए है | दिया है TA=1000 केल्विन, pB=(2/3)pA तथा pC=(1/3)pA| गणना कीजिए
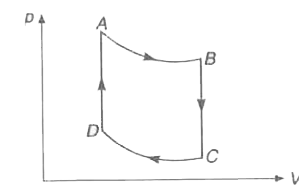
(a) प्रक्रम A→B में गैस द्वारा किया गया कार्य
(b) प्रक्रम B→C में गैस द्वारा ऊष्मा ह्रास
(c ) ताप TD
[दिया है (2/3)2/5=0.85,R=8.31 जूल मोल−1केल्विन−1]
View Solution