Two bodies performing S.H.M. have same amplitude and frequency. Their phases at a certain instant are as shown in the figure. The phase difference between them is
_E01_002_Q01.png)
Solution in Gujrati
(a)116π
(b)π
(c)53π
(d)35π
The correct Answer is:C
_E01_002_S01.png)
cosθ=A/2A=12⇒θ=π3
Total ohase difference between them
ϕ=2θ+π=5π3
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-0219videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column1videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES29videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)25videos
RACE
- BOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
Similar Questions
A mass M is performing linear simple harmonic motion. Then correct graph for acceleration a and corresponding linear velocity v is
02:25View SolutionColumn-I gives different situtions in which bodes are floating in liquid. Column-II gives correspoding time periods of oscillation.
_E01_069_Q01.png)
A cube floating in liquid.04:50View SolutionA simple pendulum has time period 2s. The point of suspension is now moved upward accoding to relation y=(6t−3.75t2)m where t is in second and y is the vertical displacement in upward direction. The new time period of simple pendulum will be
01:45View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
03:39View SolutionChoose the correct statement:
02:29View SolutionPhase difference between two waves having same frequency (v) and same amplitude (A) is 2π/3. If these waves superimpose each other, then resultant amplitude will be–
View SolutionFind the phase different between two particles executing simple harmonic motion with the same amplitude and frequency when their phases at a certain moment are as shown in the figure.
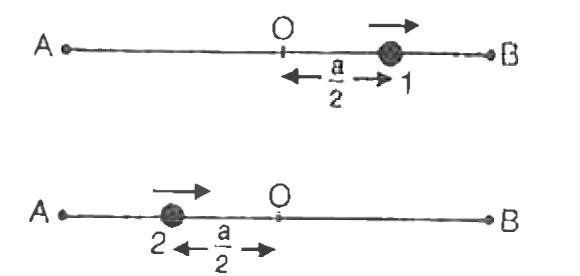
View SolutionThe phase difference between displacement and acceleration of a particle performing S.H.M. is
03:02View SolutionTwo particles execute SHM of same amplitude and same time period, about same mean position but with a phase difference between them. At an instant they are found to cross each other at x=+A3. The phase difference between them is
03:32View SolutionTwo particles P and Q performs S.H.M. of same amplitude 'A' and frequency n along the same straight line the resultant S.H.M. have the amplitude sqrt(2) times amplitude of individual. The initial phase difference between two particles will be nearly.
01:45View SolutionIf the two particles performing S.H.M. with same amplitude and initial phase angle, then the initial phase angle of resultant motion depends on
03:33View SolutionIf the two particles performing S.H.M. with different initial phase angle and amplitude, then the initial phase angle of resultant motion depends on
03:26View SolutionIf the two particles performs S.H.M. of same initial phase angle but different amplitudes of individuals, then the resultant motion initial phase angle depends on
03:18View SolutionThe two particles performing S.H.M. have a phase difference of π. If the amplitude of second particle is three times the amplitude of first particle, then the amplitude of resultant motion will be
05:10View SolutionThe phase difference between displacement and acceleration of a particle performing S.H.M. is
04:46View SolutionThe resultant of two S.H.M. having the same period T but different amplitudes and phases, along the same line is S.H.M
03:02View Solution