A particle performing SHM is found at its equilibrium position at t = 1s and it is found to have a speed 0.25m/s at t=2s. If the period of oscillation is 6. Calculate amplitude of oscillation. Solution in Gujrati
32πm
34πm.
6πm
38πm
The correct Answer is:A
x=asin(ωt+ϕ)
at t=1s,x=0=asin(ωt+ϕ)⇒ϕ=−ω
∵v=aωcos(ωt+ϕ)
∵ At t=2s,14=aωcos(2ω+ϕ)
⇒14=a(2π6)cos(ω)=aπ3cos(π3)=aπ3(12)⇒a=32π
Topper's Solved these Questions
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-0219videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise- 3 Match The Column1videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - SOME WORKED OUT EXAMPLES29videos
SIMPLE HARMONIC MOTION
- BOOK - ALLEN CHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)25videos
RACE
- BOOK - ALLEN CHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
Similar Questions
A mass M is performing linear simple harmonic motion. Then correct graph for acceleration a and corresponding linear velocity v is
05:37View SolutionColumn-I gives different situtions in which bodes are floating in liquid. Column-II gives correspoding time periods of oscillation.
_E01_069_Q01.png)
A cube floating in liquid.04:21View SolutionA simple pendulum has time period 2s. The point of suspension is now moved upward accoding to relation y=(6t−3.75t2)m where t is in second and y is the vertical displacement in upward direction. The new time period of simple pendulum will be
03:04View SolutionThe frequency of a radar is 780 MHz. After getting reflected from an approaching aeroplane, the apparent frequency is more than the actual frequency by 2.6 kHz. The aeroplane has a speed of
00:48View SolutionChoose the correct statement:
04:21View Solution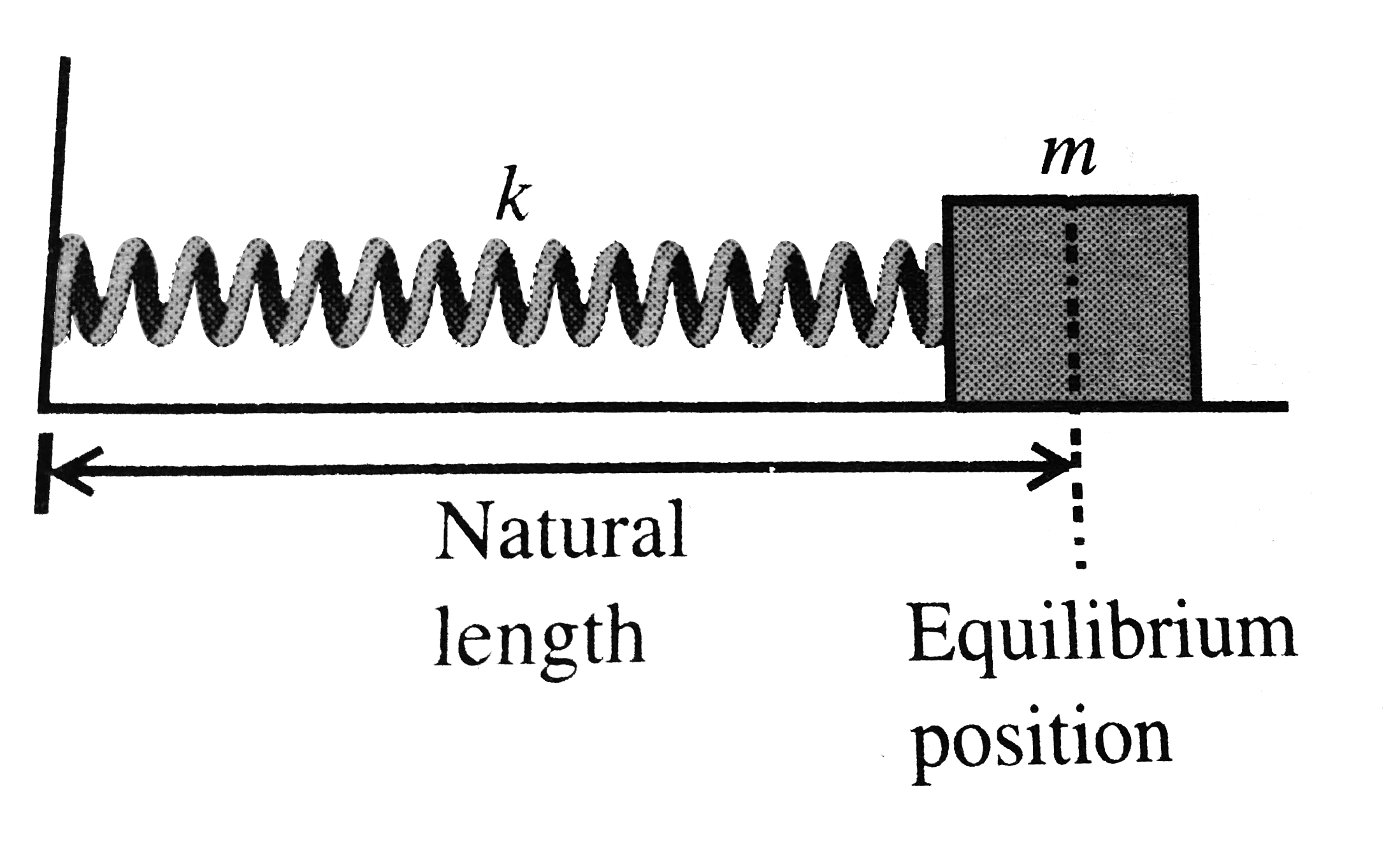
A block of mass m is connected to a spring of spring constant k as shown in Fig. The block is found at its equilibrium position t=1s and it has a velocity of +0.25ms at t=2s. The time period of oscillation is 6 s. Based on the given information answer the following question: Q. the amplitude of oscillation is03:49View SolutionA particle performing SHM is found at its eqilbrium at t=1sec.and it is found to have a speed of 0.25m/s at t=2sec. If the period of oscillation is 6sec. Calculate amplitude of oscillation-
02:44View SolutionA particle perform SHM with period T and amplitude A. the mean velocity of the particle averaged over quarter oscillation, Starting from right exterme position is
12:20View SolutionA particle executes simple harmonic oscillation with an amplitudes a. The period of oscillation is T. The minimum time taken by the particle to travel half of the amplitude from the equilibrium position is
03:28View SolutionA particle performing SHM is found at its equilibrium position at t = 1s and it is found to have a speed 0.25 = m//s at t=2s. If the period of oscillation is 6. Calculate amplitude of oscillation.
02:23View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is πm/s. Amplitude of oscillation is
(cos45∘=1√2)05:03View SolutionFor a particle performing linear SHM, its average speed over one oscillation is (where, a= amplitude of SHM, n=frequency of oscillation)
03:59View SolutionIf a particle performing S.H.M. from the mean position of amplitude 'A' and period 'T', then the lapse of fraction of period in (7//8)^(th) oscillation will be
03:30View SolutionA particle executes simple harmonic oscillation with an amplitude a.The period of oscillation is T. The minimum time taken by the particle to travel half of the amplitude from the equilibrium position is :
02:36View Solutionसरल आवर्त गति कर रहा एक कण t−1 s पर साम्यवस्था पर पाया जाता है तथा t−2 s पर इसका वेग 0.25m/s पाया जाता है।यदि दोलनकाल 8s है।
02:54View SolutionA particle performing SHM starts equilibrium position and its time period is 16 seconds. After 2 seconds its velocity is pi m//s . Amplitude of oscillation is (cos 45^(@) = 1/(sqrt(2)))
02:38View Solution