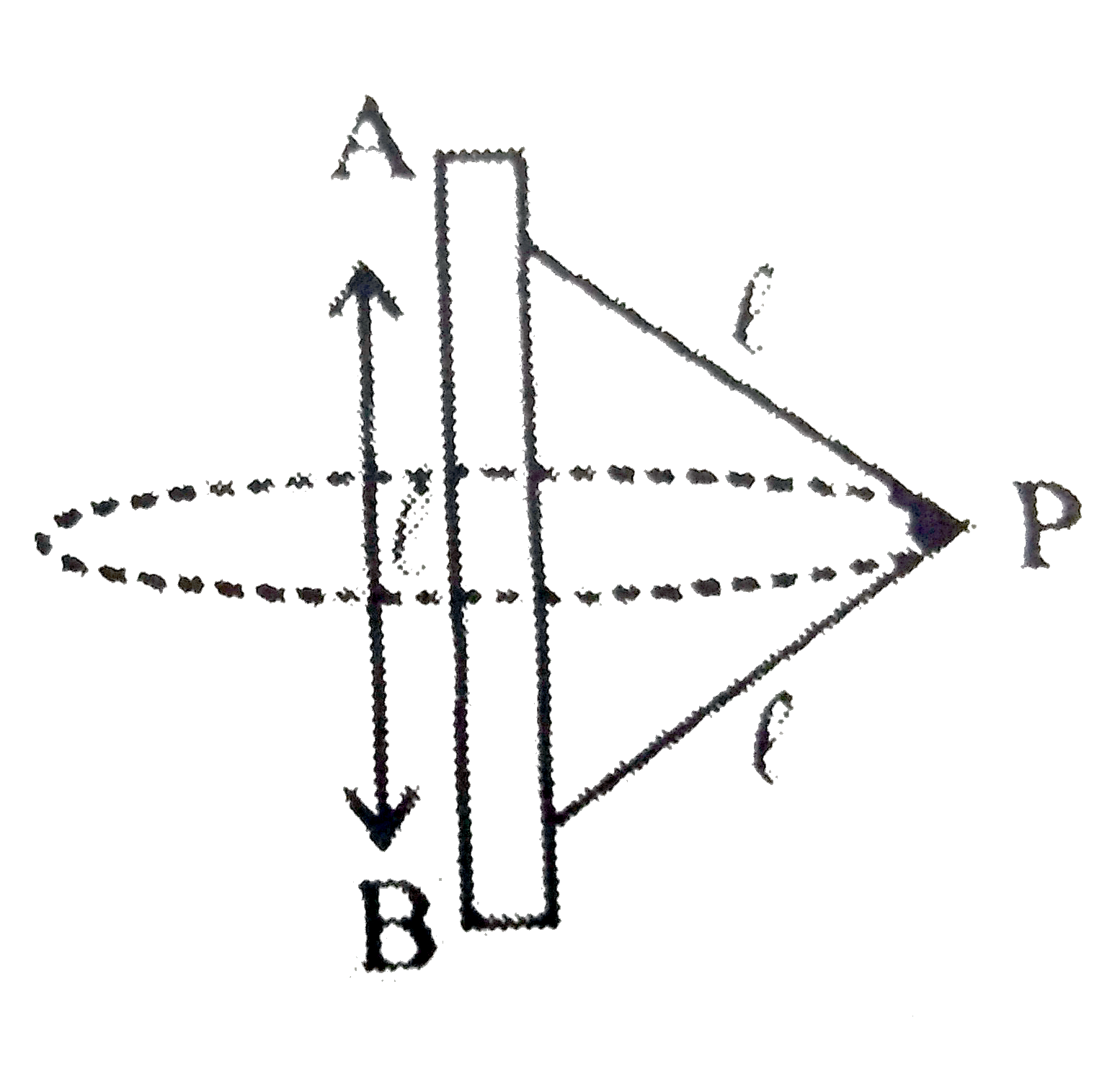
A particle P of mass m is attached to a vertical axis by two strings AP and BP of length l each. The separation AB=l. P rotates around the axis with an angular velocity ω. The tensions in the two strings are T1andT2
 Solution in Gujrati
Solution in Gujrati
T1=T2
T1+T2=mω2l
T1−T2=2mg
BP will remain taut only if ω≥√2gl
The correct Answer is:B, C, D
Step by step video & image solution for A particle P of mass m is attached to a vertical axis by two strings AP and BP of length l each. The separation AB=l. P rotates around the axis with an angular velocity omega. The tensions in the two strings are T_(1) and T_(2) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - Martrix match
1 Videos
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE(O-2)
7 Videos
- CIRCULAR MOTIONBOOK - ALLEN CHAPTER - CIRCULAR MOTIONEXERCISE - EXERCISE(O-1)
22 Videos
- BASIC MATHSBOOK - ALLEN CHAPTER - BASIC MATHSEXERCISE - Question
1 Videos
- CURRENT ELECTRICITYBOOK - ALLEN CHAPTER - CURRENT ELECTRICITYEXERCISE - EX.II
66 Videos
Similar Questions
A block is placed on a horizontal table which can rotate about its axis.The block is placed at certain distance from centre as shown in figure. Table rotates such that particle does not slide. See possible direction of net acceleration of block at the instant shown in figure
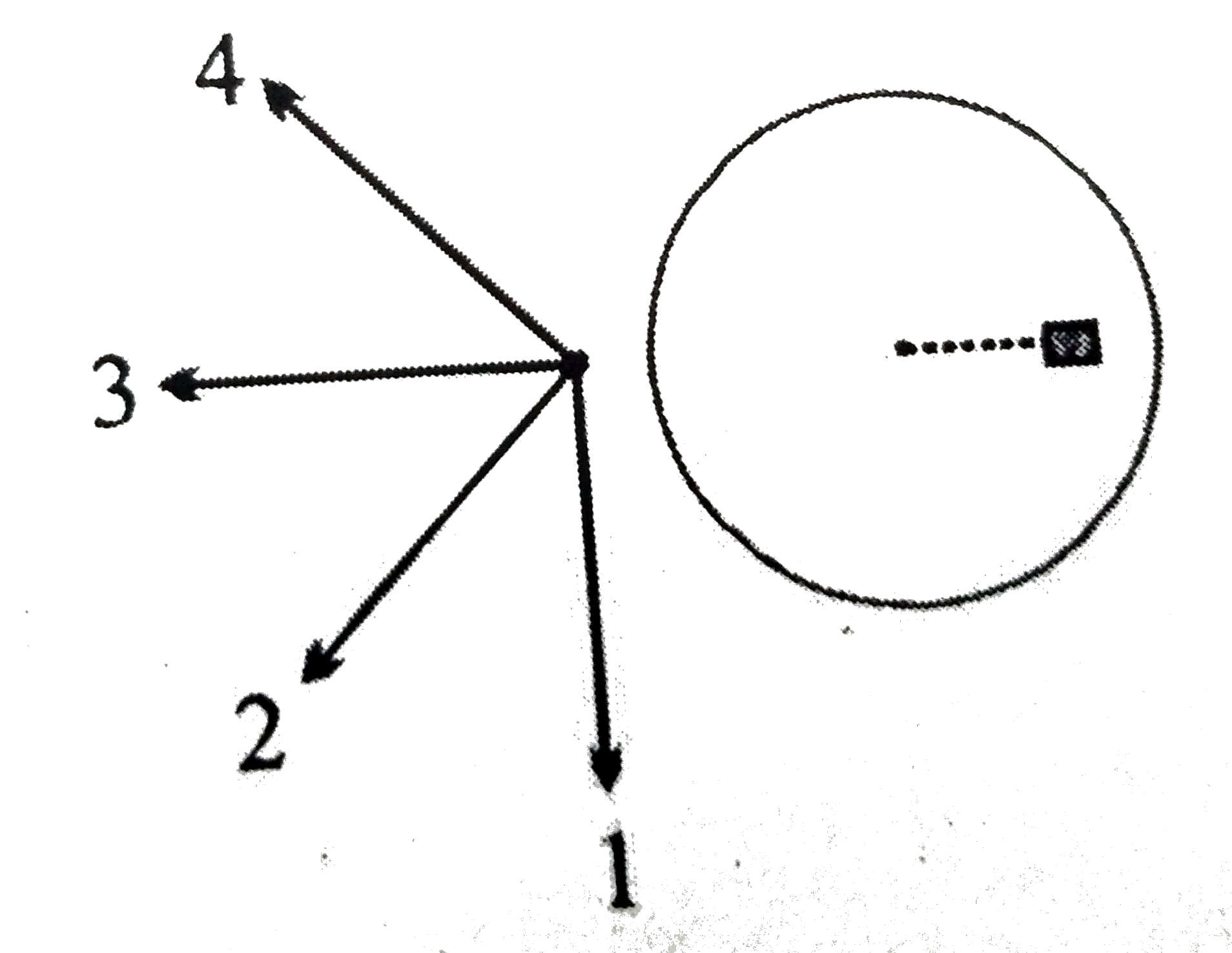
Column-IColumn-II(A)When rotation is clockwise with constant ω(P)1(B)When rotation is clock wise with decreasing ω(Q)2(C)When rotation is clockwise with increasing ω(R)3(D)Just after clockwise rotation beings from rest(S)4
View SolutionA ring of radius 'r' and mass per unit length 'm' rotates with an angular velocity 'ω' in free space then the tension is :
View SolutionThe area of the parallelogram represented by the vectors →A=2ˆi+3ˆj and →B=ˆi+4ˆj is
View SolutionFind dydx for the following :
(i) y=x7/2 (ii) y=x−3
(iii)y=x (iv) y=x5+x3+4x1/2+7
(v) y=5x4+6x3/2+9x (vi) y=ax2+bx+C
(Vii)y=3x^(5)-3x-1/x
View SolutionIn the Bohr's model of hy drogen atom, the electron moves around the nucleus in a circular orbit of radius 5×10−11m. Its time period is 1.5×10−16s. The current associated with the electron motion is:-
View SolutionA mass is supported on a frictionless horizontal surface. It Is attached to a string and rotates about a fixed center at an angular velocity omega_(0) .If the length of the string and angular velocity both are doubled, the tension in the string which was initially T_(0) is now
View SolutionA particle A of mass m is attached to a vertical axis by two stings PA and QA of lengths 3L and 4L , respectively. PQ=5L . A rotates around the axis with an angular speed omega . The tension in the two strings are T_(1) and T_(2) . (i) T_(1)=T_(2) (ii) 3T_(1)-4T_(2)=5mg (iii) 4T_(1)+3T_(2)=12momega^(2)L
View SolutionA uniform rod of mass m and length L is suspended with two massless strings as shown in figure. If the rod is at rest in a horizontal position the ratio of tension in the two strings T_(1)//T_(2) is
View SolutionA uniform thin rod AB of mass M and length l attached to a string OA of length (l)/(2) is placed on a smooth horizontal plane and rotates with angular velocity omega around a vertical axis through O. A peg P is inserted in the plane in order that on striking it the bar will come exactly to rest
View SolutionA uniform thin rod AB of mass M and length l attached to a string OA of length (l)/(2) is placed on a smooth horizontal plane and rotates with angular velocity omega around a vertical axis through O. A peg P is inserted in the plane in order that on stricking it the bar will come exactly to rest
View SolutionA particle of mass m is attached to a vertical rod with two inextensible strings AO and BO of equal lengths l. Distance between A and B is also l. The setup is rotated with angular speed ω with rod as the axis.
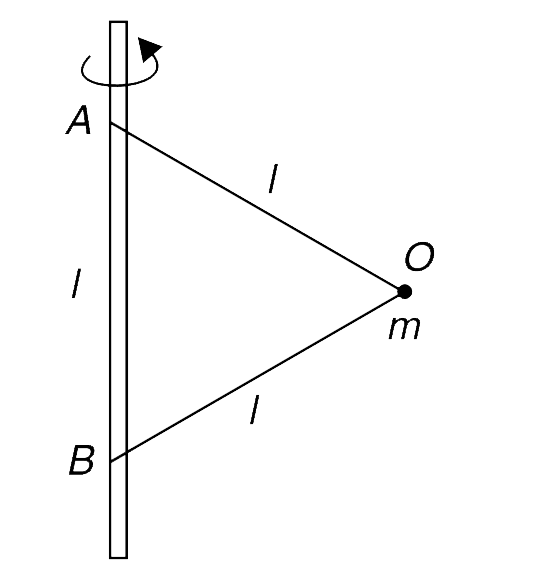
(a) Find the values of ω for which the particle remains at point B.
(b) Find the range of values of ω for which tension (T1) in the string AO is greater than mg but the other string remains slack
(c) Find the value of ω for which tension (T1) in string AO is twice the tension (T2) in string BO
(d) Assume that both strings are taut when the string AO breaks. What will be nature of path of the particle moment after AO breaks ?
View SolutionThe particle P of mass m is attached to two light, rigid rods AP and BP of length l each. A and B are hings on a fixed vertical axis. The system APB can rotate freely about this axis. The angle ABP = the angle BAP = theta . The tensions in AP and BP are T_(1) and T_(2) respectively. When the system is at rest, which of the following is not correct?
View SolutionThe particle P of mass m is attached to two light, rigid rods AP and BP of length l each. A and B are hings on a fixed vertical axis. The system APB can rotate freely about this axis. The angle ABP = the angle BAP=θ. The tensions in AP and BP are T1 and T2 respectively.
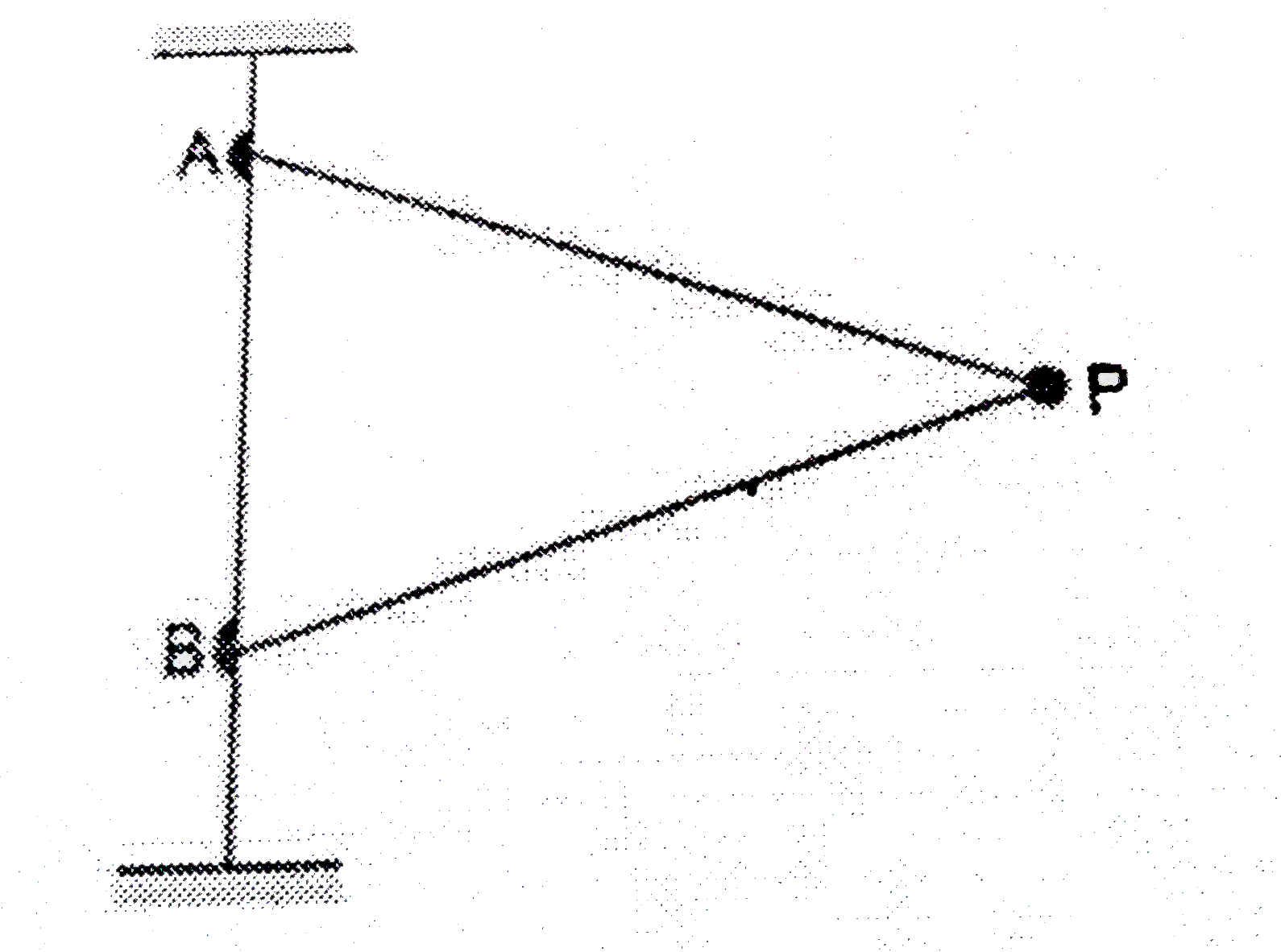
When the system is made to rotate about AB with angular velocity ω, which of the following is not correct?
View SolutionThe particle P of mass m is attached to two light, rigid rods AP and BP of length l each. A and B are hings on a fixed vertical axis. The system APB can rotate freely about this axis. The angle ABP = the angle BAP = theta . The tensions in AP and BP are T_(1) and T_(2) respectively. The system is now rotated by 90^(@) so that must be imparted to P, normal to the plane of the figure, such that it moves in a complete circular path in a vertical plane with AB as the axis?
View SolutionA uniform thin rod AB of mass M and length l attached to a string OA of length =12 is supported by a smooth horizontal plane and rotates with angular velocity ω around a vertical axis through O. A peg P is inserted in the plane in order that on striking it the rod will come to rest.
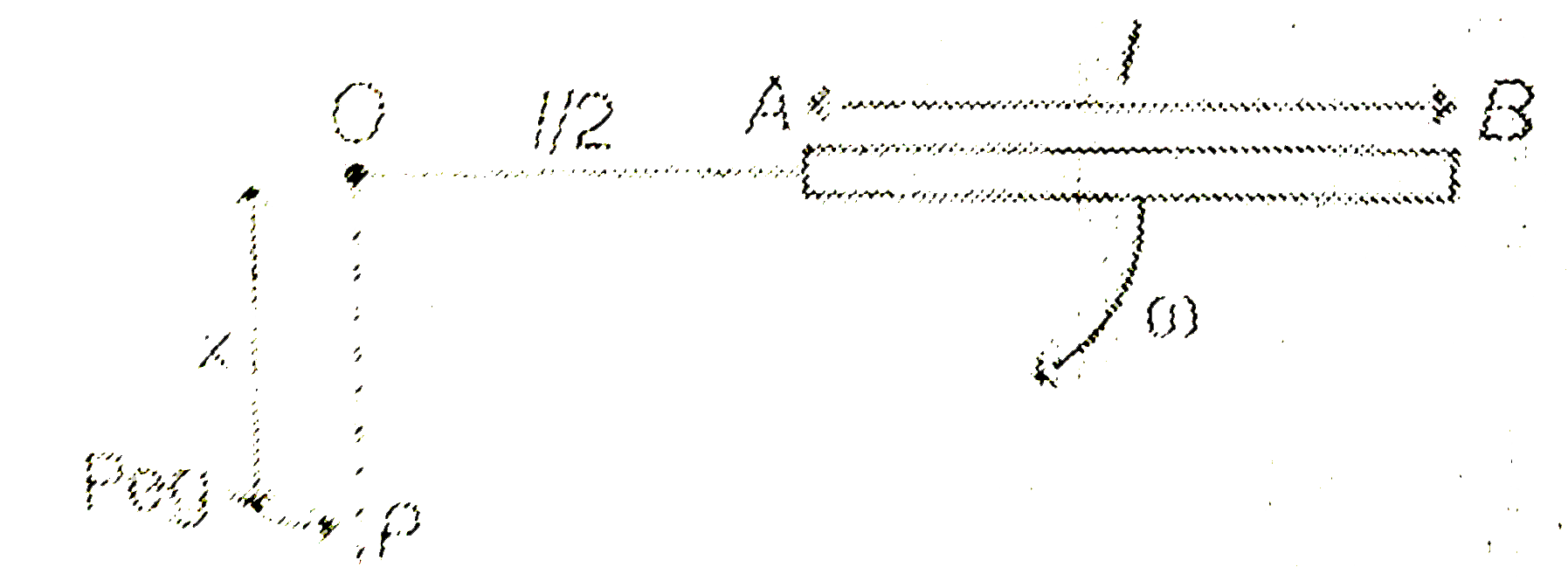
View SolutionA mass attached to a string rotates about a fixed centre with an angular velocity omega in a horizontal plane ,The length of the string and the angular velocity are now doubled .IF T_(0) is the initial tension in the string , then the new tension will be
View Solution
