Question

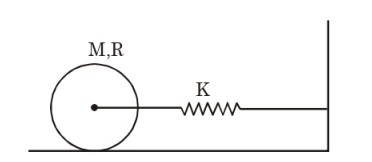
A 4 kg roller is attached to a massless spring of spring constant k = 100 N/m. It rolls without slipping along a frcitionless horizontal road. The roller is displaced from its equilibrium position by 10 cm and then released. It rolls without slipping along a frictionless horizontal road. The roller is displaced from its equilibrium position by 10 cm and then released. Its maximum speed will be

Options

A. `0.5ms^(-1)`
B. `0.6ms^(-1)`
C. `0.4ms^(-1)`
D. `0.8ms^(-1)`

Explanation

To find the maximum speed of the roller when it is displaced from its equilibrium position and then released, we can follow these steps:

1. Identify the Given Values:
- Mass of the roller, \( m = 4 \, \text{kg} \)
- Spring constant, \( k = 100 \, \text{N/m} \)
- Displacement from equilibrium, \( x = 10 \, \text{cm} = 0.1 \, \text{m} \)

2. Calculate the Potential Energy Stored in the Spring:
The potential energy (PE) stored in the spring when it is displaced from its equilibrium position is given by the formula:
\( PE = \frac{1}{2} k x^2 \)
Substituting the values:
\( PE = \frac{1}{2} \times 100 \, \text{N/m} \times (0.1 \, \text{m})^2 \)
\( PE = \frac{1}{2} \times 100 \times 0.01 = \frac{1}{2} \, \text{J} = 0.5 \, \text{J} \)

3. Apply the Conservation of Mechanical Energy:
When the roller is released, the potential energy will convert into kinetic energy (KE) at the equilibrium position. At the equilibrium position, the potential energy is zero, and all the energy is kinetic:
\( KE = \frac{1}{2} m v^2 \)
Setting the potential energy equal to the kinetic energy:
\( 0.5 \, \text{J} = \frac{1}{2} \times 4 \, \text{kg} \times v^2 \)

4. Solve for Maximum Speed \( v \):
Rearranging the equation to solve for \( v^2 \):
\( 0.5 = 2 v^2 \quad \Rightarrow \quad v^2 = \frac{0.5}{2} = 0.25 \)
Taking the square root to find \( v \):
\( v = \sqrt{0.25} = 0.5 \, \text{m/s} \)

5. Conclusion:
The maximum speed of the roller when it reaches the equilibrium position is:
\( v = 0.5 \, \text{m/s} \)