What is the need of combining different resistors? What is the resulant resistance when number of resistances are connected in series?
To solve the question, we will break it down into two parts:
1. The need for combining different resistors.
2. The resultant resistance when resistors are connected in series.
Step 1: Understanding the Need for Combining Different Resistors
Combining different resistors is often necessary for several reasons:
- Achieving Desired Resistance: By combining resistors, we can create a specific resistance value that may not be available as a single resistor. This is particularly useful in circuits where precise resistance values are required.
- Power Distribution: Different resistors can handle different power ratings. By combining them, we can distribute the power load more effectively, preventing any single resistor from overheating or failing.
- Circuit Design Flexibility: In designing circuits, engineers may need to adjust the resistance to achieve desired current and voltage levels. Combining resistors allows for more flexibility in circuit design.
- Cost Efficiency: Sometimes, using multiple lower-value resistors can be more cost-effective than using a single high-value resistor.
Step 2: Resultant Resistance in Series
When resistors are connected in series, the total or resultant resistance (R_total) can be calculated using the formula:
Rtotal=R1+R2+R3+…+Rn
Where R1,R2,R3,…,Rn are the individual resistances of the resistors connected in series.
Example Calculation:
If we have three resistors with values:
- R1=4Ω
- R2=6Ω
- R3=10Ω
The resultant resistance would be:
Rtotal=R1+R2+R3
Rtotal=4Ω+6Ω+10Ω
Rtotal=20Ω
Summary
- The need for combining different resistors includes achieving desired resistance values, distributing power, providing circuit design flexibility, and cost efficiency.
- The resultant resistance when resistors are connected in series is the sum of their individual resistances.
2. The resultant resistance when resistors are connected in series.
If we have three resistors with values:
- R1=4Ω
- R2=6Ω
- R3=10Ω
Rtotal=4Ω+6Ω+10Ω
Rtotal=20Ω
- The resultant resistance when resistors are connected in series is the sum of their individual resistances.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The equivalent resistance of resistors connected in series is always
AEqual to the mean of component resistorsBLess than the lowest of component resistorsCIn between the lowest and the highest of component resistorsDEqual to sum of component resistorsThe reciprocal of the combined resistance of any number of resistance connected in parallel is equal to
Athe sum of reciprocals of individual resistancesBreciprocal of the product of individual resistancesCreciprocal of sum of all the resistancesDNone of the aboveChoose the appropriate set of apparatus for performing the experiment to determine the equivalent resistance of two resistors when connected in series :
AVoltmeter, Ammeter, Two resistors, Key, BatteryBAmmeter, Two resistors, Key, Connecting wires, Rheostat, BatteryCKey, Rheostat, Voltmeter, Two resistors, Battery, Connecting wiresDBattery, Ammeter, Connecting wires, Rheostat Voltmeter, Two resistors, Key
The equivalent resistance of number of resistors will be lesser,if they are connected in..
View SolutionThe resultant resistance of n wires each of resistance r ohms is R, when they are connected in parallel. When these n resistances are connected in series, the resultant resistance will be
View SolutionThe resultant resistance of n wires each of resistance r ohms is R ,when they are connected in parallel.When these n resistances are connected in series,the resultant resistance will be
View SolutionA total resistance of 5.00Ω is to be produced by connecting an unknown resistance. a) What must be the value of the unknown resistance and (b) should it be connected in series or in parallel (c) What is the total resistance if the unknown resistance is connected the other way?
View SolutionWhat is resistance of following resistor.
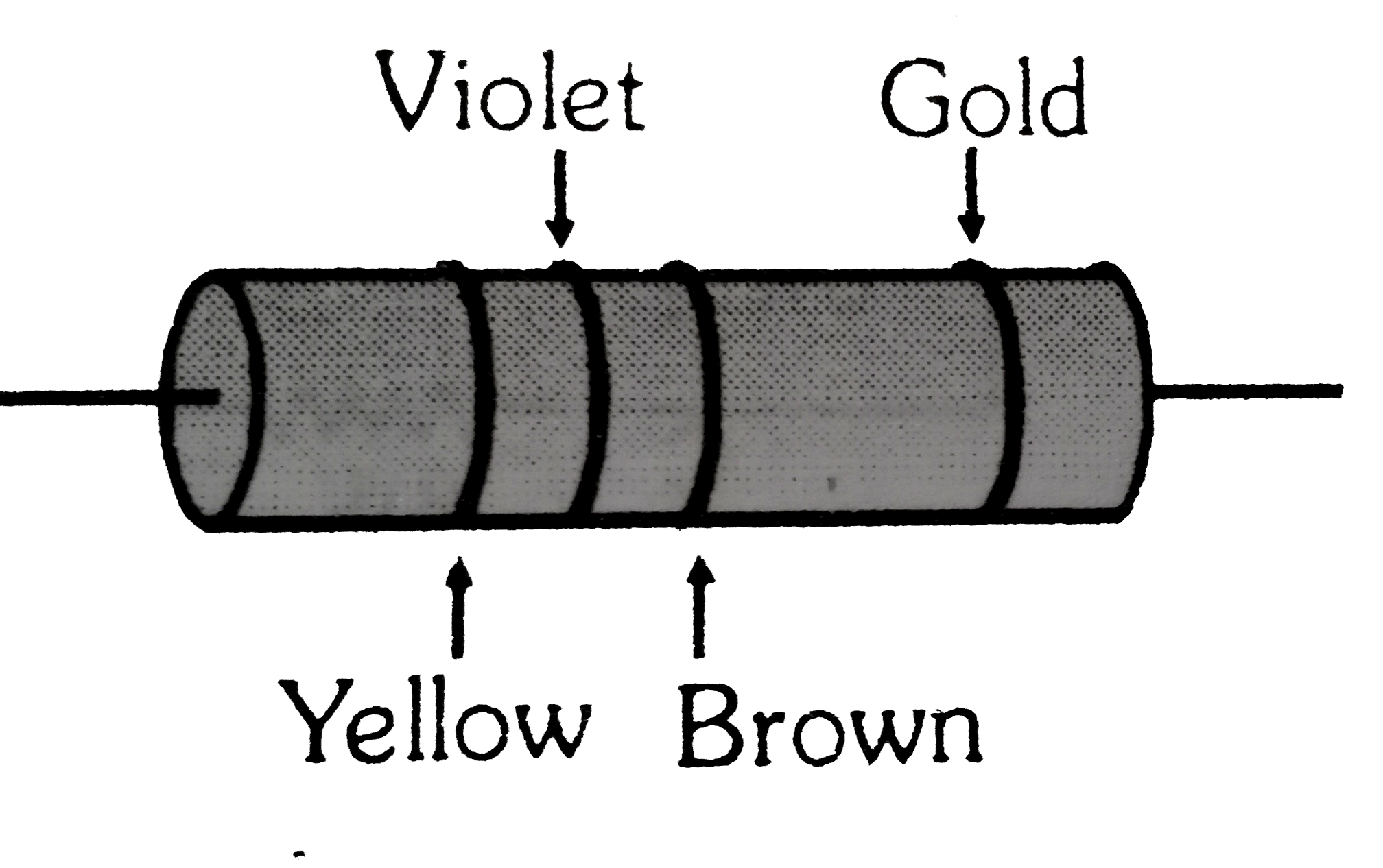
View Solution
