ABCDEFGH is inscribed in a circle with centre at O. The ratio of ∠OAB to ∠AOB is equal to:
O केंद्र वाले एक वृत्त के अंदर एक नियमित अष्टभुज ABCDEFGH निर्मित है| ∠AOB के साथ ∠OAB का अनुपात क्या होगा?
8:3
3:2
4:3
3:1
To find the ratio of angle OAB to angle AOB in the inscribed octagon ABCDEFGH, we can follow these steps:
Step 1: Understand the Geometry
Since ABCDEFGH is a regular octagon inscribed in a circle with center O, we can visualize the octagon and the angles formed at the center and at the vertices.
Step 2: Calculate Angle AOB
The angle AOB is the central angle subtended by arc AB. Since the octagon has 8 equal sides, the entire circle (360 degrees) is divided into 8 equal parts:
Angle AOB=360∘8=45∘
Step 3: Analyze Triangle OAB
In triangle OAB, we have:
- OA = OB (both are radii of the circle)
- Therefore, triangle OAB is an isosceles triangle.
Step 4: Use the Triangle Angle Sum Property
The sum of angles in triangle OAB is 180 degrees:
Angle OAB+Angle ABO+Angle AOB=180∘
Since angle OAB and angle ABO are equal (let's denote them as x):
x+x+45∘=180∘
2x+45∘=180∘
2x=180∘−45∘=135∘
x=135∘2=67.5∘
Thus, angle OAB = 67.5 degrees.
Step 5: Find the Ratio
Now we can find the ratio of angle OAB to angle AOB:
Ratio=Angle OABAngle AOB=67.5∘45∘
To simplify this:
Ratio=67.545=67.5÷22.545÷22.5=32
Final Answer
The ratio of angle OAB to angle AOB is:
32
Since ABCDEFGH is a regular octagon inscribed in a circle with center O, we can visualize the octagon and the angles formed at the center and at the vertices.
The angle AOB is the central angle subtended by arc AB. Since the octagon has 8 equal sides, the entire circle (360 degrees) is divided into 8 equal parts:
Angle AOB=360∘8=45∘
In triangle OAB, we have:
- OA = OB (both are radii of the circle)
- Therefore, triangle OAB is an isosceles triangle.
The sum of angles in triangle OAB is 180 degrees:
Angle OAB+Angle ABO+Angle AOB=180∘
Since angle OAB and angle ABO are equal (let's denote them as x):
x+x+45∘=180∘
2x+45∘=180∘
2x=180∘−45∘=135∘
x=135∘2=67.5∘
Thus, angle OAB = 67.5 degrees.
Now we can find the ratio of angle OAB to angle AOB:
Ratio=Angle OABAngle AOB=67.5∘45∘
To simplify this:
Ratio=67.545=67.5÷22.545÷22.5=32
The ratio of angle OAB to angle AOB is:
32
Similar Questions
In figure PQ is tangent to the circle with centre at O at the point B , if ∠AOB=100∘, then ∠ABP is equal to
 View Solution
View SolutionA quadrilateral ABCD is inscribed in a circle with centre O.If ∠BOC=92∘ and ∠ADC=112∘ , then ∠ABO is equal to:
एक चतुर्भुज ABCD किसी वृत्त के भीतर स्थित है जिसका केंद्र O है | यदि ∠BOC=92∘ और ∠ADC=112∘ है, तो ∠ABO ज्ञात करें |View Solution
Knowledge Check
- Question 1 - Select One
ABCDEFGH is a regualr octagon inscribedin a circle with centre at 0. The ratio of ∠OAB to ∠AOB is equal to :
A4:3B3:1C3:2D8:3 - Question 2 - Select One
If an equilateral triangle ABC is inscribed in a circle with centre O then the measure of ∠AOB is
A60∘B90∘C120∘D180∘ - Question 3 - Select One
O is the centre of a circle ∠AOB=90∘and∠BOC=120∘.∠ABC is equal to
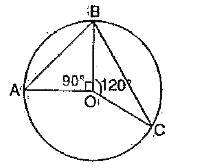 A150∘B210∘C75∘D105∘
A150∘B210∘C75∘D105∘
Two tangents PA and PB are drawn to a circle with centre O from an external point P. If ∠OAB=30∘, then ∠APB is:
केंद्र O वाले वृत्त पर एक बाहरी बिंदु P से दो स्पर्श रेखाएँ PA तथा PB खींची जाती हैं | यदि ∠OAB=30∘ है, तो ∠APB का मान क्या होगा ?View SolutionAB is a diameter of a circle with centre O, and P is a point on the circle. If ∠POA=130∘, the ∠PBO is equal to:
AB केंद्र O के साथ एक वृत्त पर एक व्यास है, और P वृत्त का एक बिंदु है। यदि ∠POA=130∘, तो ∠PBO का मान ज्ञात करे|View SolutionIf PA and PB are tangents drawn from an external point P to a circle with centre O such that ∠APB=70∘, then ∠OAB is equal to:
यदि PA और PB एक बाहरी बिंदु P से केंद्र O के साथ एक वृत्त में खींची गई स्पर्श रेखाएँ हैं जैसे कि ∠APB=70∘ तो ∠OAB बराबर है:View SolutionIf PA and PB are tangents to the circle with centre O such that ∠APB=50∘ then ∠OAB is equal to
View SolutionC is a point on the minor arc AB a circle with centre O. If ∠AOB=100∘, what is ∠ACB ?
View Solution

