A vertical tower PQ subtends the same anlgle of 30∘ at each of two points A and B ,60 m apart on the ground .If AB subtends an angle of 120∘ at P the foot of the tower ,then find the height of the tower .Solution in Bengali
In triagle APQ, we have
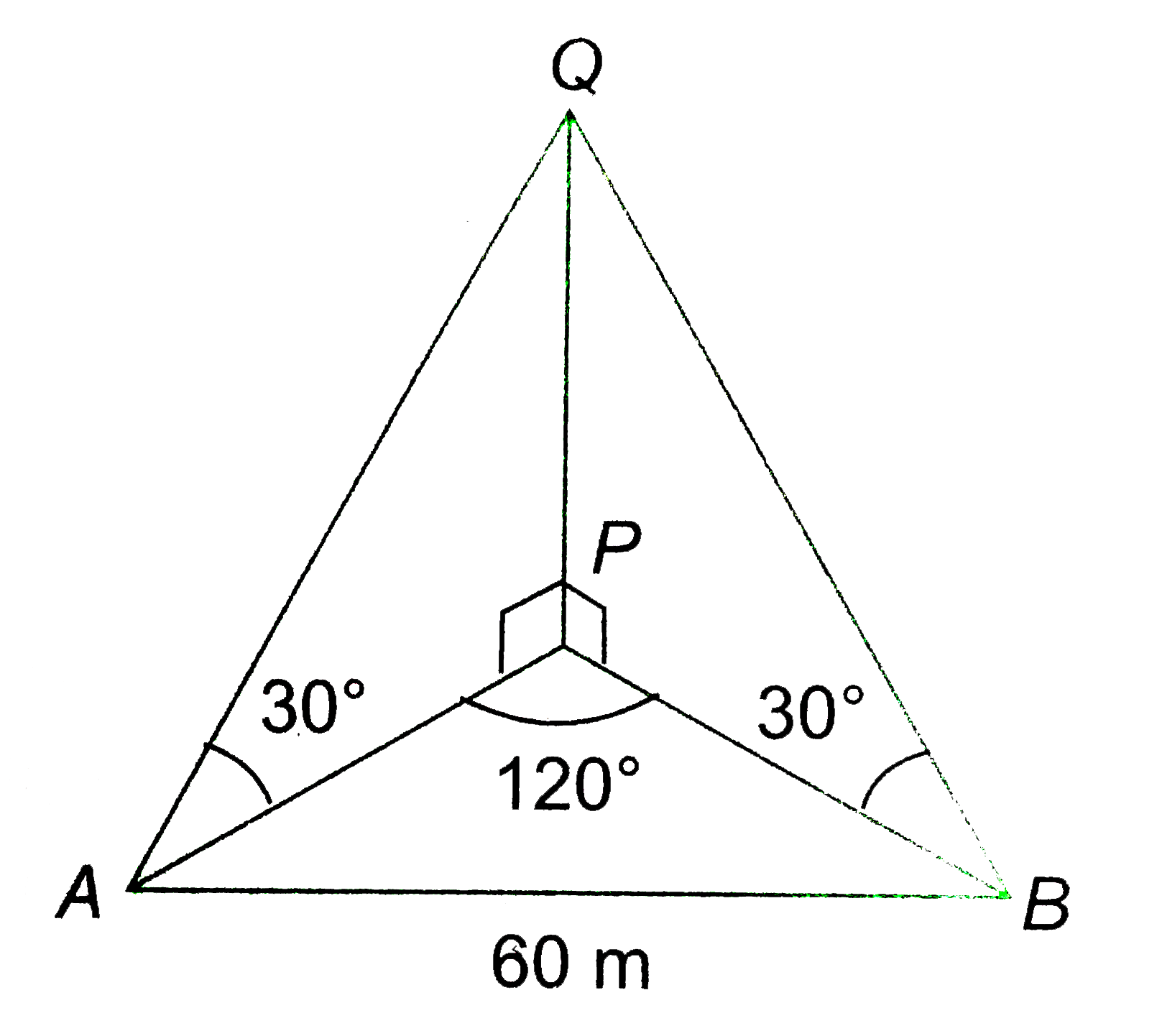
AP = PQ cot 30∘=√3PQ
In triangle BPQ we have
BP= PQ cot 30∘=√3PQ
In ΔAPB using cosine rule ,we get
cos120∘=AP2+BP2−AB22AP.BP
⇒−12=6PQ2−6022.3PQ2
⇒9PQ2=602
PQ=20m
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - HIGHT AND DISTANCE EXERCISE - Exercises18videos
HIGHT AND DISTANCE
- BOOK - CENGAGE PUBLICATIONCHAPTER - HIGHT AND DISTANCE EXERCISE - Archives3videos
HIGHT AND DISTANCE
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - HYPERBOLAEXERCISE - COMPREHENSION TYPE2videos
HYPERBOLA
Similar Questions
The tops of two poles of height 20 m and 14 m are connected by a wire. If the wire makes an angle of 30o with horizontal, then the length of the wire is (a) 12 m (b) 10 m (c) 8 m (d) 6 m
05:46View SolutionA bird is sitting on the top of a vertical pole 20 m high and its elevation from a point O on the ground is 45o . It flies off horizontally straight away from the point O. After one second, the elevation of the bird from O is reduced to 30o . Then the speed (in m/s) of the bird is (1) 40(√2−1) (2) 40(√3−2) (3) 20√2 (4) 20(√3−1)
04:37View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
05:35View SolutionThe circle x2+y2−8x=0 and hyperbola x29−y24=1 I intersect at the points A and B. Equation of a common tangent with positive slope to the circle as well as to the hyperbola is (A) 2x−√5y−20=0 (B) 2x−√5y+4=0 (C) 3x−4y+8=0 (D) 4x−3y+4=0
04:54View SolutionA vertical tower PQ subtends the same anlgle of 30∘ at each of two points A and B ,60 m apart on the ground .If AB subtends an angle of 120∘ at p the foot of the tower ,then find the height of the tower .
04:21View SolutionA tower of height b subtends an angle at a point 0 on the ground level through the foot of the tower and at a distance a from the foot of the tower. A pole mounted on the top of the tower also subtends an equal angle at 0. The height of the pole is
03:10View SolutionA vertical tower PQ subtends th same angle 30∘ at each of two place A and B, 60 m apart on the ground, AB subtends an angle 120∘ at the foot of the tower. If h is the height of the tower, then 9h2+h+1 is equal to
02:14View SolutionA tower stands at the centre of a circular park . A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60^@ at the foot of the tower , and the angle of elevation of the top of the tower from A or B is 30^@ . The height of the tower is
03:59View SolutionA vertical tower subtends an angle of 60∘ at a point on the same level as the foot of the tower. On moving 100 m further in line with the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower is
02:35View SolutionA tower stands at the centre of a circular park .A and B are two points on the boundary of the park such that AB = (=a) subtends an angle of 60^@ at the foot of the tower ,and the angle of elevation of the top of the tower from A or B is 30^@ the height of hte tower is
02:54View SolutionA vertical tower PQ subtends the same anlgle of 30∘ at each of two points A and B ,60 m apart on the ground .If AB subtends an angle of 120∘ at p the foot of the tower ,then find the height of the tower .
02:44View SolutionA tower of height b subtends an angle at a point 0 on the ground level through the foot of the tower and at a distance a from the foot of the tower. A pole mounted on the top of the tower also subtends an equal angle at 0. The height of the pole is
03:58View SolutionA Tower stands at the centre of a circular park. A and B are two points on the boundary of the park such that AB (a) subtends an angle of 60^(@) at the foot of the tower, and the angle of elevation of the top of the tower from A or B is 30°. The height of the tower is
07:28View SolutionA tower subtends an angle α at a point A on the same level as the foot of the tower B is a point vertically above A and AB = h metres. The angle of depression of the foot of the tower from B is β. The height of the tower is
06:52View SolutionA tower stands at the center of a circular park. A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60^(@) at the foot of the tower, and the angle of elevation of the top of the tower from A or B is 30^(@) . The height of the tower is
04:47View Solution

