Draw the graph of y=√x2−1 Solution in Bengali
Video Solution
Text Solution
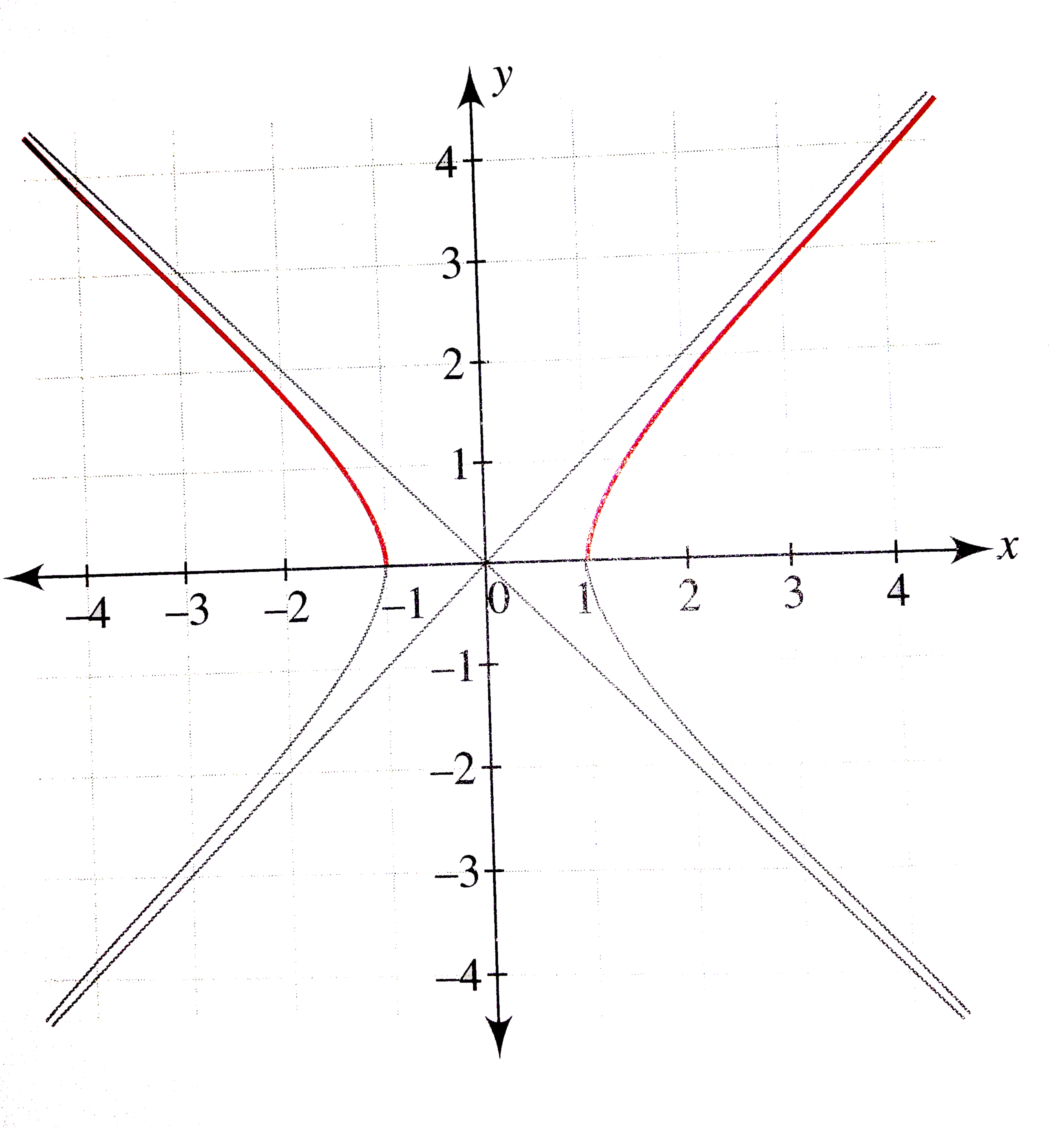
We have y=√x2−1
or y2=x2−1
or x2−y2=1, which is a rectangular hyperbola.
But y=√x2−1≥0
The graph of y=f(x) is part of the hyperbola x2−y2=1, which is lying above the x-axis.
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES34videos
GRAPHS OF ELEMENTARY FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises17videos
GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - GRAPHS OF TRIGONOMETRIC FUNCTIONSEXERCISE - Exercises22videos
GRAPHS OF TRIGONOMETRIC FUNCTIONS
Similar Questions
Draw the graph of y=1(1/x).
01:02View SolutionDraw the graph of y=(x−1)(x2−x+1).
01:15View SolutionDraw the graph of y= cosec 2x−cot2x. Is f(x) periodic ? If yes, what is its fundamental period ?
03:00View SolutionDraw the graph of y=√x2−1
03:58View SolutionDraw the graph of y=−√x2+2
01:56View SolutionDraw the graph of y=√xx−1
08:08View SolutionDraw the graph of the relation y=|x|√1−x2
04:19View SolutionDraw the graph of √|x|+√|y|=1
02:28View SolutionDraw the graph of y=loge(x+√x2+1)
02:30View SolutionDraw the graph of y=x2√x+1
02:16View SolutionDraw the graph of y=√x2−1
04:30View SolutionDraw the graph of y=−√x2+2
02:46View SolutionDraw the graph of y=√1+x2−x
02:02View SolutionDraw the graph of y=√xx−1
03:54View SolutionDraw the graph of √|x|+√|y|=1
02:48View Solution

