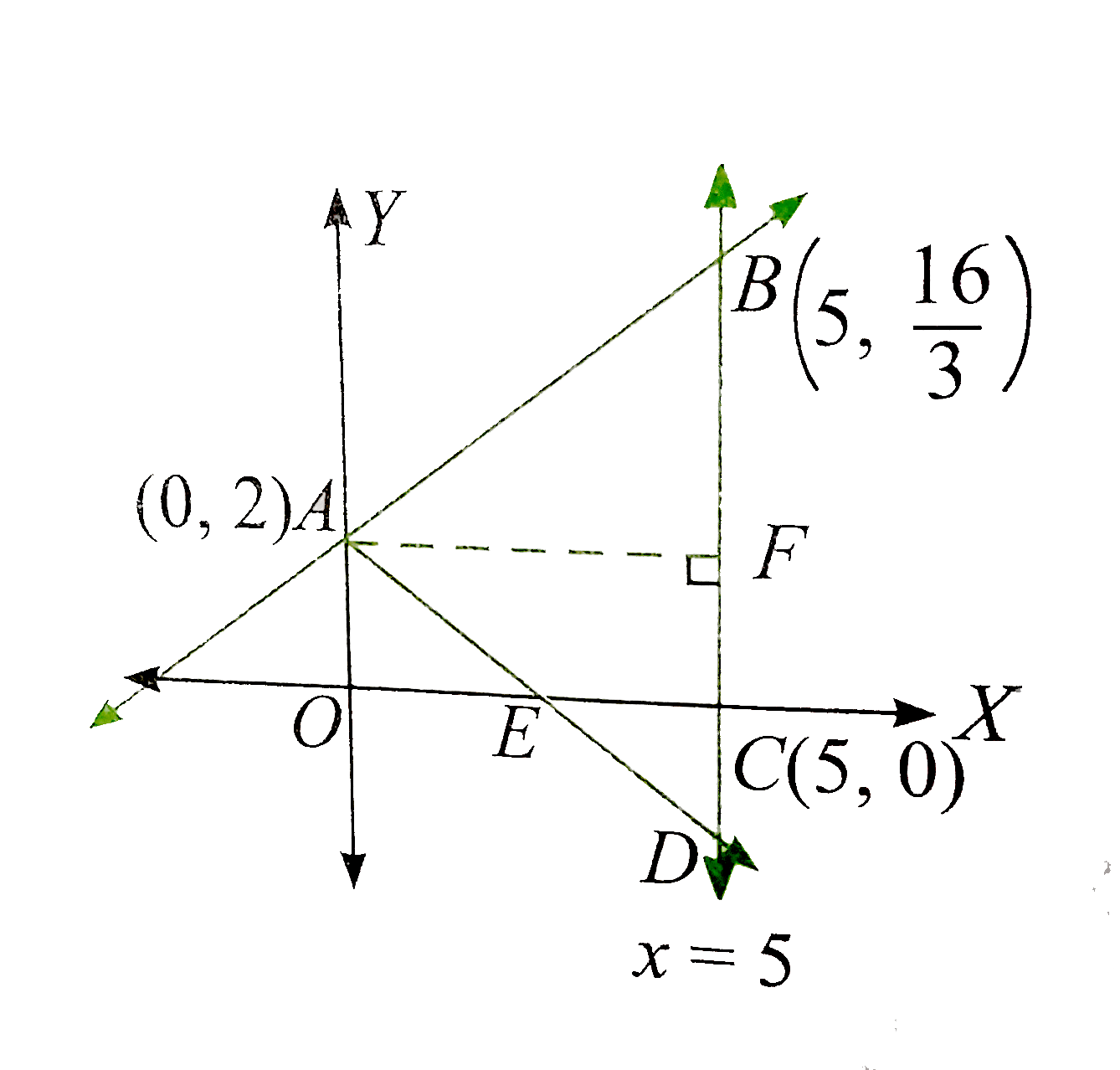
A line L1=3y−2x−6=0 is rotated about its point of intersection with the y-axis in the clockwise direction to make it L2 such that the are formed by L1,L2 the x-axis, and line x=5 is 493sq.units if its point of intersection with x=5 lies below the x-axis. Find the equation of L2.Solution in Bengali
The correct Answer is:x+y=2
L1=3y−2x−6=0
The point about which the line is rotated is A = (0,2). Let the equation of L2 be y = mx +2. As L2 will be cutting line x=5 below the x-axis, we have
A≡(0,2),B≡(5,163),C≡(5,0),
D≡(5,5m+2),E≡(−2m,0)
Now, Area of AECB= Area of ΔADB−Area of ΔECD
or 493=12BD×AF−12EC×CD
=12[163−(5m+2)]×5−12×(5+2m)[−(5m+2)]
or 493=110m+126mor m=−1
Hence, the equation of L2 is x+y=2.
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.24videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.37videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXAMPLE12videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
Two particles start from point (2, -1), one moving two units along the line x+ y = 1 and the other 5 units along the line x - 2y = 4, If the particle move towards increasing y, then their new positions are:
06:19View SolutionFind the points on y−ais whose perpendicular distance from the line 4x−3y−12=0 is 3.
04:38View SolutionShow that the lines 4x+y−9=0,x−2y+3=0,5x−y−6=0 make equal intercepts on any line of slope 2.
01:32View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
04:44View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
02:09View SolutionA line L1≡3y−2x−6=0 is rotated about its point of intersection with the y-axis in the clockwise direction to make it L2 such that the are formed by L1,L2 the x-axis, and line x=5 is 493sq.units if its point of intersection with x=5 lies below the x-axis. Find the equation of L2.
03:06View SolutionLet L_1 :x+y-3= 0 and L_2:7x -y +5 = 0 be two given lines. Let image of every point on L_1, with respect to a line L lies on L_2 then possible equation of L can be :
07:00View SolutionLines L1 and L2 have slopes m and n, respectively, suppose L1 makes twice as large angle with the horizontal (mesured counter clockwise from the positive x-axis as does L2 and L1 has 4 times the slope of L2. If L1 is not horizontal, then the value of the proudct mn equals.
02:47View SolutionLet L1 be a tangent to the parabola y2=4(x+1)andL2 be a tangent to the parabola y2=8(x+2) such that L1andL2 intersect at right angles. Then L1andL2 meet on the straight line :
02:05View SolutionA line makes zero intersects on x axis and y axis and it is prependicular to the line 3x+4y+6=0 then its equation is
03:37View SolutionConsider the lines given by L1:x+3y−5=0 L2:3x−ky−1=0 L3:5x+2y−12=0 Column I|Column II L1,L2,L3 are concurrent if|p. k=−9 One of L1,L2,L3 is parallel to at least one of the other two if|q. k=−65 L1,L2,L3 form a triangle if|r. k=56 L1,L2,L3 do not form a triangle if|s. k=5
04:30View SolutionA line L1≡3y−2x−6=0 is rotated about its point of intersection with the y-axis in the clockwise direction to make it L2 such that the are formed by L1,L2 the x-axis, and line x=5 is 493sq.units if its point of intersection with x=5 lies below the x-axis. Find the equation of L2.
08:00View Solutionθ1andθ2 are the inclination of lines L1andL2 with the x-axis. If L1andL2 pass through P(x1,y1), then the equation of one of the angle bisector of these lines is
05:56View SolutionL1andL2 are two intersecting lines. If the image of L1 w.r.t. L2 and that of L2 w.r.t. L1 concide, then the angle between L1andL2 is
07:46View SolutionConsider two lines L1:2x+y=4 and
L2:(2x−y=2)
Find the equation of the line passing through the intersection of L1 and L2 which makes an angle 45° with the positive direction of x−aξs.03:37View SolutionConsider two lines L1:2x+y=4 and
L2:(2x−y=2)
Find the equation of the line passing through the intersection of L1 and L2 which makes an angle 45° with the positive direction of x−aξs.
Find the x and y intercepts of the third line obtained.09:23View Solution

