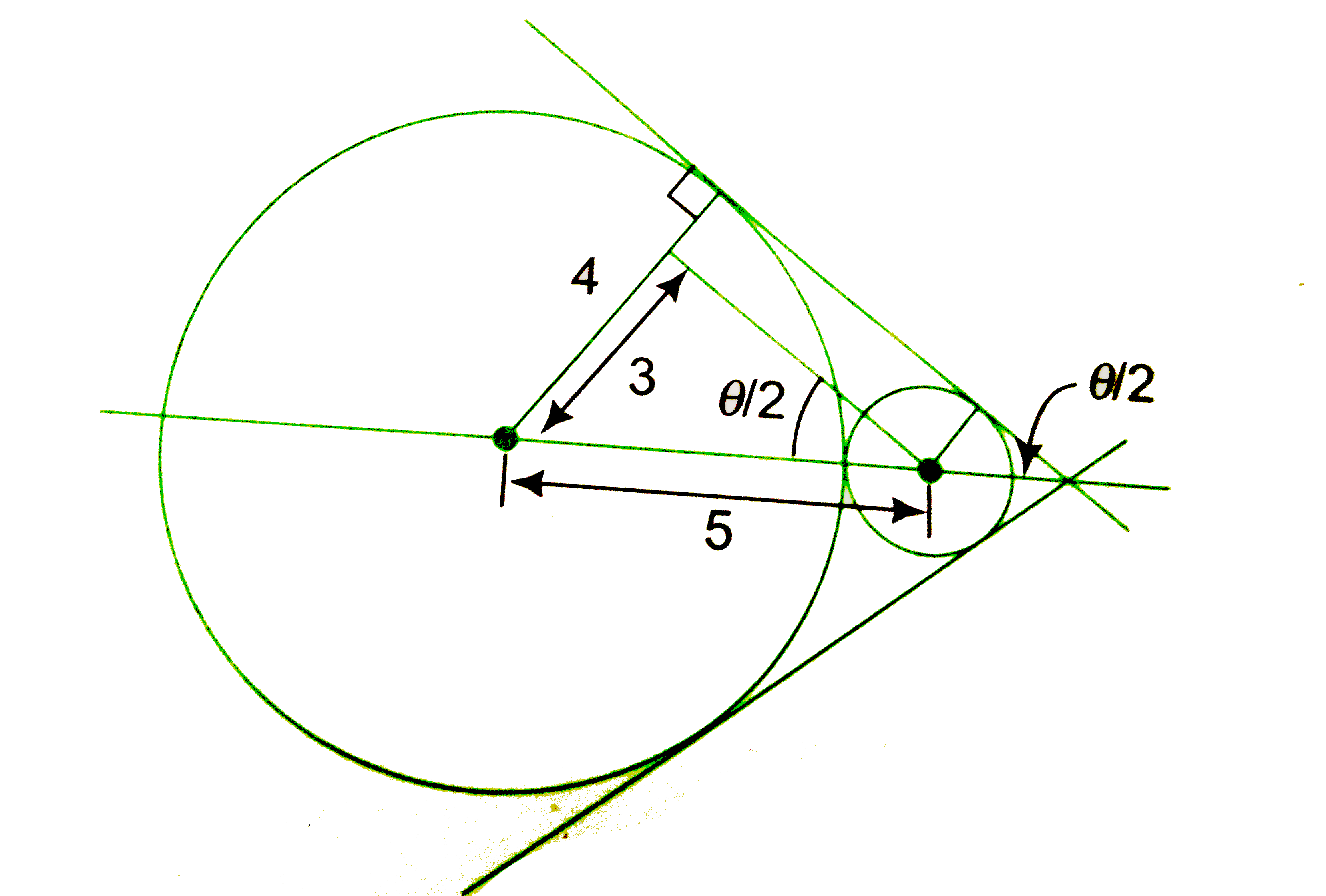
Two circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Find sin(θ2)+cos(θ2).Solution in Bengali
The correct Answer is:7/5
From figure, we have
sin(θ)2=35
cos(θ)2=45
∴Sin(θ)2+cos(θ)2=75
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Concept Application Exercises 2.28videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Concept Application Exercises 2.34videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - example10videos
TRIGONOMETRIC FUNCTIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)4videos
TRIGONOMETRIC EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Archives (Numerical Value Type)2videos
TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULAS
Similar Questions
Prove that sinx−cosx+1sinx+cosx−1=secx+tanx
03:43View SolutionA horse is tied to a post by a rope. If the horse moves along a circular path always keeping the rope tight, and describes 88 metres when it traces 72∘ at the centre, find the length of the rope.
03:43View SolutionIf 2cosx+sinx=1, then find the valie of 7x+6sinx.
04:05View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
02:26View SolutionThe maximum value of the expression 1sin2θ+3sinθcosθ+5cos2θ is………
03:52View SolutionTwo circles with radii a and b touch each other externally such that theta is the angle between their direct common tangent (a>b>=2), then
00:48View SolutionTwo circles of radii a and b touch each other externally and theta be the angle between their direct common tangents (a>b>=2) then
04:05View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Find sinθ2+cosθ2.
05:46View SolutionTwo circles with radii aandb touch each other externally such that θ is the angle between the direct common tangents, (a>b≥2) . Then prove that θ=2sin−1(a−ba+b) .
04:31View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Then sinθ is equal to 2425 (b) 1225 34 (d) none of these
04:01View SolutionIf two circles of radius 4cm and 1cm touch each other externally then length of the direct common tangents is
02:58View SolutionTwo circles of radii 5cm and 2cm touch each other externally and θ is the angle contained by their direct common tangents.Then sinθ is equal to 12√10λ where λ equals
04:53View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Find sin(θ2)+cos(θ2).
03:33View SolutionTwo circles with radii aandb touch each other externally such that θ is the angle between the direct common tangents, (a>b≥2) . Then prove that θ=2sin−1(a−ba+b) .
03:27View SolutionTwo circles with radii aandb touch each other externally such that θ is the angle between the direct common tangents, (a>b≥2) . Then prove that θ=2sin−1(a−ba+b) .
04:22View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Then sinθ is equal to (a)2425 (b) 1225 (c)34 (d) none of these
06:35View Solution

