Two equal circles of largest radii have following property:
(i) They intersect each other orthogonally,
(ii) They touch both the curves $4 (y + 2) = x^{2}$ and $4 (2 - y) = x^{2}$ in the region $x \in [- 2 \sqrt{2}, 2 \sqrt{2}]$ . Then radius of this circle is

A

$\sqrt{2}$

B

$\sqrt{3}$

C

$\frac{1}{\sqrt{3}}$

D

$\frac{3}{2}$

Text Solution

Verified by Experts

The correct Answer is:A

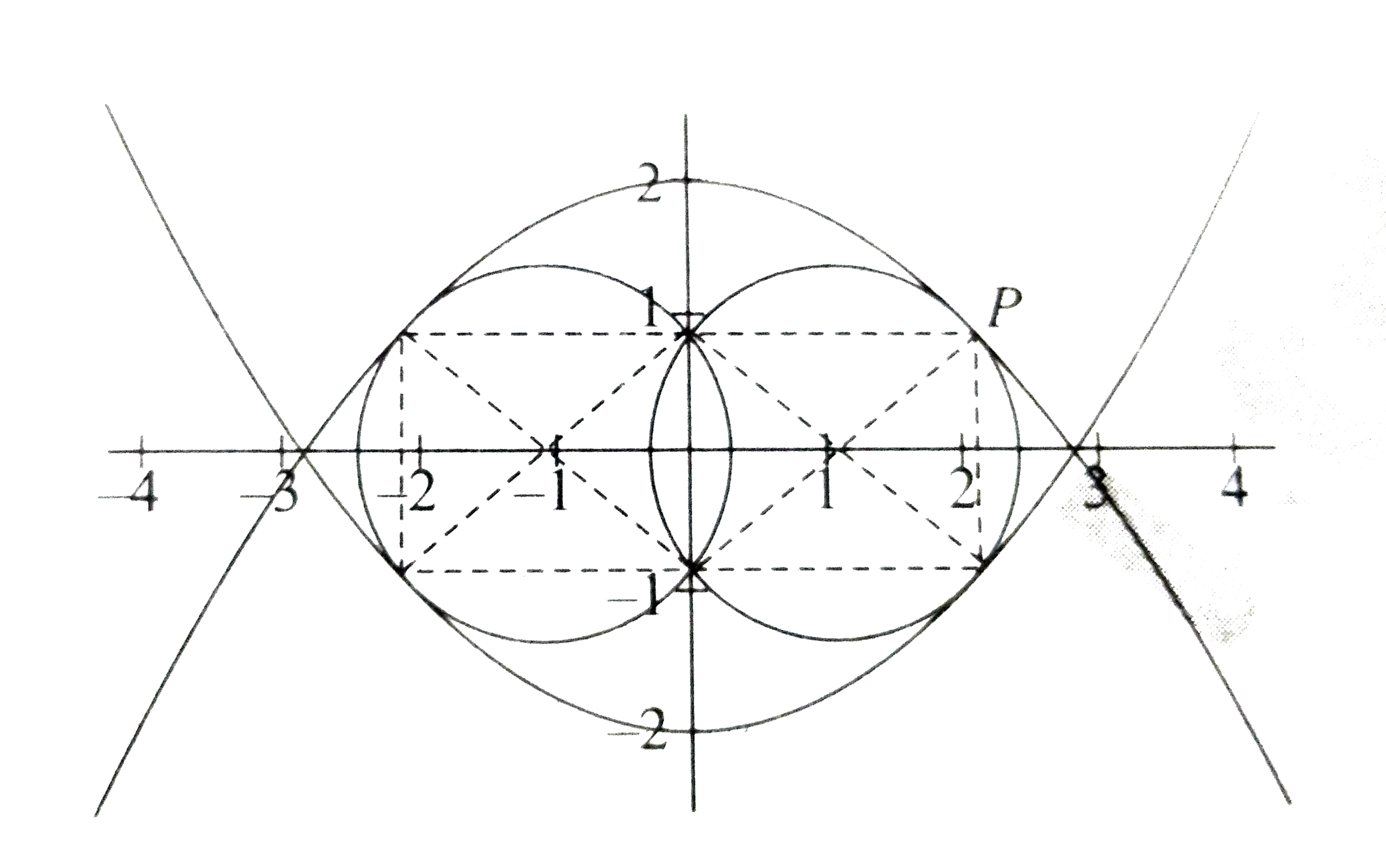
Let the radius of circle is r.
From geometry, $P \equiv (r \sqrt{2}, \frac{r}{\sqrt{2}})$ .
Now, this point lies on the curve $4 (2 - y) = x^{2}$
$\Rightarrow 4 (2 - \frac{r}{\sqrt{2}}) = {(r \sqrt{2})}^{2}$
$\Rightarrow r = \sqrt{2}$

|

Updated on:21/07/2023

Class 12 MATHS PARABOLA

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If the line ax + y = c, touchs both the curves $x^{2} + y^{2} = 1$
and $y^{2} = 4 \sqrt{2} x$ , then |c| is equal to
A $\frac{1}{\sqrt{2}}$
B2
C $\sqrt{2}$
D $\frac{1}{2}$
Question 2 - Select One
If the line $a x + y = c$ , touches both the curves $x^{2} + y^{2} = 1 and y^{2} = 4 \sqrt{2} x$ , then $| c |$ is equal to:
A $\frac{1}{2}$
B $\sqrt{2}$
C2
D $\frac{1}{\sqrt{2}}$
Question 3 - Select One
The two circles $x^{2} + y^{2} - c x = 0 and x^{2} + y^{2} = 4$ touch each other if:
A $| c | = 4$
B $| c | = 2$
C $| c | = 8$
DNone of these

CENGAGE PUBLICATION-PARABOLA-Single Correct Answer Type

Home
Ask Doubt
Profile