Consider equation $(x - sin α) (x - cos α) - 2 = 0$ . Which of the following is /are true?

A

If $0 < α < \frac{π}{4}$ , then the equation has both roots in $(sin α, cos α)$

B

If $\frac{π}{4} < α \frac{π}{2}$ , then the equations has both roots in $(sin α, cos α \infty)$

C

If $0 < α < \frac{π}{4}$ , the one roots lies in $(- \infty, sin α)$ and the other in $(sin α, \infty)$

D

If $\frac{π}{4} < α < \frac{π}{2}$ then one root lies in $(- \infty, cos α)$ and the other is $(sin α, \infty)$

Text Solution

Verified by Experts

The correct Answer is:3,4

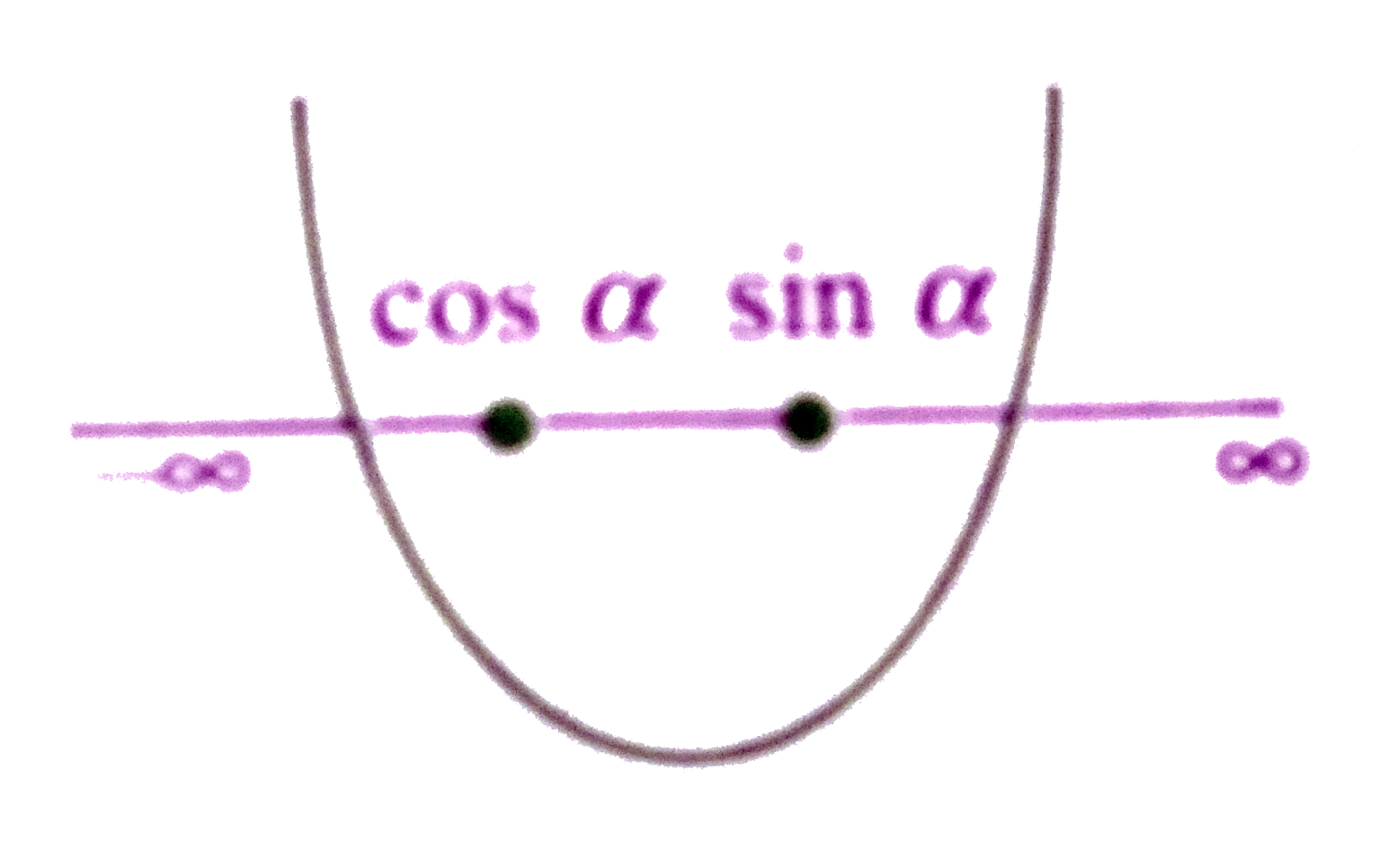
Let , $f (x) = (x - sin α) (x - cos α) - 2$
Then. $f (sin α) = - 2 < 0$ and
$f (cos α) = - 2 < 0$ and
$f (cos α) = - 2 < 0$
So, sin $α and cos α$ lie between the
roots

|

Updated on:21/07/2023

Class 12 MATHS QUADRATIC EQUATIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
If 1 lies between the roots of the equation $3 x^{2} - 3 sin α x - 2 {cos}^{2} α = 0$ , the $α$ lies in the interval
A $(0, \frac{π}{2})$
B $(\frac{π}{12}, \frac{π}{2})$
C $(\frac{π}{6}, \frac{5 π}{6})$
D $(\frac{π}{6}, \frac{π}{2}) \cup (\frac{π}{2}, \frac{5 π}{6})$
Question 2 - Select One
Let $f (x) = {sin}^{2} (x + α) + {sin}^{2} (x + β) - 2 cos (α - β) sin (x + α) sin (x + β)$ . Which of the following is TRUE ?
Af(x) is strictly increasing in $x \in (α, β)$
Bf(x) is strictly decreasing in $x \in (α, β)$
Cf(x) is strictly increasing in $x \in (α, \frac{α + β}{2})$ and strictly decreasing in $x \in (\frac{α + β}{2}, β)$
Df(x) is a constant function
Question 3 - Select One
Let $sin α, cos α$ be the roots of the equation $x^{2} - b x + c = 0$ . Then which of the following statements is/are correct?
A $c \leq \frac{1}{2}$
B $b \leq \sqrt{2}$
C $c > \frac{1}{2}$
D $b > \sqrt{2}$

CENGAGE PUBLICATION-THEORY OF EQUATIONS-Multiple Correct Answer Type

Home
Ask Doubt
Profile