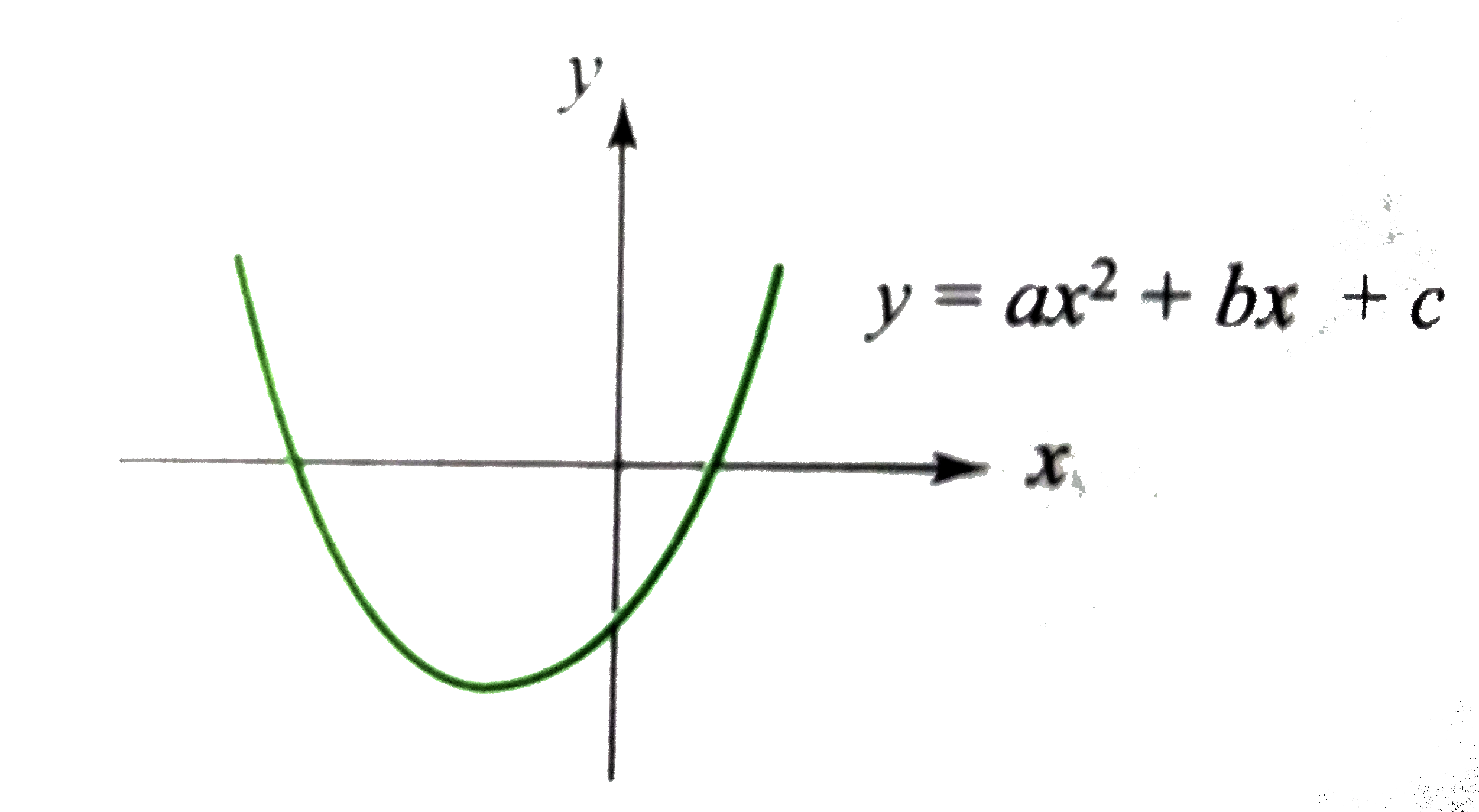
Let f(X)=ax2+bx+c. Consider the following diagram
 .
.
A
c<0
B
b>0
C
a+b−c>0
D
abc<0
Text Solution
The correct Answer is:1,2,3,4
Let f(x)=ax2+bx+c
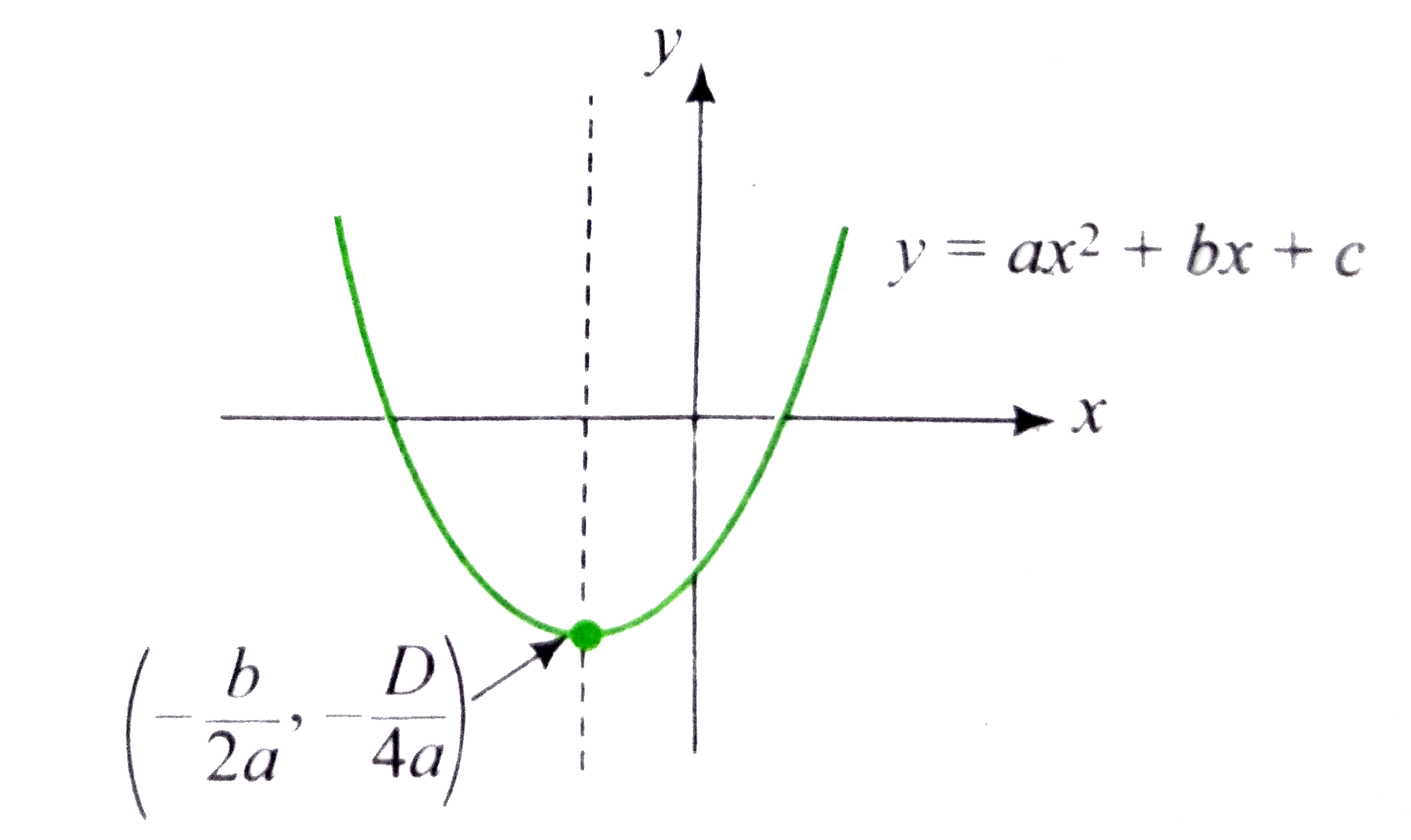
For the diagram, we can see that a>0,c<0and−[b(2a)]<0 .
Hence , b>0
∴a+b−c>0
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - Linked Comprechension Type37videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - MATRIX MATCH TYPE6videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - Single Correct Answer Type : Exercise89videos
THEORY OF EQUATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE)1videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions291videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
Knowledge Check
- Question 1 - Select One
If f(x)=ax2+bx+c find f1(1).
Aa+2bB2a−bCa−2bD2a+b - Question 2 - Select One
Consider that f(x)=ax2+bx+c,D=b2−4ac , then which of the following is not true ?
AIf a>0 then minimum value of f(x) is −D4aBIf a<0, then maximum value of f(x) is −D4aCif a>0,D<0, then f(x)>0 for all x ∈ RDIf a>0,D>0 , then f(x)>0 for alll x∈R - Question 3 - Select One
Let f(x)=ax2−bx+c2,b≠0andf(x)≠0 for all x∈R. Then
Aa+c2<bB4a+c2>2bC9a−3b+c2<0Dnone of these
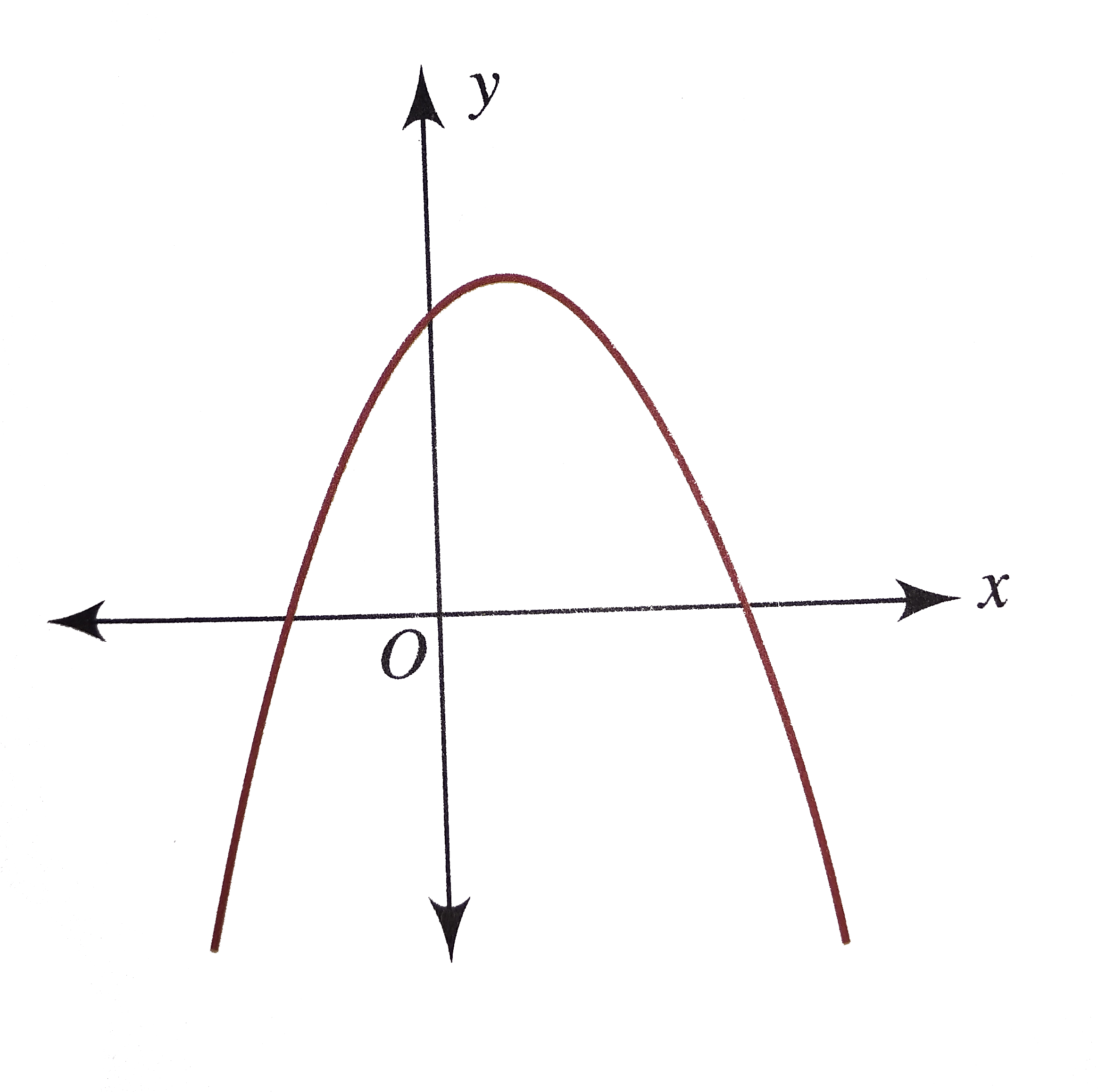
The following figure shows the graph of f(x)=ax2+bx+c, find the signs of a,bandc.
 View Solution
View SolutionConsider that f(x)=ax2+bx+c,D=b2−4ac , then which of the following is not true ?
View SolutionLet f(x)=ax2−bx+c2,b≠0andf(x)≠0 for all x∈R. Then
View SolutionThe following figure shows the graph of f(x)=ax2+bx+c, then find the sign of values of a,bandc.
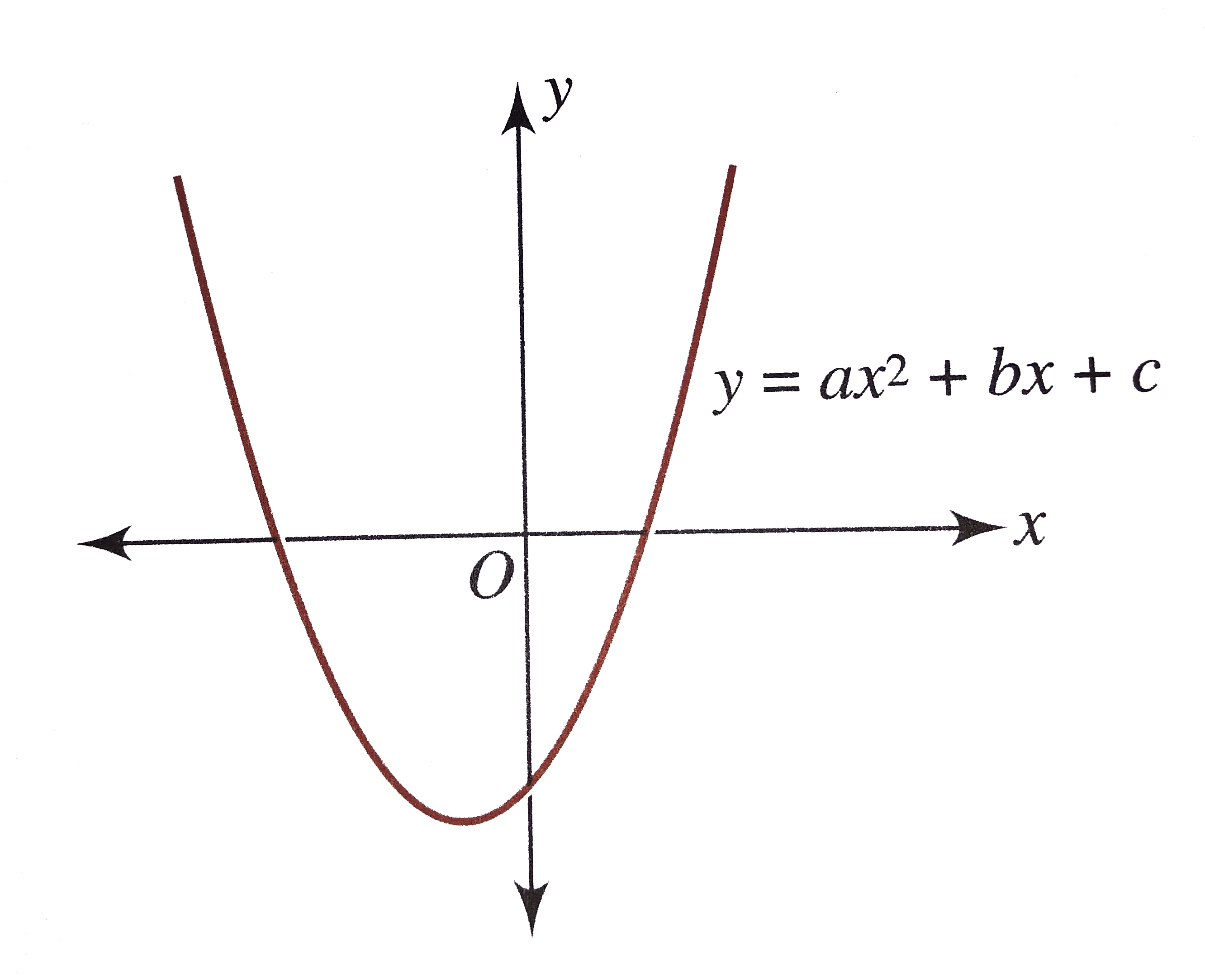 View Solution
View SolutionLet f(x)=ax2+bx+c such that f(1)=f(−1) and a,b,c are in Arithmetic Progression (AP).
Q. What is the value of b?View Solution

