A hemi-spherical tank of radius 2 m is initially full of water and has an outlet of 12cm2 cross-sectional area at the bottom. The outlet is opened at some instant. The flow through the outlet is according to the law v(t)=0.6√2gh(t), where v(t) and h(t) are, respectively, the velocity of the flow through the outlet and the height of water level above the outlet and the height of water level above the outlet at time t, and g is the acceleration due to gravity. Find the time it takes to empty the tank.Solution in Bengali
Let the water level be at a height h after time t, and water level falls by dh in time dt, and the corresponding volume of water gone out be dV.
∴dV=−πr2dh
∴dVdt=−πr2dhdt ∴ as t increases, h decreases
Now, velocity of water, v=35√2gh
Rate of flow of water =Av (A=12cm2)
∴dVdt=(35√2ghA)
=−πr2dhdt

Also from the figure,
R2=(R−h)2+r2
or r2=2hr−h2
So, 35√2g√hA=−π(2hR−h2)×dhdt
or 2hR−h2√hdh=−35π√2hAdt
Integrating, we get
∫0R(2R√h−h3/2)dh=−3√2g5πA.∫t0dt
or T=−5π3A√2g(2Rh3/23/2−h5/25/2)R0
=5π3A√2g(4R3R3/2−25R5/2)
=5π3A√2g1415R5/2
For R=200cm,
T=56π9A√g(10)5
=56π9×12√g(10)5
=14π27√g(10)5 units
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type11videos
DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS
- BOOK - CENGAGE PUBLICATIONCHAPTER - DIFFERENTIATIONEXERCISE - Archives14videos
DIFFERENTIATION
Similar Questions
Let →a=a1ˆi+a2ˆj+a3ˆk,→b=b1ˆi+b2ˆj+b3ˆkand→c=c1ˆi+c2ˆj+c3ˆk be three non-zero vectors such that →c is a unit vector perpendicular to both →aand→b. If the angle between →aand→bisπ/6 then the value of ∣∣ ∣∣a1b1c1a2b2c2a3b3c3∣∣ ∣∣is
04:23View SolutionLet y be an implicit function of x defined by x2x−2xxcoty−1=0. Then y'(1) equals: a.1 b. log2 c. −log2 d. −1
12:13View SolutionFind the time required for a cylindrical tank of radius r and height H to empty through a round hole of area a at the bottom. The flow through the hole is according to the law v(t)=k√2gh(t) , where v(t) and h(t) , are respectively, the velocity of flow through the hole and the height of the water level above the hole at time t, and g is the acceleration due to gravity.
02:54View SolutionA hemi-spherical tank of radius 2 m is initially full of water and has an outlet of 12cm2 cross-sectional area at the bottom. The outlet is opened at some instant. The flow through the outlet is according to the law v(t)=√0.62gh(t), where v(t) and h(t) are, respectively, the velocity of the flow through the outlet and the height of water level above the outlet and the height of water level above the outlet at time t, and g is the acceleration due to gravity. Find the time it takes to empty the tank.
View SolutionWater filled in an empty tank of area 10 A through a tap of cross sectional area A. The speed of water flowing out of tap is given by v(m/s) =10(1-sin((pi)/(30)t) where t is in second. The height of water level from the bottom of the tank at t=15 second will be:
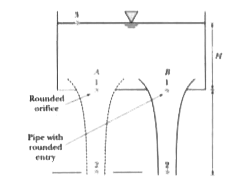
05:42View SolutionA tank has two outlets (i) a rounded orifice A of diameter D and (ii) a pipe B with well - rounded entry and of length L , as shown in Fig. For a height of water H in the tank , determine the (a) discharge from the outlets A and B , (b) velocities in the two outlets at levels 1 and 2 indicated in Fig .
 05:48View Solution
05:48View SolutionThe intake in Figure, Reservoir has cross-sectional area of 0.740 m^(2) and water flows in at 0.400 m/s. At the outlet, the cross-sectional area is smaller than at the intake and the water flows out at 9.50 m/s. The pressure difference between intake and outlet is 3.00 MPa. What is vertical distance D?
13:22View SolutionFind the time required for a cylindrical tank of radius r and height H to empty through a round hole of area a at the bottom. The flow through the hole is according to the law v(t)=k√2gh(t) , where v(t) and h(t) , are respectively, the velocity of flow through the hole and the height of the water level above the hole at time t, and g is the acceleration due to gravity.
04:04View SolutionA hemi-spherical tank of radius 2 m is initially full of water and has an outlet of 12cm2 cross-sectional area at the bottom. The outlet is opened at some instant. The flow through the outlet is according to the law v(t)=0.6√2gh(t), where v(t) and h(t) are, respectively, the velocity of the flow through the outlet and the height of water level above the outlet and the height of water level above the outlet at time t, and g is the acceleration due to gravity. Find the time it takes to empty the tank.
08:38View SolutionFind the time required for a cylindrical tank of radius r and height H to empty through a round hole of area a at the bottom. The flow through the hole is according to the law v(t)=k√2gh(t) , where v(t) and h(t) , are respectively, the velocity of flow through the hole and the height of the water level above the hole at time t, and g is the acceleration due to gravity.
02:17View Solution2 मीटर त्रिज्या का एक अंगोलाकार टैंक पानी से पूर्ण भरा हुआ है। जिसके तल भाग में 2 वर्ग सेमी अनुप्रस्थ काट वाली एक टोंटी लगी है। टोंटी को कुछ समय के लिए खोल दिया जाता है। टोंटी से निकलने वाले पानी का रिसाव V(t)=0.6√2gh(t) नियम के अनुसार है, जहाँV(t) तथा h(t) समय t पर क्रमशः टोंटी से निकलने वाले पानी का वेग तथा टोंटी से पानी के तल की ऊँचाई है तथा g गुरुत्वाकर्षण के कारण त्वरण है। टैंक के खाली होने में लगा समय ज्ञात कीजिए।
05:35View SolutionWater filled in an empty tank of area 10 A through a tap of cross sectional area A. The speed of water flowing out of tap is given by v(m/s) =10(1-sin((pi)/(30)t) where t is in second. The height of water level from the bottom of the tank at t=15 second will be:
12:00View SolutionFind the time required for a cylindrical tank of radius r and height H to empty through a round hole of area a at the bottom. The flow through the hole is according to the law v(t)=k√2gh(t) , where v(t) and h(t) , are respectively, the velocity of flow through the hole and the height of the water level above the hole at time t, and g is the acceleration due to gravity.
01:09View SolutionA hemi-spherical tank of radius 2 m is initially full of water and has an outlet of 12cm2 cross-sectional area at the bottom. The outlet is opened at some instant. The flow through the outlet is according to the law v(t)=0.6√2gh(t), where v(t) and h(t) are, respectively, the velocity of the flow through the outlet and the height of water level above the outlet and the height of water level above the outlet at time t, and g is the acceleration due to gravity. Find the time it takes to empty the tank.
05:17View SolutionFind the time required for a cylindrical tank of radius r and height H to empty through a round hole of area a at the bottom. The flow through the hole is according to the law v(t)=k√2gh(t) , where v(t) and h(t) , are respectively, the velocity of flow through the hole and the height of the water level above the hole at time t, and g is the acceleration due to gravity.
03:57View SolutionA hemi-spherical tank of radius 2 m is initially full of water and has an outlet of 12cm2 cross-sectional area at the bottom. The outlet is opened at some instant. The flow through the outlet is according to the law v(t)=0.6√2gh(t), where v(t) and h(t) are, respectively, the velocity of the flow through the outlet and the height of water level above the outlet and the height of water level above the outlet at time t, and g is the acceleration due to gravity. Find the time it takes to empty the tank.
04:30View Solution

