Show that $f (x) = {\begin{matrix} x sin \frac{1}{x}, & when & x \neq 0 0, & when & x = 0 \end{matrix}$ is continuous but not differentiable at x = 0 Solution in Punjabi

$lim x \to 0 f (x)$ exists for every $n > 1$

f is continuous at x = 0 for $n > 1$

f is differentiable at x= 0 for every $n > 1$

None of the above

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, C

Updated on:21/07/2023

Answer

Step by step video & image solution for Show that f(x)={{:("x sin"(1)/(x)",","when",x ne 0),(0",","when",x = 0):} is continuous but not differentiable at x = 0 by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.

Updated on:21/07/2023

Class 12 MATHS CONTINUITY AND DIFFERENTIABILITY

Topper's Solved these Questions

CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise (Statement I And Ii Type Questions)
11 Videos
CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - EXERCISE 4
3 Videos
CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise (Single Option Correct Type Questions)
50 Videos
COMPLEX NUMBERSBOOK - ARIHANT MATHSCHAPTER - COMPLEX NUMBERSEXERCISE - Complex Number Exercise 8
3 Videos
COORDINATE SYSTEM AND COORDINATES BOOK - ARIHANT MATHSCHAPTER - COORDINATE SYSTEM AND COORDINATES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
7 Videos

Similar Questions

Statement I $f (x) = sin x + [x]$ is discontinuous at x = 0.
Statement II If g(x) is continuous and f(x) is discontinuous, then g(x) + f(x) will necessarily be discontinuous at x = a.
View Solution
A man leaves his home early in the morning to have a walk. He arrives at a junction of roads A and B as shown in figure. He takes the following steps in later journies :

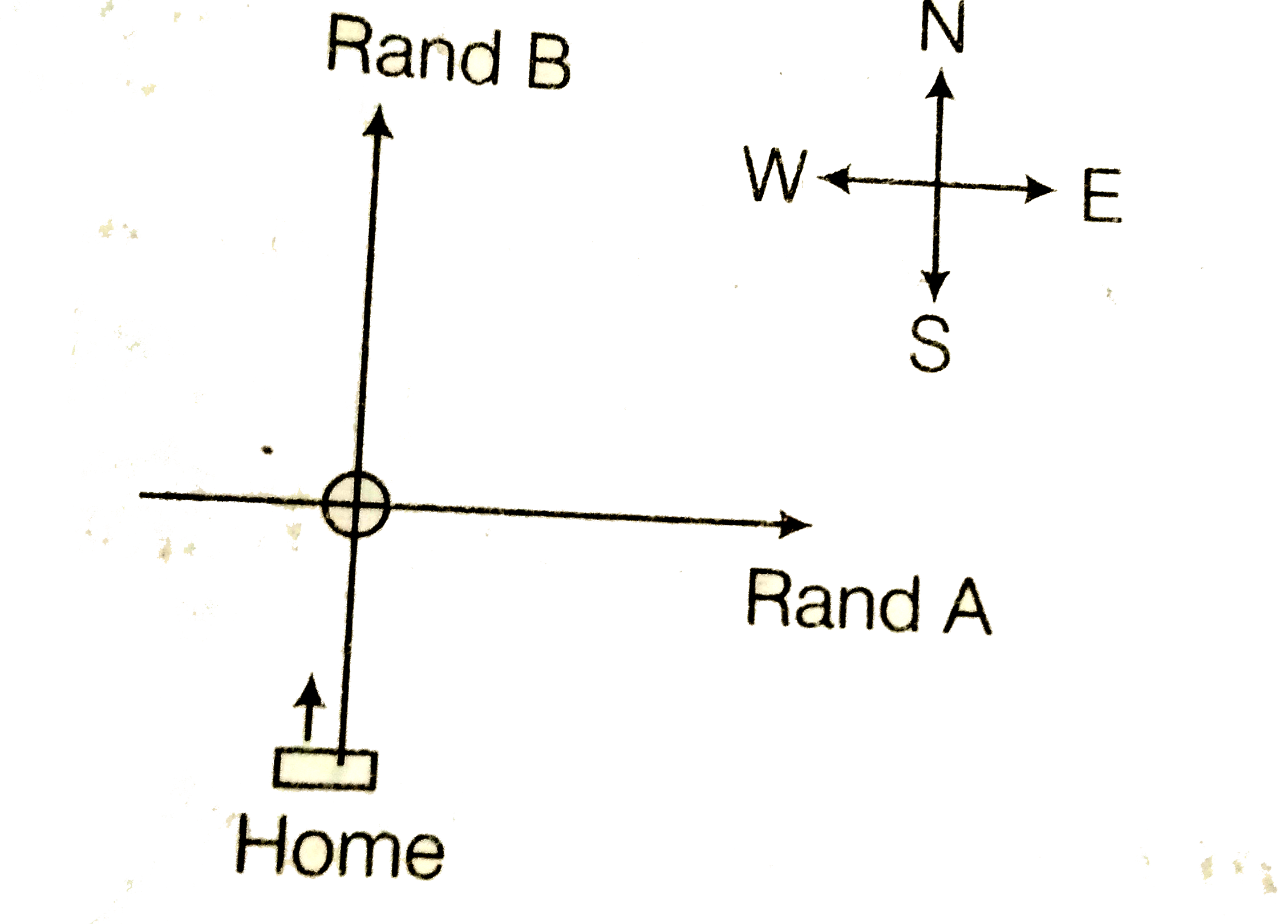
(i) 1 km in North direction.
(ii) Changes direction and moves in North-East direction for $2 \sqrt{2}$ km.
(iii) Changes direction and moves Southwards for distance of 2 km.
(iv) Finally he changes the direction and moves in South-East direction to reach road A again.
Now if roads A and B are taken as X-axis and y-axis ,then visible point representing the graph of y=f(x)
Then the value of x at which function f(x) is discontinous
View Solution
If $f (x) = {\begin{matrix} sin \frac{π x}{2}, & x < 1 [x], & x \geq 1 \end{matrix}$ , where [x] denotes the greatest integer function, then
View Solution
Find the modules of $2 + 3 ι$
View Solution
If a vertex of a triangle is (1, 1) and the mid-points of two side through this vertex are (-1, 2) and (3, 2), then centroid of the triangle is
View Solution
Show that the function f(x)={x sin((1)/(x)) when x!=0;=0, when x=0 is continuous butnot differentiable at x=0
View Solution
Let $f (x) = {\begin{matrix} x^{n} sin \frac{1}{x} & x \neq 0 0 & x = 0 \end{matrix}$ Then f(x) is continuous but not differentiable at x=0. If
View Solution
If $f (x) = ⎧ ⎨ ⎩ \begin{matrix} x^{k} sin (\frac{1}{x}), & x \neq 0 0, & x = 0 \end{matrix}$ is continuous at x = 0, then
View Solution
Show that $f (x) = {\begin{matrix} x sin \frac{1}{x}, & when & x \neq 0 0, & when & x = 0 \end{matrix}$ is continuous but not differentiable at x = 0
View Solution
If $f (x) = {\begin{matrix} x sin \frac{1}{x} & when x \neq 0 0 & when x = 0 \end{matrix}$
then at x = 0, the function f(x) is -
View Solution
দেখাও যে, $f (x) = {x cos (\frac{1}{x})$ যখন $x \neq 0$
$0$ যখন $x = 0$
অপেক্ষক $x = 0$ বিন্দুতে সন্তত
View Solution
Show that the function $f (x)$ given by $f (x) = {x \frac{sin 1}{x}, x \neq 00, x = 0$
View Solution
Test differentiability and continuity of the following functions.
$f (x) = {\begin{matrix} x sin (\frac{1}{x}) & x \neq 0 0 & x = 0 \end{matrix} a t x = 0 .$
View Solution
Show that $f (x) = x sin (\frac{1}{x}), x \neq 0, f (0) = 0$ is continuous at x = 0
View Solution
Show that the function $f (x) = x^{p} sin (\frac{1}{x}) x, \neq 0, f (0) = 0$ is continuous but not differentiable at $x = 0$ if $0 < p \leq 1 .$
View Solution
Show that the functions $f (x) = x^{3} sin (\frac{1}{x})$ for $x \neq 0, f (0) = 0$ is differentiable at $x = 0 .$
View Solution

ARIHANT MATHS-CONTINUITY AND DIFFERENTIABILITY-Exercise (More Than One Correct Option Type Questions)

If f(x) = |x+1|(|x|+|x-1|), then at what point(s) is the function not ...
04:35
Let [x] be the greatest integer function f(x)=(sin(1/4(pi[x]))/([x])) ...
02:33
If f(x) = {{:((sin^(-1)x)^(2)cos((1)/(x))",",x ne 0),(0",",x = 0):} th...
04:59
f(x) = cos x and H(x) = {{:(min{f(t), 0 le t lt x},0 le x le (pi)/(2))...
02:16
If f(x) = 3(2x + 3)^(2//3) + 2x + 3, then: (a) f(x) is continuous but ...
05:06
If f(x) ={{:(-x=(pi)/(2),xle -(pi)/(2)), (- cos x, -(pi)/(2)lt x ,le ...
05:19
if f(x) ={{:( (x log cos x)/( log( 1+x^(2) )), x ne 0) ,( 0, x=0):} ...
05:50
Let [x] denote the greatest integer less that or equal to x. If f(x) =...
04:35
The function f(x)=x-[x] , where [⋅] denotes the greatest integer fu...
02:54
The function f(x)=sqrt(1-sqrt(1-x^2)) a. has its domain -1 le x le 1 ...
03:19
Consider the function f(x) = |x^(3) + 1|. Then,
04:12
f is a continuous function in [a,b]; g is a continuous function in [b,...
02:42
Which of the following function(s) has/have the same range ?
04:41
If f(x) = sec 2x + cosec 2x, then f(x) is discontinuous at all points ...
03:39
Show that f(x)={{:("x sin"(1)/(x)",","when",x ne 0),(0",","when",x = 0...
04:16
A function is defined as f(x) = {{:(e^(x)",",x le 0),(|x-1|",",x gt 0)...
04:49
Let f(x) = int(-2)^(x)|t + 1|dt, then a. f(x) is continuous in [-1, ...
03:08
A function f(x) satisfies the relation f(x+y) = f(x) + f(y) + xy(x+y),...
06:14
Show that the function f (x)={ {:(3x^(2) + 12 x - 1,- 1 le x le 2 )...
10:11
If f(x) = 0 for x lt 0 and f(x) is differentiable at x = 0, then for x...
05:29

Home
Ask Doubt
Profile

Show that f(x)={x sin1x,whenx≠00,whenx=0 is continuous but not differentiable at x = 0 Solution in Punjabi

The correct Answer is:A, B, C

Topper's Solved these Questions

Similar Questions

ARIHANT MATHS-CONTINUITY AND DIFFERENTIABILITY-Exercise (More Than One Correct Option Type Questions)

Show that $f (x) = {\begin{matrix} x sin \frac{1}{x}, & when & x \neq 0 0, & when & x = 0 \end{matrix}$ is continuous but not differentiable at x = 0 Solution in Punjabi