If a tower subtends angles θ,2θand3θ at three points A,B,and C respectively, lying on the same side of a horizontal line through the foot of the tower, show that ABBCcotθ−cot2θcot2θ−cot3θ. Solution in Punjabi
Step by step video & image solution for If a tower subtends angles theta, 2 theta and 3 theta at three points A,B,and C respectively, lying on the same side of a horizontal line through the foot of the tower, show that (AB)/(BC) (cot theta- cot 2 theta)/(cot 2 theta - cot 3 theta). by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - PROPERTIES AND SOLUTIONS OF TRIANGLES EXERSISE 1: SINGLE OPTION CORRECT TYPE QUESTIONS
1 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Single Option Correct Type Questions)
65 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 7
10 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
In the adjoining figure, the circle meets the sides of an equilateral triangle at six points.
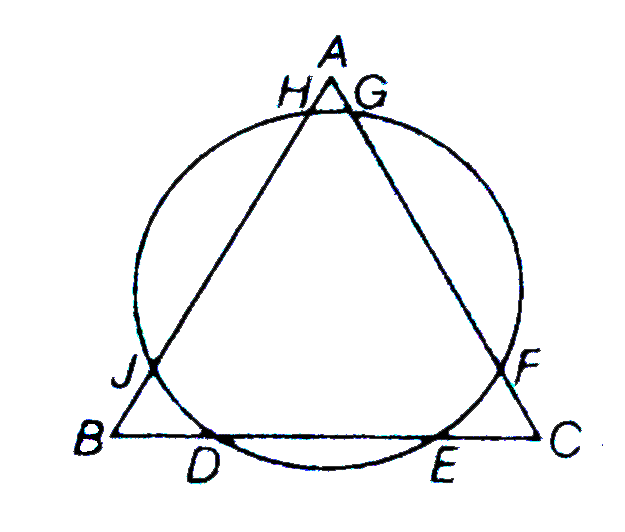
If AG=2,GF=13,FC=1andHJ=7, then DE equals to
View SolutionIn a ΔABC, if a=13,b=14andc=15, then ∠A is equal to (All symbols used have their usual meaning in a triangle.)
View SolutionIn triangle ABC,a:b:c=(1+x):1:(1−x) where x∈(0,1) If ∠A=π2+∠C, then x equal to
View SolutionLet O be the origin and let PQR be an arbitrary triangle. The point S is such that ¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OQ+¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OR+¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OS Then the triangle PQR has S as its
View SolutionLet a,b,c be in A.P. and |a|<1,|b|<1|c|<1.ifx=1+a+a2+....to ∞,y=1+b+b2+....to ∞and,z=1+c+c2+...to ∞, then x,y,z are in
View SolutionProve that: cotθcot2θ+cot2θcot3θ+2=cotθ(cotθ−cot3θ)
View Solutionprove that cotθ.cot2θ+cot2θ.cot3θ+2=cotθ(cotθ−cot3θ)
View SolutionIf cot^(2)theta=cot(theta-alpha)cot(theta-beta), then show that cot2 theta=(1)/(2)[cot alpha+cot beta]
View SolutionShow that :3(tan2θ+cot2θ)−9(cotθ+tanθ)+10>0
View Solutionसिद्ध कीजिए कि : cosec θ+cotθcosec θ−cotθ=(cosecθ+cotθ)2
View SolutionProve that:cotθcot2θ+cot2θcot3θ+2=cotθ(cotθ−cot3θ)
View SolutionA tower subtends angle theta, 2theta, 3theta at the three points A,B and C respectively lying on a horizontal line through the foot of the tower. Then AB/BC is equal to
View Solutioncosec2θ/cotθ−cotθ=
View SolutionProve that: cosecθ+cotθcosecθ−cotθ=(cosecθ+cotθ)2=1+2cot2θ+2cosecθcotθ.
View SolutionShow that cotθ⋅cot2θ+cot2θ⋅cot3θ+2=cotθ(cotθ−cot3θ)
View SolutionIf A+B+C=π then show that cotθ=cotA+cotB+cotC⇔sin(A−θ)⋅sin(B−θ)⋅sin(C−θ)=sin3θ.
View Solution
