The angle of elevation of the top of the tower observed from each of three points A,B , C on the ground, forming a triangle is observed to be ′ θ ′ . If R is the circum-radius of the triangle ABC, then find the height of the tower Solution in Punjabi
Step by step video & image solution for The angle of elevation of the top of the tower observed from each of three points A,B , C on the ground, forming a triangle is observed to be ′ θ ′ . If R is the circum-radius of the triangle ABC, then find the height of the tower by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - PROPERTIES AND SOLUTIONS OF TRIANGLES EXERSISE 1: SINGLE OPTION CORRECT TYPE QUESTIONS
1 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Single Option Correct Type Questions)
65 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 7
10 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
In the adjoining figure, the circle meets the sides of an equilateral triangle at six points.
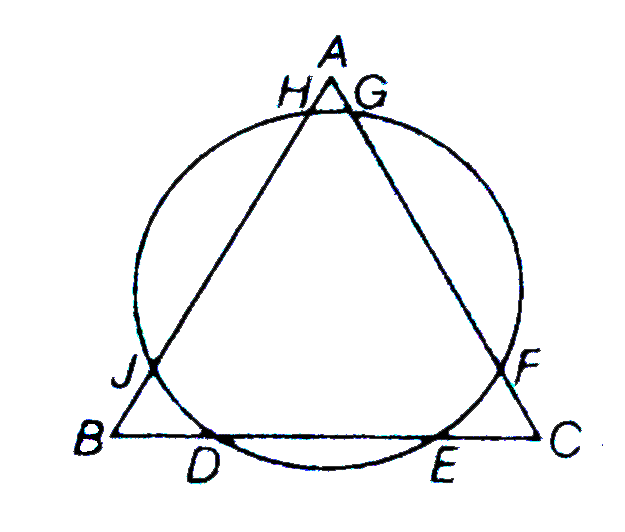
If AG=2,GF=13,FC=1andHJ=7, then DE equals to
View SolutionIn a ΔABC, if a=13,b=14andc=15, then ∠A is equal to (All symbols used have their usual meaning in a triangle.)
View SolutionIn triangle ABC,a:b:c=(1+x):1:(1−x) where x∈(0,1) If ∠A=π2+∠C, then x equal to
View SolutionLet O be the origin and let PQR be an arbitrary triangle. The point S is such that ¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OQ+¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OR+¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OS Then the triangle PQR has S as its
View SolutionLet a,b,c be in A.P. and |a|<1,|b|<1|c|<1.ifx=1+a+a2+....to ∞,y=1+b+b2+....to ∞and,z=1+c+c2+...to ∞, then x,y,z are in
View SolutionFrom a point on the ground,the angle of elevation of the top of the tower is observed to be 60^(@). Form a point 40m vertically above the first point of observation,the angle of elevation of the top of the tower is 30^(@). Find the height of the tower and its horizontal distance from the point of observation
View SolutionFrom a point on the ground which is at a distance of 50 m from the foot of the towe, the angle of elevation of the top of the tower is observed to be 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of the tower observed from each of three points A,B , C on the ground, forming a triangle is the same angle α. If R is the circum-radius of the triangle ABC, then find the height of the tower
View SolutionThe angle of elevation of the top of the tower observed from each of the three points A,B,C on the ground, forming a triangle is the same anlge alpha . IF R is the circumradius of the Delta ABC , then the height of the tower is
View SolutionA pole 5 m high is fixed on the top of a tower. The angle of elevation of the top of the pole as observed from a point A on the ground is 60∘ and the angle of depression of the point A from the top of the tower is 45∘. Find the height of the tower.
View Solutionधरातल पर त्रिभुज बनाने वाले तीन बिंदुओं A,B,C में से प्रत्येक का किसी टॉवर के शिखर पर उन्नयन कोण α है। यदि R,ΔABC की परित्रिज्या हो, तो टॉवर की ऊंचाई है
View Solutionएक मीनार के सिरे का भुमि पर त्रिभुज बनाने वाले तीन बिन्दुओं A,B,C में से प्रत्येक से उन्यन कोण समान तथा α के बराबर है यदि त्रिभुज ABC के परित्रिज्या R हैं तब मीनार की ऊँचाई है
View SolutionA pole 5 m high is fixed on the top of a tower. The angle of elevation of the top of the pole observed from a point 'A' on the ground is 60∘ and the angle of depression of the point ‘A’ from the top of the tower is 45∘. Find the height of the tower
View SolutionThe angle of elevation of the top of the tower observed from each of three points A,B , C on the ground, forming a triangle is the same angle α. If R is the circum-radius of the triangle ABC, then find the height of the tower
View SolutionThe angle of elevation of the top of the tower observed from each of the three points A,B,C on the ground, forming a triangle is the same angle ∝. If R is the circum-radius of the triangle ABC, then the height of the tower is
View Solution
