[Punjabi] A train travelling on one of two intersecting railway lines,

In the adjoining figure, the circle meets the sides of an equilateral triangle at six points.
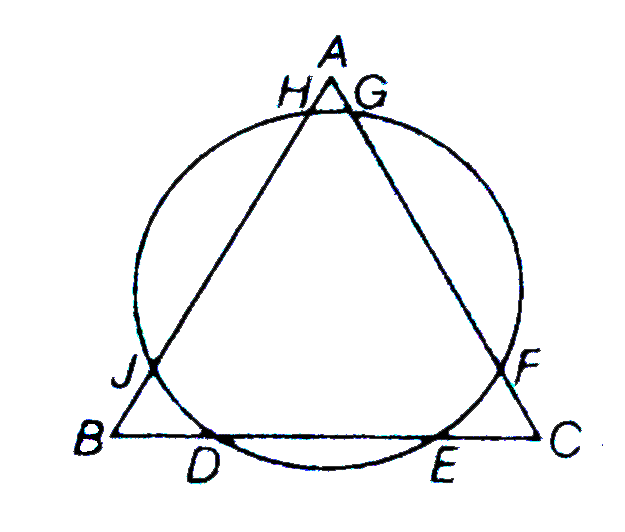
ocr_image
If $A G = 2, G F = 13, F C = 1 and H J = 7,$ then DE equals to

View Solution

In a $Δ A B C,$ if $a = 13, b = 14 and c = 15,$ then $∠ A$ is equal to (All symbols used have their usual meaning in a triangle.)

View Solution

In triangle $A B C, a : b : c = (1 + x) : 1 : (1 - x)$ where $x \in (0, 1)$ If $∠ A = \frac{π}{2} + ∠ C$ , then $x$ equal to

View Solution

Let O be the origin and let PQR be an arbitrary triangle. The point S is such that $¯ ¯¯¯¯ ¯ O P \cdot ¯ ¯¯¯¯ ¯ O Q + ¯ ¯¯¯¯ ¯ O R \cdot ¯ ¯¯¯¯ ¯ O S = ¯ ¯¯¯¯ ¯ O R \cdot ¯ ¯¯¯¯ ¯ O P + ¯ ¯¯¯¯ ¯ O Q \cdot ¯ ¯¯¯¯ ¯ O S = ¯ ¯¯¯¯ ¯ O Q \cdot ¯ ¯¯¯¯ ¯ O R + ¯ ¯¯¯¯ ¯ O P \cdot ¯ ¯¯¯¯ ¯ O S$ Then the triangle PQR has S as its

View Solution

Let a,b,c be in A.P. and $| a | < 1, | b | < 1 | c | < 1 . if x = 1 + a + a^{2} + . . . . to \infty, y = 1 + b + b^{2} + . . . . to \infty and, z = 1 + c + c^{2} + . . . to \infty$ , then x,y,z are in

View Solution

If alpha and beta are acute angles and cot alpha=(1)/(4) and cot beta=(5)/(3)* prove that alpha-beta=45

View Solution

Suppose $α, β a n d t h η$ are angles satisfying $0 < α < θ < β < \frac{π}{2} .$ Then prove that $\frac{sin α - sin β}{cos β - cos α} = - cot θ$

View Solution

If alpha and beta be the angles subtended by the major axis to an ellipse at the extremities of a pair of conjugate diameters then cot^(2)alpha+cot^(2)beta is equal to

View Solution

If cot^(2)theta=cot(theta-alpha)cot(theta-beta), then show that cot2 theta=(1)/(2)[cot alpha+cot beta]

View Solution

From the bottom of a pole of height h the angle of elevation of the top of a tower is alpha and the pole subtends an angle beta at the top of the tower . Prove that the height of the tower is (h cot(alpha - beta))/(cot(alpha - beta) - cot alpha) .

View Solution

$If \frac{sin (α - β)}{sin β} = \frac{sin (α + θ)}{sin θ}, < b r > prove that cot β - cot θ = cot (α + θ) + cot (α - β)$

View Solution

If ${cot}^{2} θ = cot (θ - α) cot (θ - β)$ , prove that $cot α + cot β = 2 cot 2 θ$

View Solution

A train travelling on one of two intersecting railway lines, subtends at a certain station on the other line, an angle $α$ when the front of the carriage reaches the junction, and an angle $β$ when the end of the carriage reaches it. Prove that the two lines are inclined to each other at an angle $θ$ determined by $2 cot θ = cot α - cot β$ .

View Solution

${cot}^{2} θ = cot (θ - α) cot (θ - β)$ হলে দেখাও যে, $cot α + cot β = 2 cot 2 θ$

View Solution

A plumb line gets inclined to vertical at angle $θ$ when the carriage travels with an acceleration a. The plumb line is suspended from the roof of the carriage. The acceleration a is given by
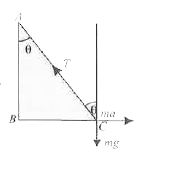
ocr_image

View Solution

যদি $α$ ও $β$ কোণ দুটি পরস্পর পূরক কোণ হয়, তাহলে দেখাও যে, $cot β + cos β = \frac{cos β}{cos α} (1 + sin β)$

View Solution

Topper's Solved these Questions

Similar Questions

ARIHANT MATHS-PROPERTIES AND SOLUTION OF TRIANGLES -Exercise For Sesssion 8