A plank is resting on a horizontal ground in the northern hemisphere of the Earth at a latitude. Let the angular speed of the Earth be and its radius . The magnitude of the frictional force on the plank will be
Zero
The correct Answer is:C
To find the magnitude of the frictional force on the plank resting on a horizontal ground at a latitude of 45∘, we can follow these steps:
1. Identify Forces Acting on the Plank:
- The weight of the plank mg acts downward.
- The normal force N acts upward.
- The centrifugal force due to Earth's rotation acts outward, which can be expressed as Fc=mω2r, where r is the radius of the circular path of the plank.
2. Determine the Radius r:
- At a latitude of 45∘, the radius r can be related to the Earth's radius Re using trigonometric relationships. The radius r is given by:
r=Recos(45∘)=Re√2
3. Calculate the Centrifugal Force:
- Substitute r into the expression for centrifugal force:
Fc=mω2r=mω2(Re√2)=mω2Re√2
4. Resolve Forces:
- The centrifugal force can be resolved into two components: one acting perpendicular to the normal force and one acting parallel (tangential) to the surface.
- The component acting parallel to the surface (which will be balanced by friction) is:
Ftangential=Fcsin(45∘)=mω2Re√2⋅1√2=mω2Re2
5. Frictional Force:
- The frictional force f must balance the tangential component of the centrifugal force:
f=mω2Re2
Final Answer:
The magnitude of the frictional force on the plank is:
f=mReω22
- The weight of the plank mg acts downward.
- The normal force N acts upward.
- The centrifugal force due to Earth's rotation acts outward, which can be expressed as Fc=mω2r, where r is the radius of the circular path of the plank.
- At a latitude of 45∘, the radius r can be related to the Earth's radius Re using trigonometric relationships. The radius r is given by:
r=Recos(45∘)=Re√2
- Substitute r into the expression for centrifugal force:
Fc=mω2r=mω2(Re√2)=mω2Re√2
- The centrifugal force can be resolved into two components: one acting perpendicular to the normal force and one acting parallel (tangential) to the surface.
- The component acting parallel to the surface (which will be balanced by friction) is:
Ftangential=Fcsin(45∘)=mω2Re√2⋅1√2=mω2Re2
- The frictional force f must balance the tangential component of the centrifugal force:
f=mω2Re2
The magnitude of the frictional force on the plank is:
f=mReω22
Topper's Solved these Questions
- BOOK - KVPY PREVIOUS YEARCHAPTER - QUESTION PAPER 2013EXERCISE - PART-II ( PHYSICS)10videos
QUESTION PAPER 2013
- BOOK - KVPY PREVIOUS YEARCHAPTER - QUESTION PAPER 2013EXERCISE - PART-II (PHYSICS)5videos
QUESTION PAPER 2013
- BOOK - KVPY PREVIOUS YEARCHAPTER - MOCK TEST 9EXERCISE - EXERCISE19videos
MOCK TEST 9
- BOOK - KVPY PREVIOUS YEARCHAPTER - QUESTION PAPER 2020EXERCISE - PART-I : PHYSICS50videos
QUESTION PAPER 2020
Similar Questions
In the figure shown, a solid sphere of mass 'm' and radius r is released from a height 6r to slide down a smooth surface. A plank of same mass 'm' touches the horizontal portion of the surface at the ground. The co-efficient of friction between the plank and the sphere is μ and that between the plank and the ground is μ/4. Find the work done by the friction force between the plank and the ground till the sphere starts pure rolling on the plank. Neglect the height of the plank.
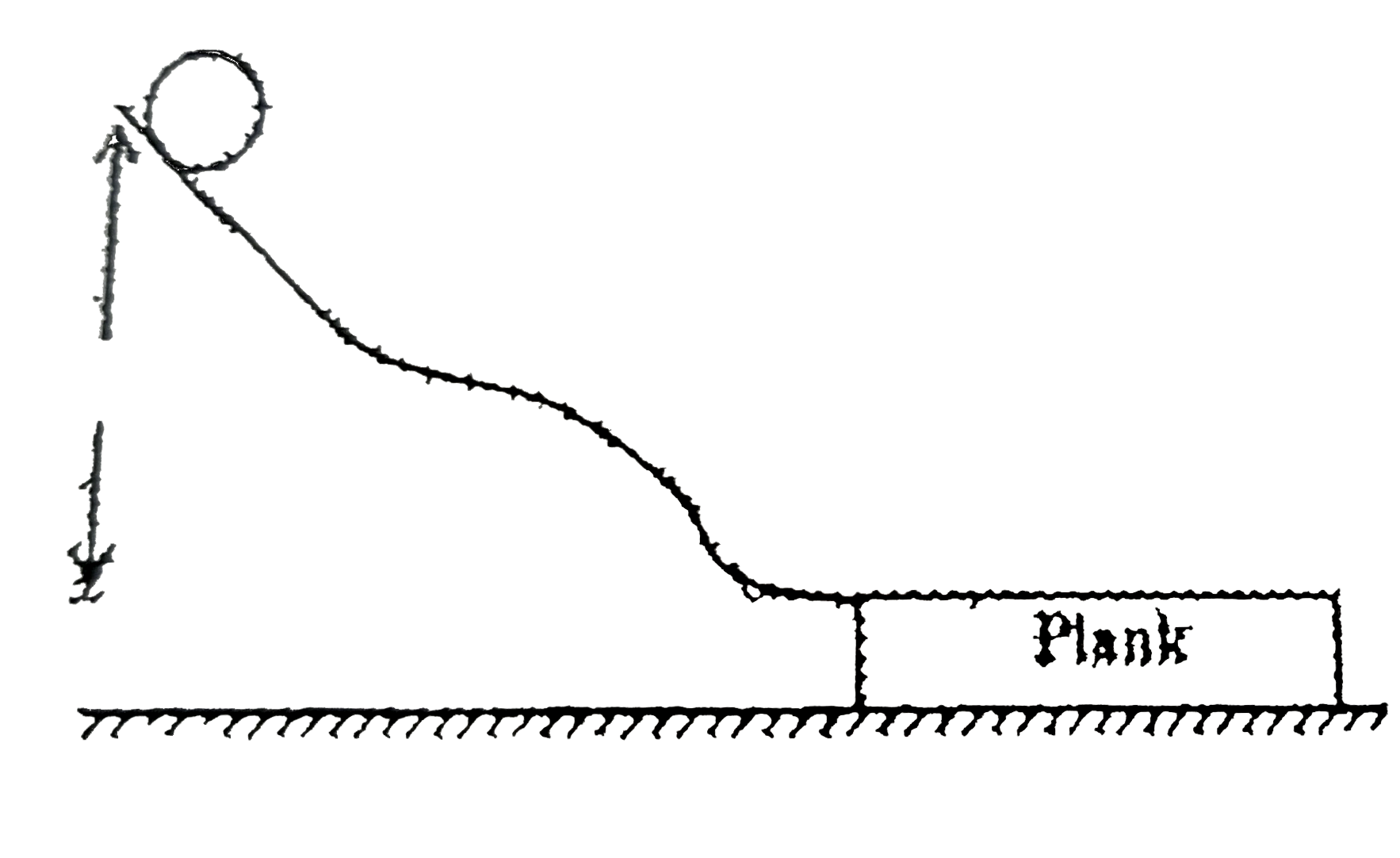 View Solution
View Solution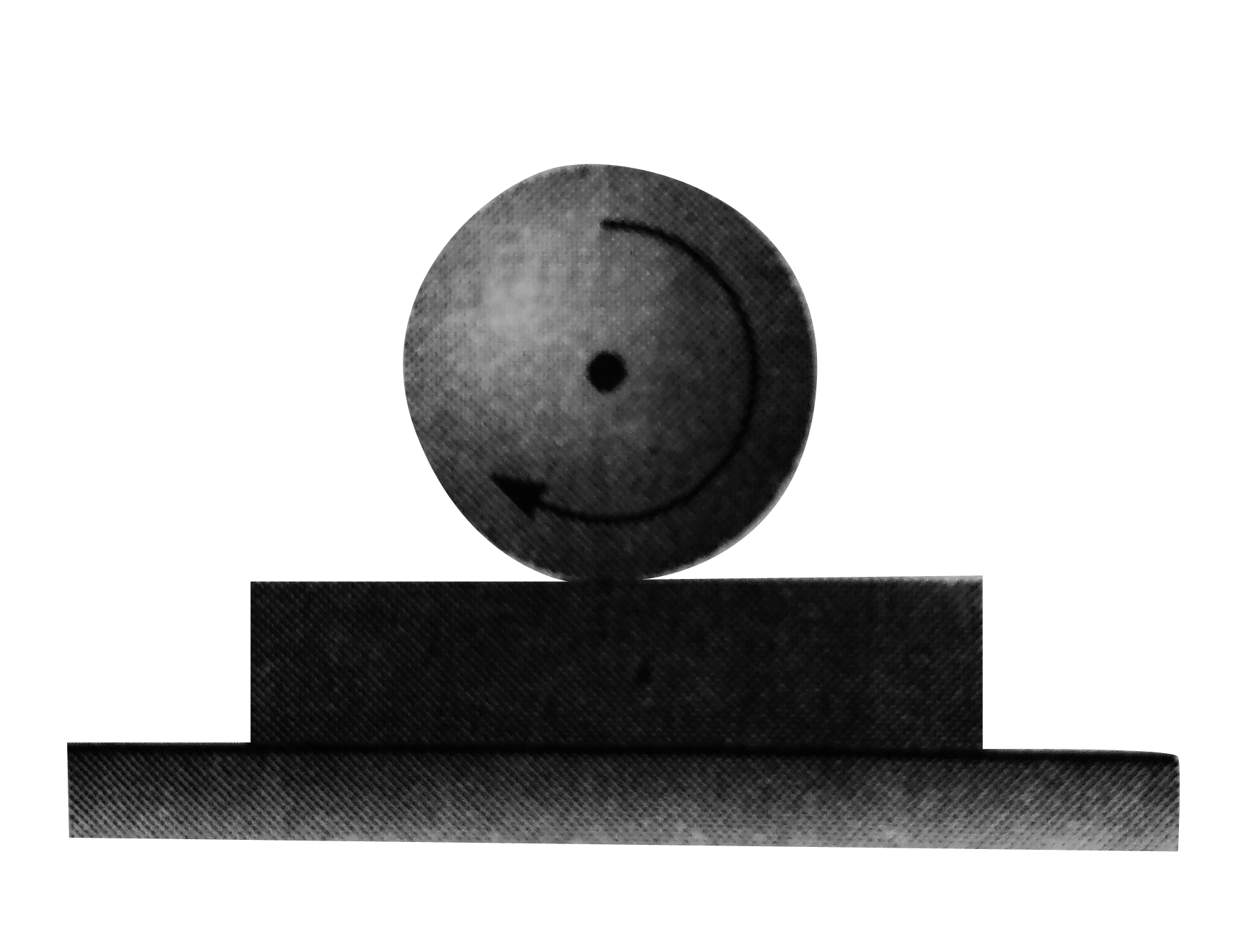
A horizontal plank having mass m lies on a smooth horizontal surface. A sphere of same mass and radius r is spined to angular frequency ω0 and gently placed on the plank as shown in the figure. If coefficient of friction between the plank and the sphere is μ. Find the distance moved by the plank till sphere starts pure rolling on the plank. the plank is long enough.View Solution
Knowledge Check
- Question 1 - Select One
A uniform thin rod of mass m and length R is placed normally on surface of earth as shown. The mass of earth is M and its radius R. Then the magnitude of gravitational force exerted by earth on the rod is
 AGMm2R2BGMm4R2C4GMm9R2DGMm8R2
AGMm2R2BGMm4R2C4GMm9R2DGMm8R2 - Question 2 - Select One
A train of mass m moves with a velocity υ on the equator from east to west. If ω is the angular speed of earth about its axis and R is the radius of the earth then the normal reaction acting on the train is
Amg[1−(ωR−2υ)ωg−υ2Rg]Bmg[1−2(ωR−υ)ωg−υ2Rg]Cmg[1−(ωR−2υ)ωg−υ2Rg]Dmg[1−2(ωR−υ)ωg−υ2Rg] - Question 3 - Select One
If ωE and ωH are the angular velocities of the earth rotating about its own axis and the hour hand of the clock respectively, then
AωE=15ωHBωE=2ωHCωE=ωHDωE=12ωH
A plank of mass M rests on a smooth horizontal plane. A sphere of mass m and radius r is placed on the rough upper surface of the plank and the plank is suddenly given a velocity v in the direction of its length. Find the time after which the sphere begins pure rolling, if the coefficient of friction between the plank and the sphere is μ and the plank is sufficiently long.
View SolutionIf earth suddenly stop rotating , then the weight of an object of mass m at equator will [ ω is angular speed of earth and R is its radius . ]
View SolutionA long horizontal plank of mass m is lying on a smooth horizontal surface. A sphere of same mass m and radius r is spinned about its own axis with angular velocity we and gently placed on the plank. The coefficient of friction between the plank and the sphere is μ. After some time the pure rolling of the sphere on the plank will start. Answer the following questions.

Find the displacement of the plank till the sphere starts pure rolling.View SolutionA long horizontal plank of mass m is lying on a smooth horizontal surface. A sphere of same mass m and radius r is spinned about its own axis with angular velocity we and gently placed on the plank. The coefficient of friction between the plank and the sphere is μ. After some time the pure rolling of the sphere on the plank will start. Answer the following questions.
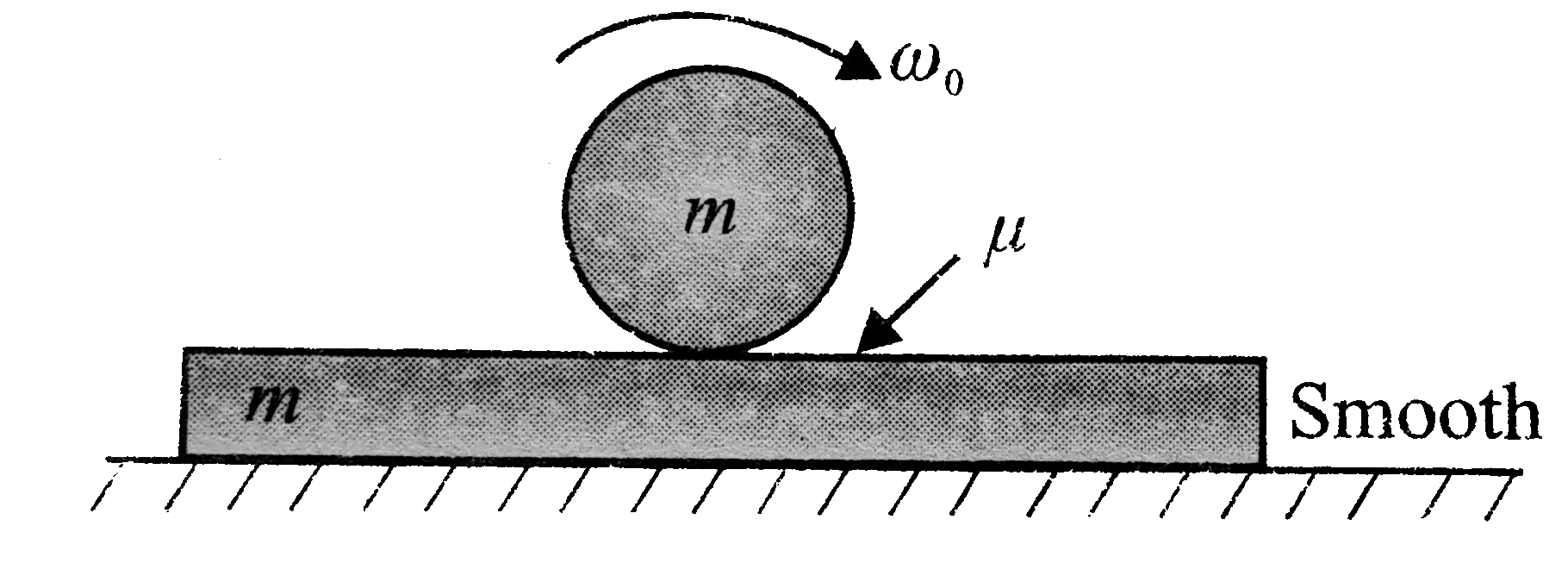
Find the velocity of the sphere after the pure rolling startsView SolutionA long horizontal plank of mass m is lying on a smooth horizontal surface. A sphere of same mass m and radius r is spinned about its own axis with angular velocity we and gently placed on the plank. The coefficient of friction between the plank and the sphere is μ. After some time the pure rolling of the sphere on the plank will start. Answer the following questions.
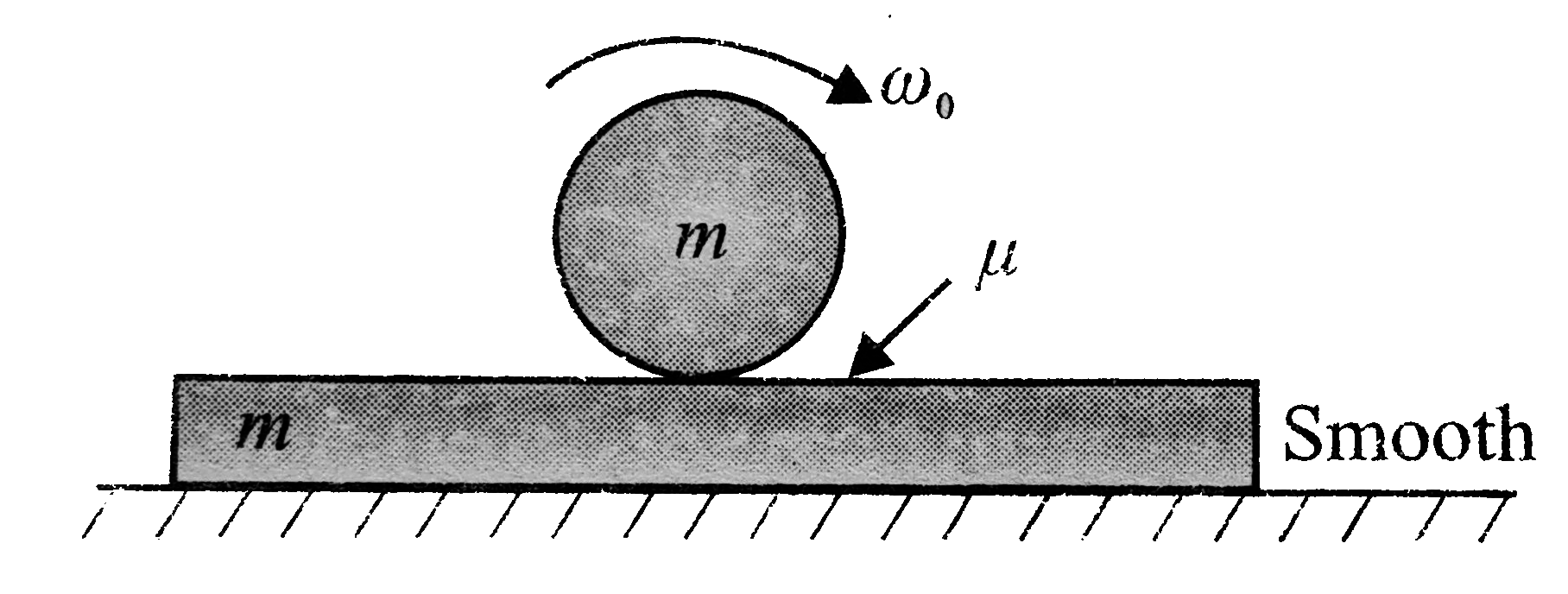
Find the time t at which the pure roiling startsView Solution

