777777 .......... 129 times is divided by 37, the remainder obtained is ?
0
37
1
36
The correct Answer is:b
To solve the problem of finding the remainder when the number formed by 777... (129 times) is divided by 37, we can follow these steps:
Step 1: Understand the Number
The number we are dealing with is 777... (129 times). This can be expressed as:
N=777...777 (129 times)
Step 2: Express the Number Mathematically
We can express this number as:
N=7×10128+7×10127+7×10126+…+7×100
This is a geometric series where the first term a=7 and the common ratio r=10.
Step 3: Sum the Geometric Series
The sum S of the first n terms of a geometric series can be calculated using the formula:
S=a(rn−1)(r−1)
In our case, n=129, a=7, and r=10:
S=7(10129−1)(10−1)
S=7(10129−1)9
Step 4: Find the Remainder when Divided by 37
Now we need to find Smod37:
N=7(10129−1)9
To find Nmod37, we first need to calculate 10129mod37.
Step 5: Calculate 10129mod37
Using Fermat's Little Theorem, since 37 is prime:
1036≡1mod37
Now, we can reduce 129mod36:
129÷36=3 remainder 21
So,
129mod36=21
Thus,
10129≡1021mod37
Step 6: Calculate 1021mod37
We can calculate 1021mod37 by successive squaring:
- 101≡10
- 102≡100mod37≡26
- 104≡262mod37≡36≡−1
- 108≡(−1)2≡1
- 1016≡12≡1
Now, we can combine these:
1021=1016×104×101
1021≡1×(−1)×10≡−10mod37≡27
Step 7: Substitute Back to Find Nmod37
Now we substitute back:
N≡7(27−1)9mod37
N≡7×269mod37
Calculating 7×26=182:
Now we need to find 1829mod37.
Step 8: Find the Modular Inverse of 9 mod 37
We need to find the modular inverse of 9 modulo 37. Using the Extended Euclidean Algorithm, we find that the inverse is 33 (since 9×33≡1mod37).
Step 9: Calculate the Final Remainder
Now we can calculate:
N≡182×33mod37
Calculating 182mod37:
182÷37=4 remainder 34
So,
N≡34×33mod37
Calculating 34×33=1122:
Now find 1122mod37:
1122÷37=30 remainder 12
Thus, the remainder when 777...777 (129 times) is divided by 37 is:
12
The number we are dealing with is 777... (129 times). This can be expressed as:
N=777...777 (129 times)
We can express this number as:
N=7×10128+7×10127+7×10126+…+7×100
The sum S of the first n terms of a geometric series can be calculated using the formula:
S=a(rn−1)(r−1)
S=7(10129−1)(10−1)
S=7(10129−1)9
Now we need to find Smod37:
N=7(10129−1)9
Using Fermat's Little Theorem, since 37 is prime:
1036≡1mod37
129÷36=3 remainder 21
So,
129mod36=21
Thus,
10129≡1021mod37
We can calculate 1021mod37 by successive squaring:
- 101≡10
- 102≡100mod37≡26
- 104≡262mod37≡36≡−1
- 108≡(−1)2≡1
- 1016≡12≡1
1021=1016×104×101
1021≡1×(−1)×10≡−10mod37≡27
Now we substitute back:
N≡7(27−1)9mod37
N≡7×269mod37
Now we need to find 1829mod37.
We need to find the modular inverse of 9 modulo 37. Using the Extended Euclidean Algorithm, we find that the inverse is 33 (since 9×33≡1mod37).
Now we can calculate:
N≡182×33mod37
Calculating 182mod37:
182÷37=4 remainder 34
So,
N≡34×33mod37
Calculating 34×33=1122:
Now find 1122mod37:
1122÷37=30 remainder 12
12
Topper's Solved these Questions
Similar Questions
Knowledge Check
When
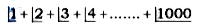 is divided by 12, the remainder obtained is? A6B5C9D7
is divided by 12, the remainder obtained is? A6B5C9D7When 777777 ............ 363 times, is divided by 11, the remainder obtained is?
A10B7C3D8When
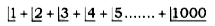 is divided by 10, the remaindeer obtained is ? A3B1C2D9
is divided by 10, the remaindeer obtained is ? A3B1C2D9
When 666666 ........... 134 times, is divided by 13, the remainder obtained is?
View SolutionWhen 1177 is divided by 7, the remainder obtained is ?
View SolutionWhen 54124 is divided by 17, the remainder obtained is ?
View SolutionWhen 4177 is divided by 17, the remainder obtained is ?
View SolutionWhen 242 is divided by 33, the remainder obtained is ?
View Solution

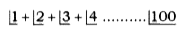 , is divided by 5, the remainder obtained is ?
, is divided by 5, the remainder obtained is ?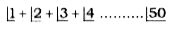 , is divided by 12, the remainder obtained is ?
, is divided by 12, the remainder obtained is ?