If G is centre of $Δ A B C$ , AG = BC, Find $∠ B F C$ .

$45^{\circ}$

$90^{\circ}$

$63^{\circ}$

$75^{\circ}$

Video Solution

Text Solution

Generated By DoubtnutGPT

To solve the problem, we need to find the angle $∠ B F C$ given that $G$ is the centroid of triangle $A B C$ and $A G = B C$ .
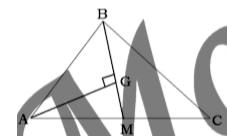
1. Understand the Properties of the Centroid:
- The centroid $G$ of triangle $A B C$ divides each median into a ratio of 2:1. This means that $A G$ is two-thirds the length of the median from $A$ to side $B C$ .
2. Draw the Triangle:
- Draw triangle $A B C$ and mark the centroid $G$ . Connect points $A$ , $B$ , and $C$ to form the triangle.
3. Label the Points:
- Let $D$ be the midpoint of side $B C$ . Since $G$ is the centroid, it lies on the median $A D$ .
4. Use the Given Condition:
- We are given that $A G = B C$ . Since $G$ divides $A D$ in a 2:1 ratio, we can express $A D$ in terms of $A G$ :
$A G = \frac{2}{3} A D and A D = \frac{3}{2} A G$
5. Set Up the Angles:
- In triangle $A B C$ , the angles at $G$ can be expressed in terms of the angles at $A$ , $B$ , and $C$ :
$∠ G B C + ∠ B G C + ∠ G C B = 180^{\circ}$
6. Use the Angle Sum Property:
- Since $A G = B C$ , we can conclude that $∠ B G C$ is a right angle:
$∠ B G C = 90^{\circ}$
7. Calculate the Remaining Angles:
- Thus, we can simplify:
$∠ G B C + ∠ G C B = 90^{\circ}$
8. Conclusion:
- Therefore, we find that $∠ B F C$ is $90^{\circ}$ .
Final Answer:
$∠ B F C = 90^{\circ}$

Updated on:7/8/2024

In a $Δ A B C, G$ is centroid , $A G = B C$ . Find $∠ B G C$ .
A(a) 100 degree
B(b) 90 degree
C(c) 80 degree
D(d) 120 degree
G is the centroid of $Δ A B C$ . If $A G = B C$ , then measure of $∠ B G C$ is
A $45^{\circ}$
B $60^{\circ}$
C $90^{\circ}$
D $120^{\circ}$
G is the centroid of $Δ A B C$ . If $A G = B C$ , then measure of $∠ B G C$ is
A $¯ ¯¯¯¯ ¯ G P$
B $2 ¯ ¯¯¯¯ ¯ G P$
C $3 ¯ ¯¯¯¯ ¯ G P$
D $2 ¯ ¯¯¯¯ ¯ G A$