If O is a circumcentre of ΔABC and OD⊥BC the find ∠BOD?
∠A
12∠A
12∠B
12∠C
To solve the problem, we need to find the angle ∠BOD given that O is the circumcenter of triangle ABC and OD is perpendicular to BC.
1. Draw the Circumcircle and Triangle:
- Begin by drawing the circumcircle of triangle ABC with circumcenter O. Label the triangle vertices as A, B, and C.
2. Identify the Perpendicular:
- Draw the line segment OD such that it is perpendicular to BC. This means that ∠ODB=90∘ and ∠ODC=90∘.
3. Use the Property of the Perpendicular from the Circumcenter:
- Recall that if a perpendicular is drawn from the circumcenter O to a chord BC, it bisects the chord. Therefore, BD=DC.
4. Establish Congruent Triangles:
- Since OD is perpendicular to BC and BD=DC, we can conclude that triangles ODB and ODC are congruent by the RHS (Right angle-Hypotenuse-Side) congruence criterion.
- This gives us:
- OB=OC (radii of the circumcircle)
- OD is common to both triangles
- BD=DC
5. Determine Angles in the Congruent Triangles:
- From the congruence of triangles ODB and ODC, we have:
- ∠ODB=∠ODC=90∘
- ∠BOD=∠COD
6. Relate Angles BOC and A:
- The angle ∠BOC is an inscribed angle that subtends arc BC. By the inscribed angle theorem, ∠BOC=2×∠A (where ∠A is the angle at vertex A of triangle ABC).
7. Find Angle BOD:
- Since ∠BOD+∠COD=∠BOC, and we know that ∠BOD=∠COD (from the congruence of triangles), we can write:
- 2×∠BOD=2×∠A
- Thus, ∠BOD=∠A.
Final Answer:
Therefore, the angle ∠BOD is equal to ∠A.
- Begin by drawing the circumcircle of triangle ABC with circumcenter O. Label the triangle vertices as A, B, and C.
- Draw the line segment OD such that it is perpendicular to BC. This means that ∠ODB=90∘ and ∠ODC=90∘.
- Recall that if a perpendicular is drawn from the circumcenter O to a chord BC, it bisects the chord. Therefore, BD=DC.
- Since OD is perpendicular to BC and BD=DC, we can conclude that triangles ODB and ODC are congruent by the RHS (Right angle-Hypotenuse-Side) congruence criterion.
- This gives us:
- OB=OC (radii of the circumcircle)
- OD is common to both triangles
- BD=DC
- From the congruence of triangles ODB and ODC, we have:
- ∠ODB=∠ODC=90∘
- ∠BOD=∠COD
- The angle ∠BOC is an inscribed angle that subtends arc BC. By the inscribed angle theorem, ∠BOC=2×∠A (where ∠A is the angle at vertex A of triangle ABC).
- Since ∠BOD+∠COD=∠BOC, and we know that ∠BOD=∠COD (from the congruence of triangles), we can write:
- 2×∠BOD=2×∠A
- Thus, ∠BOD=∠A.
Therefore, the angle ∠BOD is equal to ∠A.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If O is the circumcentre of ΔABC and OD ⊥ BC, then ∠BOD must be equal to
A∠AB12∠AC12∠BD12∠CIf S is the circumcentre of ΔABC and ∠A=50∘, then the value of ∠BCS is
A20∘B40∘C60∘D80∘In a ΔABC,AB=ACandAD⊥BC, then:
AAB<ADBAB>ADCAB=ADDAB≤AD
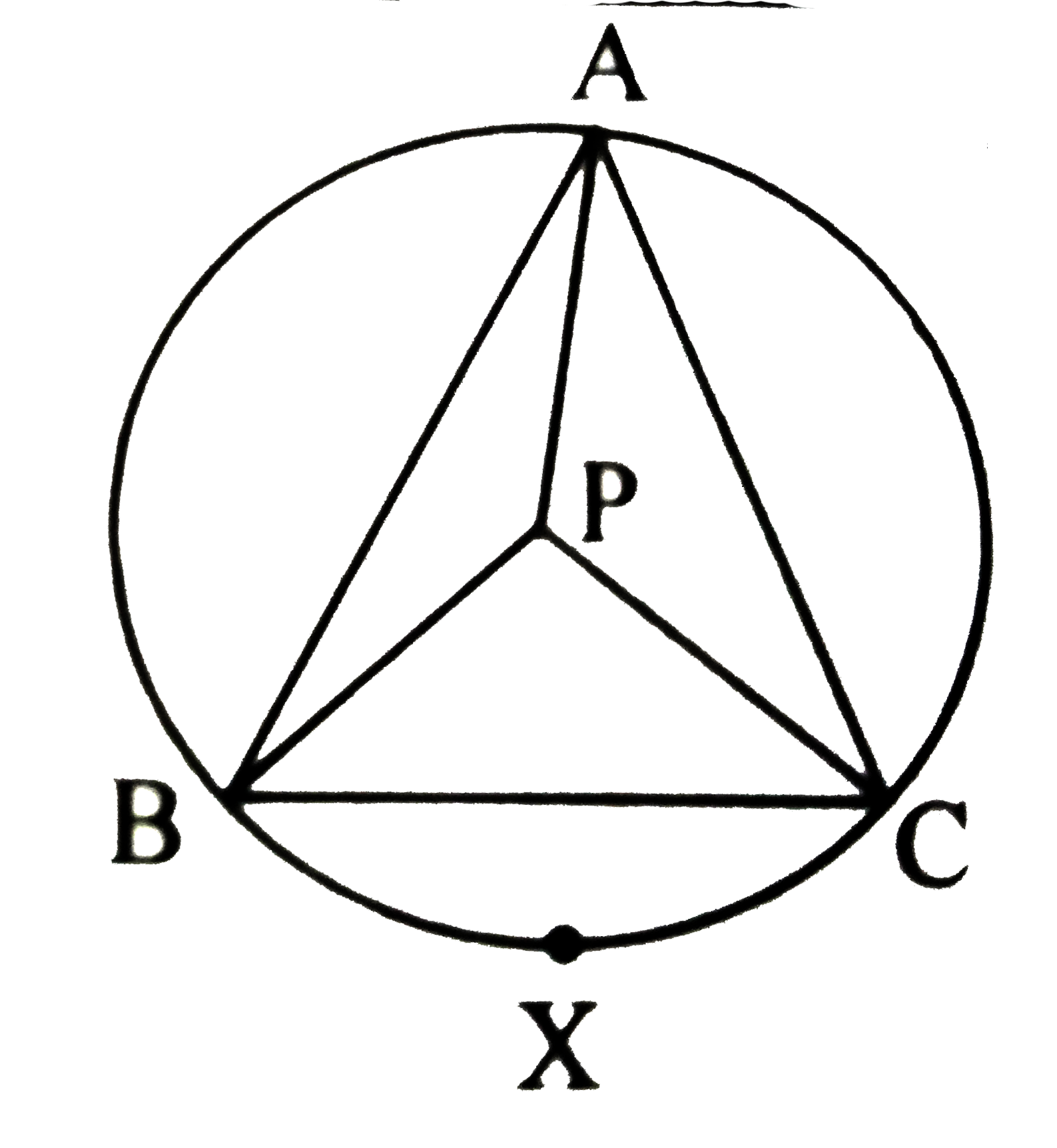
In the adjoining figure, P is the circumcentre of the ΔABC.m∠APC=118∘ and m∠PBC=45∘ , then find:
(i) m(arc BXC)
(ii) m(arc BCA)
View SolutionO is circumcentre of ΔABC if ∠BAC=85∘∠BCA=80∘ find ∠OAC.
View SolutionThe diagonal of the square field measures 50m. The area of square field is?
View SolutionO is the circumcentre of ΔABC, given ∠BAC=85∘and∠BCA=55∘, find ∠OAC.
View SolutionIn a ΔABC,∠A=∠B+∠C. O and S are the orthocentre and the circumcentre of ΔABC. If AB=12 cm and AC=5 cm, then find the distance between O and S.
View Solution
